3
$\begingroup$

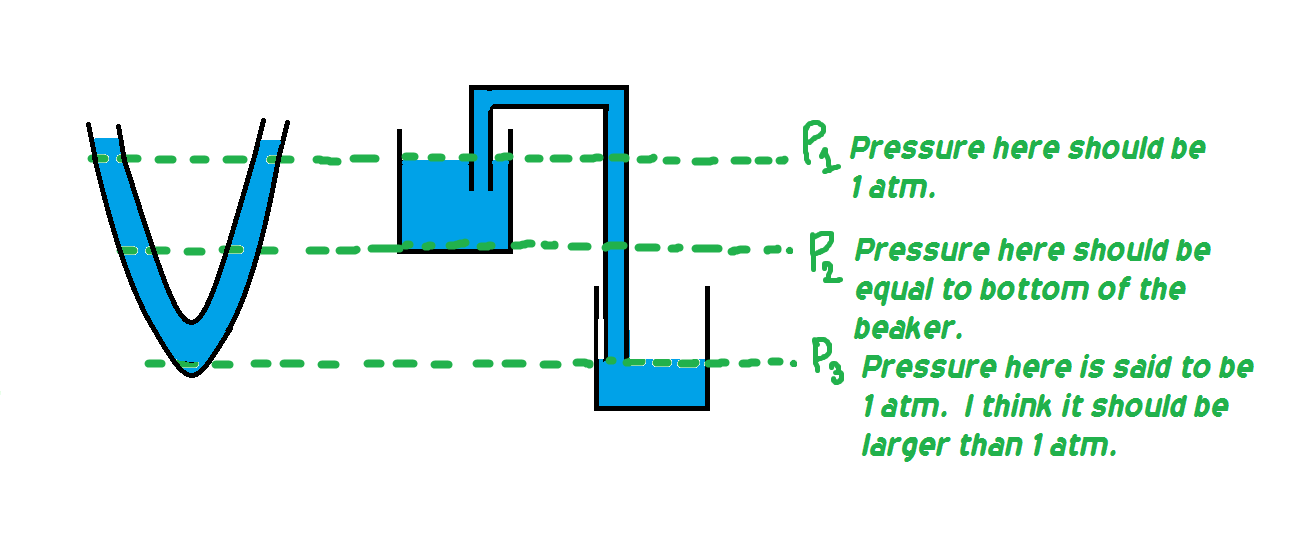
I assume the area of horizontal cross section of beaker at the top to be way larger than the area of horizontal cross section of pipe

I assume the area of horizontal cross section of beaker at the top to be way larger than the area of horizontal cross section of pipe

I think at the same depth pressure of a specific liquid which are connected to each other, as in manometer, should be same. But, in siphon that does not seem to be the case, which is the reason for my question.

Why at the bottom of the pipe of siphon pressure is 1 atm? I know that it is said that pressure is provided by atmosphere. But, why do not the pressure increase because depth increases?

Improve this question
$\endgroup$
9
  • 3
    $\begingroup$ The system on the right is not in equilibrium. If the blue stuff is a fluid, then it must be flowing at the moment pictured. If you prevent the fluid from flowing by stopping the lower end of the pipe, then the pressure inside the pipe at level P3 would be greater than atmospheric, while the pressure outside would be equal to atmospheric. $\endgroup$
    – Solomon Slow
    Commented Jul 27, 2018 at 14:42
  • $\begingroup$ But, is not the pressure at p3 said to be 1 atm? $\endgroup$
    – Ahmad
    Commented Jul 27, 2018 at 14:50
  • $\begingroup$ The pressure at any point inside a pipe is not necessarily the same as the pressure at a nearby point outside the pipe. $\endgroup$
    – Solomon Slow
    Commented Jul 27, 2018 at 14:56
  • $\begingroup$ Okay. Are you saying pressure inside the pipe works like manometer but pressure in the bottom beaker works like the top beaker? $\endgroup$
    – Ahmad
    Commented Jul 27, 2018 at 15:09
  • $\begingroup$ The pressure P3 is atmospheric. What does that tell you about the pressure at the very top of the siphon loop? $\endgroup$
    – Chet Miller
    Commented Jul 27, 2018 at 15:27

5 Answers 5

Reset to default
1
$\begingroup$

In this system, it is necessary to include the viscous frictional resistance of the fluid in the analysis, and not merely treat the fluid as being inviscid. All liquids exhibit viscous frictional behavior and, in the present case, it is dominant. If we neglect the kinetic energy increases and decreases of the fluid in the entrance and exit region of the siphon in the upper and lower tanks, then we have: $$\rho g h = \frac{1}{2}\rho v^2 f \left[\frac{4L}{D}\right]$$where L is the total length of the siphon tubing, D is the siphon tube diameter, h is the height difference between levels 1 and 3, and f is the Fanning friction factor (a constant for very rough pipe and a function of the Reynolds number for smooth pipe). So the pressure at point 1 in the down-leg of the siphon is given by: $$P_{1, down-leg}=P_{atm}-\rho g h+\frac{1}{2}\rho v^2 f \left[\frac{4h}{D}\right]=P_{atm}-\frac{1}{2}\rho v^2 f \left[\frac{4(L-h)}{D}\right]$$So the pressure at level 1 in the down leg is lower than atmospheric by the frictional pressure drop over the length of the loop of tubing between the fluid level in the upper tank and level 1 in the down-leg. This is consistent with the pressure being atmospheric at the fluid surface in the upper tank.

These results are all consistent with the fluid pressures being atmospheric as the surface of both tanks.

Improve this answer
$\endgroup$
0
$\begingroup$

The pressure at p3 and p1 have to be atmospheric because the fluid at those points are in direct contact with atmospheric pressure. When fluid is moving, you cannot just take the height into account. The system is governed by Bernoulli's equation.

$P+\rho\ g\ h +\frac{\rho\ v^2}{2}=\text{Constant}$

where

$P$ is the pressure, and $\rho$ is the fluid density. The velocity $v$ will depend on fluid viscosity, height of the siphon, pipe roughness, cross-sectional area of the pipe, etc.

The Bernoulli equation is just conservation of energy.

Improve this answer
$\endgroup$
0
$\begingroup$

Why at the bottom of the pipe of siphon pressure is 1 atm?

The pressure at the bottom of the right column is actually greater than $1\; \mathrm{atm}$, i.e., it is greater than the pressure at the surface of the bottom vessel, which is $1\; \mathrm{atm}$.

In other words, the pressure along the horizontal line $P_3$ is changing: from a higher pressure right under the column to a lower pressure at the surface. This is why the fluid is constantly moving. The pressure along this line would be constant, if the fluid was in balance or static, which, in an operating siphon, is not the case.

If we look at the whole picture, we could roughly say (without getting into details about various mechanisms involved in a siphon operation) that the fluid in the siphon moves due to the height difference between the left and the right columns, which introduces disbalance despite the equality of atmospheric pressure in both vessels.

Since, due to this disbalance, the fluid is moving, the distribution of pressure along its path cannot be determined based on the principles of hydrostatics.

Improve this answer
$\endgroup$
0
$\begingroup$

I think you are right because $P_1$ and $P_3$ are occurring at surface molecules and when all the water has been filled up by siphon, there is no point of any other force acting on it. Here $P_3>P_1$ as there are more air molecules in $P_3$ than on $p_1$

Improve this answer
$\endgroup$
0
$\begingroup$

As @Chet Miller shows, friction effects explain how the pressure at both free surfaces is the atmospheric pressure. One more point. If the elevation at the top of the siphon is too high, the pressure drops to the saturation pressure of the liquid at its temperature, the liquid flashes to vapor and the siphon ceases to function.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.