quantum superdense coding -How many qubits are needed?

Question

This is an extract from Wikipedia about superdense coding:

"In quantum information theory, superdense coding is a quantum communication protocol to transmit two classical bits of information (i.e., either 00, 01, 10 or 11) from a sender (often called Alice) to a receiver (often called Bob), by sending only one qubit from Alice to Bob, under the assumption of Alice and Bob pre-sharing an entangled state"

Question: I want to understand how this improves the computational efficiency in quantum computers - especially for many qubits.

In this simple example, Bob and Alice still need 2 qubits to be able to transmit two classical bits. One qubit is for transmission and one is needed as a reference in the entangled pair. This does not seem to improve the number of classical bits versus qubits needed - $2$ qubits needed to transmit $2 [=2^{2-1}]$ classical bits. However, in quantum computing, there will be , for example $30$ qubits in an entangled state. Is it fair to generalize ad state that we will still need a qubit as a reference and that we can transmit $2^{30-1}$ classical bits with $30$ entangled qubits? Or how should I see this?

Answer 1

I want to understand how this improves the computational efficiency in quantum computers - especially for many qubits

It doesn't. Superdense coding is a quantum communication protocol. As such, it is meant to provide advantages in the context of transmitting information, not to improve computational efficiency.

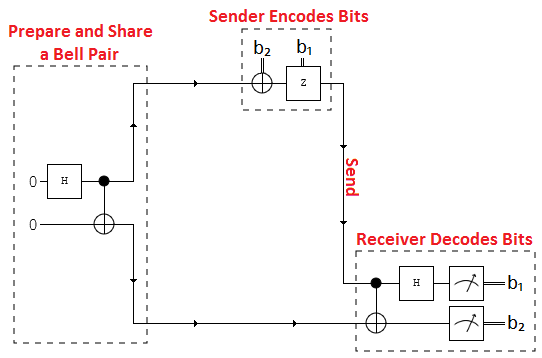
This does not seem to improve the number of classical bits versus qubits needed - 2 qubits needed to transmit 2 [=2^(2-1)] classical bits.

This is true, but shouldn't be surprising: it is a known result that $n$ qubits cannot be used to store more than $n$ bits of information. To devise a supersuperdense coding scheme to transmit more than two bits of information with only two qubits, would mean to find a way to encode and decode more than two bits of information in the overall state of two qubits, and we know that this is not possible.

Nonetheless, the superdense coding protocol does provide advantages with respect to the classical case. Indeed, the qubit used as channel can be generated and shared a long time before the communication begins, and just be kept "in store" for whenever Alice and Bob feel the need to use it.

When they finally decide to communicate, they can now "compress" two bits of information into a single qubit, thus effectively doubling their channel capacity, at the cost of "consuming" the pre-shared qubit.

In other words, you can think of the superdense protocol as a way to "preload" the communication, in order to make it more efficient in the future. What is neat and "quantum" about this is that it can be done without any assumption on the actual information that will be transmitted later. This would not be possible in a classical context.