Why lattice constant (unit cell) is always a parallelepiped?

Question

Wikipedia defines lattice constant as physical dimension of unit cells in a crystal lattice.

Unit cell is defined as:

The unit cell is defined as the smallest repeating unit having the full symmetry of the crystal structure.[4] The geometry of the unit cell is defined as a parallelepiped

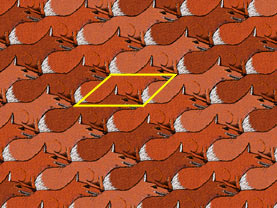
Why that elementary pattern that repeats itself in crystals is always a parallelepiped? In real life such elementary repeating unit/part/block is very often not a parallelepiped at all!

Is this some abstraction and a "bounding parallelepiped" is meant - the one into which definitely fits whatever real elementary repeating pattern is?

Answer 1

The key point here is that if you have a tiling of the plane using

an elementary pattern that repeats itself,

then the pattern itself as originally given might indeed not be a parallelepiped, like the big-square-joined-with-a-smaller-square lumpy shape that's used to produce the following tiling,

^{Image source}

but, even in those cases, there always exists an alternative rearrangement of the pattern that can reproduce the entire tiling using a parallelepiped-shaped unit cell. This simpler unit cell might not be unique in its shape or contents, but the existence of a parallelepiped-shaped unit cell is always guaranteed by the periodicity of the tiling. In the case above, here are two viable options,

^{Image source}

and generally speaking, no matter how complicated an Escherian tiling pattern you're working with, if it is periodic, then you're always guaranteed the existence of at least one way to cut and trim and re-arrange your basic unit pattern into a box with parallel sides:

^{Image source}

Answer 2

Any shape that can be indefinitely extended along three axes (the definition of the "unit cell" is the thing that, when repeated, fills space) can be mapped to a parallelepiped.

Individual atoms may or may not be on the corners of the parallelepiped - but the "bounding box" that contains atoms at the same (relative) location on every instance must be a parallelepiped.

See this drawing (2D) for an example of why that must be so (and it does confirm your intuition of the "bounding parallelepiped"):

Note - it is possible to have a "different" shape for certain specific cases (like a hexagonal lattice, certain "Escherian" shapes) - but that can ALSO be mapped to a parallelepiped (which is therefore a more sensible general shape, because it always works):

Answer 3

The key feature of a crystal lattice its its translational symmetry corresponding to a Bravais lattice. This means that a given pattern repeats in 3D-space with 3 (linearly independent) translational lattice basis vectors $\vec a_1$, $\vec a_2$, $\vec a_3$. These basis vectors are not unique but they always define a parallepiped which constitutes a unit cell of the lattice. Therefore the repeating pattern of a crystal is always connected with the parallelepiped of a unit cell.