How does a rigid body constiting of two particles rotate?

Question

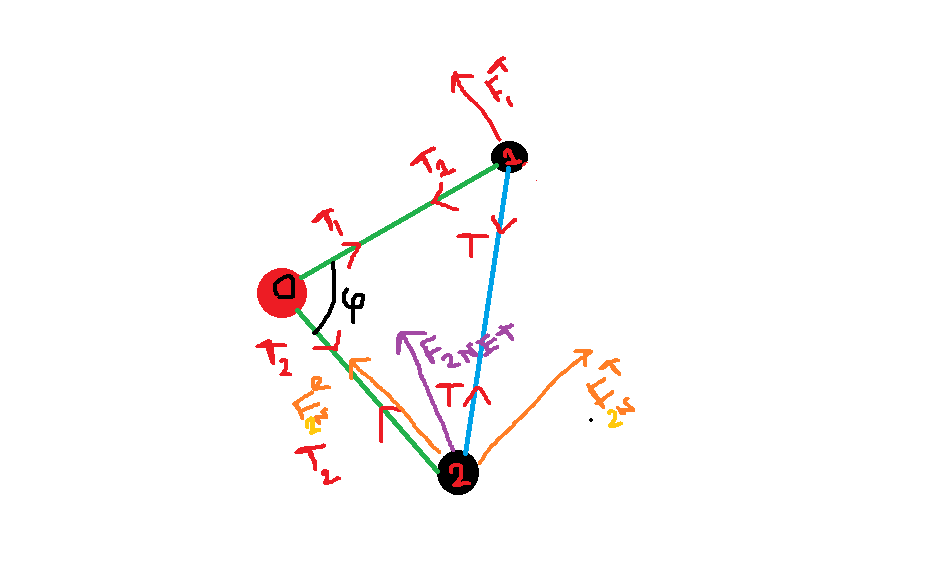
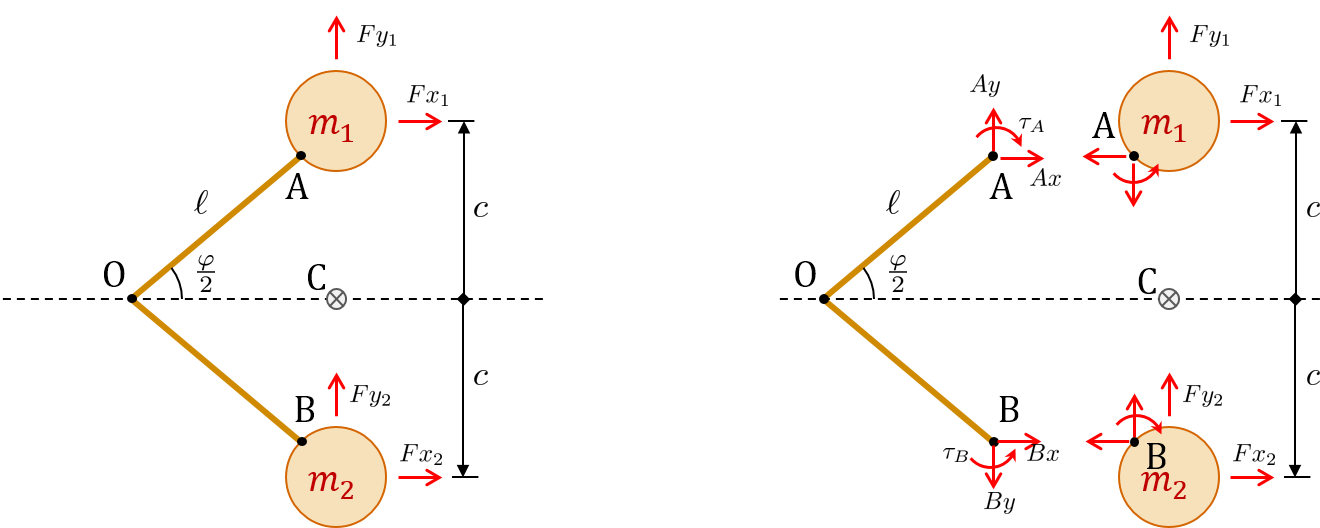
Suppose you have an axis $O$, and two masses $m_1$ and $m_2$, which are joined to $O$ by a rod of $r_1$ and $r_2$, and $m_1, m_2$ are joined by $r$ (rods are maseless and rigid). Now, $\angle m_1Om_2 = \phi$, and you push $m_1$ by tangential force $F_1^T$. They're shown in the picture.

Now, as the entire configuration is rigid and a force is applied, both the masses will rotate with the same angular velocity (possibly varying over time). As per my understanding, the only forces acting on $m_2$ is the tension $\vec{T_2}$ on the rod $Om_2$ and the tension $\vec{T}$ on $m_1m_2$ rod, (which are directed towards $O$ and $m_1$ respectively) which combines for $\vec{F_{2NET}}$. I have broken $F_{2NET}$ into radial component $F_2^r$ (which is useless), and tangential component $\vec{F_{2N}^{T}}$. Now, $\vec{F_{2N}^{T}}$ will be only force that contributes to $m_2$'s rotation.

Now the thing that I don't understand is that when $\phi \rightarrow 180$, to get a considerable amount of tangential component $\vec{F_{2N}^{T}}$, atleast one of $T, T_2$ has to be very very large (should be infinity when $\phi = 180$). But isn't this physically impossible ? What's more confusing to me is that I can make this makeshift model using pencil and clay lumps and it rotates perfectly even when $\phi \approx 180$ .

Answer 1

Note that tension may not be the only force being transmitted through the links. If the links between the masses are in indeed inextensible and non-flexible, which it should be if the above system is a rigid body, shears forces (internal forces oriented perpendicular to the direction of the link) and bending moments (internal moments whose axes are perpendicular to the link) may be present. Elastic beam theory (such as Euler-Bernoulli beam theory) might prove insightful how these shear forces and bending moments develop in real-life links and bars.

Answer 2

Try a simpler case. Attach a clay lump to a pencil. Now shake the pencil around, left, right, and all around. If the force on the clay lump is only directed along the pencil as you claim, then the clay lump will only accelerate in the direction of the pencil. But clearly you can move the clay lump in any direction you want. The answer is that the pencil's force is not necessarily directed on along the line of the pencil.

Answer 3

If the line of action of the applied force is not directed through the pivot then the system will rotate. It does not make any difference what the angle is between the rods.

You can think of the framework as a rigid body. You do not need to consider internal forces. The applied force provides a torque about the pivot. If there is a torque, the system will rotate.

If the applied force is directed through the pivot point O then there is no torque and the system will not rotate.

Answer 4

Mass 2 will move even when phi = 180 degrees if you push mass 1.

Qualitatively, the short answer is constraints. When you move mass 1, the rod connecting mass 1 and mass 2 has to move along with it (the rod can't break or bend or stretch), which in turn causes mass 2 to move. (Does this imply that tension does not always act along the rod? Becuase otherwise how would it create a torque on mass 2? Someone please answer this in the comments.)

Quantitively, I do not think I can find the torque on mass 2 by considering the forces on it, which is what you ask (right?). What you can do, however, is calculate it indirectly:

Step 1: Find the angular velocity of mass 2 wrt O - it should be equal to that of mass 1. This implies that angular acceleration of mass 2 about is equal to that of mass 1. (I'm not entirely sure about the second line of this step.)

Step 2: Use torque = I*alpha to find the torque on mass 2.

PS: Sorry if the formatting is not up to scratch. I find SE tools somewhat confusing.

Answer 5

As @SprocketsAreNotGears mentioned, your assumption of forces constrained to the lines OM2 and M1M2 is wrong:

rods are not ropes

A rod can exert a force perpendicular to its length. An idealized rope cannot. A rod is a rigid body. A rope is not.

As the simplest possible counter example, imagine a ball attached to a stick and held out parallel to the ground. The rod exerts a force up on the ball counteracting gravity even if the rod’s length is perpendicular to up.

Once you remove the constraint on T2 and T1 the infinities at $\phi = 180$ go away.

Answer 6

What you are missing from the free body diagrams are the moments that the rods can apply to the masses. You have substituted the moments with a virtual member connecting the two masses and a tension between them. In term, when $\varphi=\pi$ all three points are colinear, making the production of moments impossible.

You are looking at a situation like this:

where at point A, for example, the forces $A_x$, $A_y$ and moment $\tau_A$ is applied to the massless rod, and an equal and opposite set is applied to mass (1). Similarly, at point B, the forces $B_x$, $B_y$ and moment $\tau_B$ apply to the massless rods.

Since the rods are massless you need to balance out the forces & moments.

$$ \pmatrix{A_x \\ A_y } + \pmatrix{B_x \\ -B_y} = \pmatrix{0\\0} \\ -\tau_A -c \,A_x + \tau_B + c\,B_x = 0 $$

You also need to form equations of motion for the two masses (a total of 6) for a total of 9 equations. The unknowns are the 6 internal forces/moments at A and B and the 3 degrees of freedom, most likely the motion of the center of mass (point C above).

9 equations and 9 unknowns make for a solvable linear system.

---

If the rods cannot apply any moments because they are pinned, then you don't have a rigid body. In this case, the point masses don't have a rotational degree of freedom and your problem is only solvable if the member AOB is treated like a two force member.