Balancing forces is useful, but it is not enough. There aren't enough constraints on the problem to find all the forces just by setting the net force to zero. In your diagram, you could add any value to $\vec{F_h}$. As long as you add the same to $\vec{T}_c$, the net force doesn't change. So find some sum of forces that comes to zero, then add 1 Newton to both horizontal forces on the problem, and you have a new zero-net-force solution.
On the other hand, as long as the net force and net torque are both zero on a stationary rigid object, it will stay stationary. We need both because net force only tells us about the acceleration of the center of mass. If a beam starts spinning around its center, it has zero net force on it (because the center of mass isn't moving), but there was some net torque that got it spinning. So setting $F_{net} = 0$ is not enough.
As for what happened in your work, it's hard to tell. First, try to stick with well-defined notation. This will make it much easier for people to understand what you mean. Your question defines the variables $\vec{T}_c$, $\vec{F}_v$, and $\vec{F}_h$, which you didn't use. Your proposed solution uses the undefined variables "Fhx" "Fhy" and "Ft". Then you write about "theta" without defining it, either.
My best guess is that you assumed that the net force from the wall on the beam acts along that beam. That is, in the notation of the problem, you assumed
$$\frac{\vec{F}_v}{\vec{F}_h} = \frac{a}{b}$$
but there is no reason to assume this. That sort of assumption works for strings or ropes, which can support any shear, but for beams, the wall can exert forces in the horizontal and vertical directions independently. You won't know how big those forces are unless you impose both the condition that the net force on the beam is zero, and the condition that the net torque on the beam is zero.
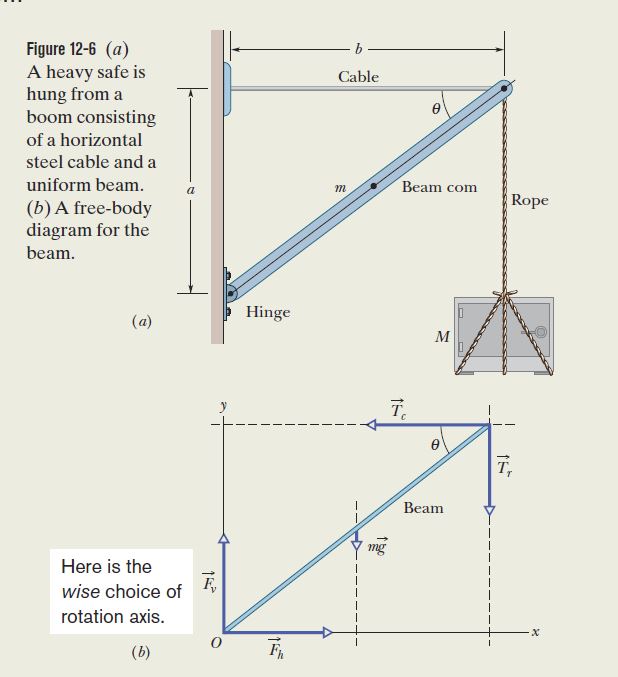 You are asked to find the magnitude of the tension in the cable (the magnitude of the force from the beam on the cable). With an axis of rotation at the hinge so as to eliminate any forces at that point, the solution is completed by balancing torques:
You are asked to find the magnitude of the tension in the cable (the magnitude of the force from the beam on the cable). With an axis of rotation at the hinge so as to eliminate any forces at that point, the solution is completed by balancing torques:
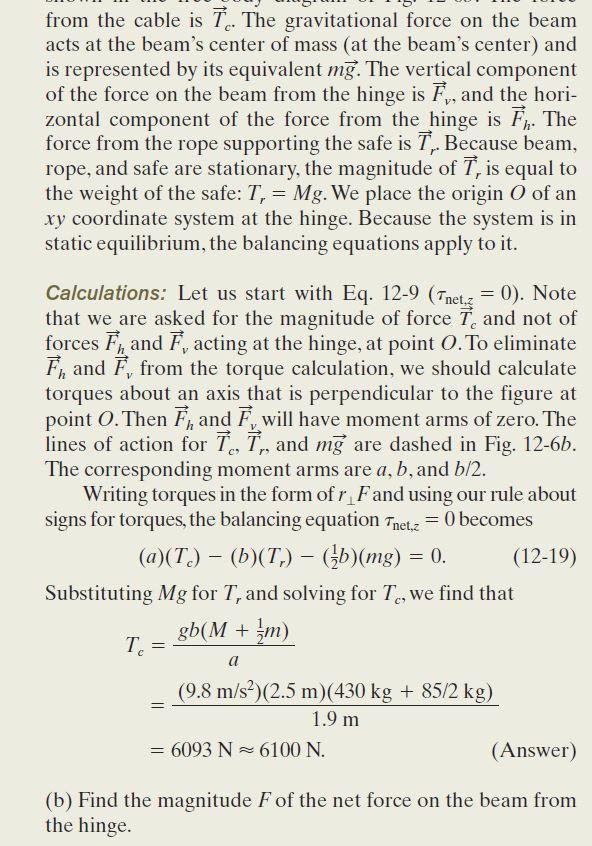 I understand why that works, but I'm not sure why you can't instead balance forces as was done in previous problems. In other words:
I understand why that works, but I'm not sure why you can't instead balance forces as was done in previous problems. In other words: