Voltage drop across an inductor [closed]

Question

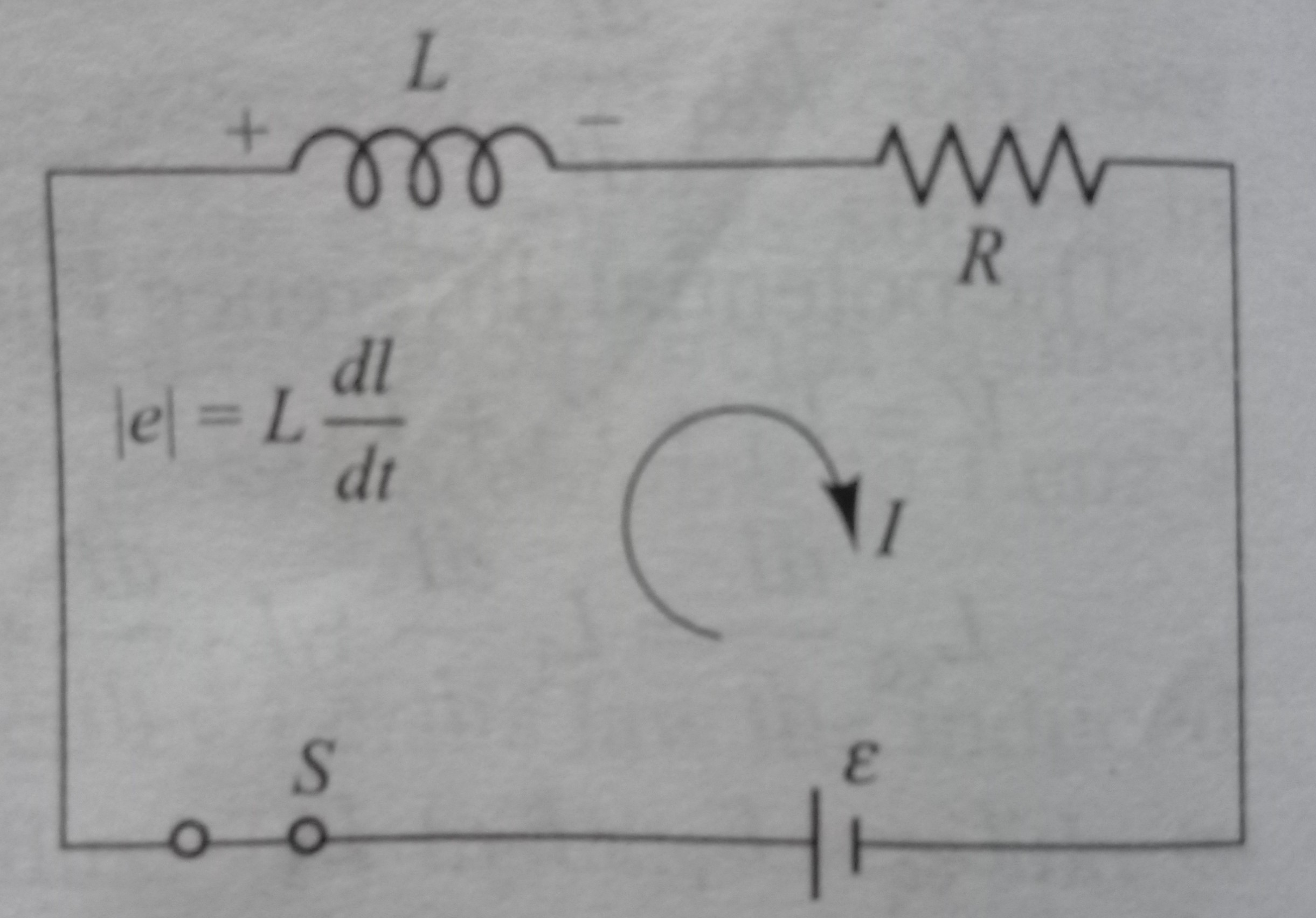
An inductor should oppose the current right, so shouldn't the voltage drop across the inductor be in the opposite direction as that shown in the figure?

Answer 1

An inductor should oppose the current right

No, that's not right. An (ideal) inductor 'opposes' a change in current but offers no opposition to a fixed current, i.e., the voltage $e$ across the inductor is zero for constant $I$.

so shouldn't the voltage drop across the inductor be in the opposite direction as that shown in the figure?

The $+$ and $-$ signs are there to indicate the reference polarity of the voltage across the inductor. The voltage $e$ can be positive or negative and, according to the drawing, if $e$ is positive, the left-most inductor terminal is positive with respect to the right-most terminal. But, if $e$ is negative, the left-most terminal is negative with respect to the right-most terminal.

As the circuit is drawn, if $I$ is increasing, the inductor voltage $e$ should be positive to oppose the increase of $I$.

---

From the comments:

But the inductor opposes the change in current by inducing an emf opposite to the direction of flow of current, isn't it?

Why? If $I$ is increasing (decreasing), the voltage across the inductor $e$ is positive (negative) regardless of whether the instantaneous value of $I$ is negative or positive.

If you solve the differential equation for the current, you'll find that

$$I(t) = \frac{\mathcal{E}}{R} + \left(I_0 - \frac{\mathcal{E}}{R}\right)e^{-tR/L}$$

where $I_0 = I(0)$ is the instantaneous value of the current when $t = 0$.

The voltage across the inductor is then

$$e = L\frac{dI}{dt} = \left(\mathcal{E} - I_0R\right)e^{-tR/L}$$

If you stare at that a bit, you'll see that voltage across the inductor is strictly positive for $I_0 < \frac{\mathcal{E}}{R}$ and strictly negative for $I_0 > \frac{\mathcal{E}}{R}$.

So, if the current is initially negative, the current will increase through zero to become positive (asymptotically approaching the steady state value $I_\infty = \frac{\mathcal{E}}{R}$) but the voltage across the inductor will always be positive.

In other words, $e$ will not change signs even though $I(t)$ does.