How does an aspirator pump work?

Question

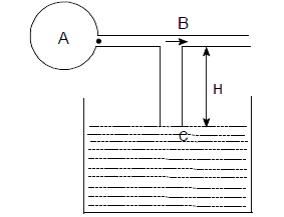
The below picture is of a aspirator pump. If we press the bulb at A the air in it with density $\sigma$ will flow out with velocity $V$. This would cause lower pressure at B and hence the liquid with density $\rho $ will come up. The Gauge pressure is $P$.

I don't understand how this works. The term 'Gauge Pressure' confuses me. It isn't the pressure at A nor it is the pressure at B, so what is it?

Now I apply Bernoulli's principle for the air. So the pressure at A equals the pressure at B + $\frac 12 \sigma V^2$, right? But below the text I find this equation.$$ P_B + \frac 12 \sigma V^2 = P_A + P$$ How does gauge pressure enter this equation?

Now I try to find the excess pressure at B. So it should be $ P_B + \rho gH = P_C $ (excess pressure at a depth). But I find this equation. $$ P_B=P_A - \rho gH $$ Where does $ P_A $ come from?

Answer 1

The diagram and text does seem to be a bit confusing. Here's my best guess at interpreting the diagram and text: The $P$ is actually the ambient atmospheric pressure. In order for the first equation that you wrote down to make sense, the pressure $P_A$ then has to be the gauge pressure of the rubber bulb on the left (i.e, the pressure of the air in the bulb measured with respect to atmospheric pressure), and $P_B$ is the absolute pressure of the air at point B.

Now the pressure differential which determines the height of the liquid in the column is $P-P_B$. If this quantity is positive, the liquid in the column goes with respect to the level of the liquid in the surrounding container. If this quantity is negative, the liquid in the column goes down with respect to the surrounding liquid level.

From your first equation, we then have $P-P_B=(1/2){\sigma}V^2-P_A$, and so it follows that ${\rho}gH=(1/2){\sigma}V^2-P_A$, where H is the height of the liquid in the column. This seems to make sense. If the right end of the open tube is stopped up so that little or no air can flow out the right end of the tube, then the velocity V is negligible or zero and the height H is determined by just the gauge pressure pressure $P_A$ in rubber bulb. Note that in this case that squeezing the bulb makes the liquid in the column go down (i.e., H<0), as expected. If, on the other hand, the right end of the open tube is left completely open, then the right side of the equation is dominated by the $(1/2){\sigma}V^2$ term and the column of liquid rises (i.e., H>0).

Answer 2

Edit 2019/7/24: Gauge pressure is pressure relative to the current atmospheric pressure. You use either all absolute pressure or gauge pressure in Bernoulli's EQUATION.

All this math is quite pointless because the conditions assumed are flawed. The pressure at A is higher than B in order to get the air flowing. The pressure at "B" is 'essentially' atmospheric. As stated in one comment, adding tubing causes bubbles to be blown more violently. The viscous 'drag' of the exit pipe causes the pressure at "B" to go higher the longer that tube is made. So the faster the flow, the higher the pressure inside the tube at B goes. ... Build one as drawn and you will find that all it does is blow bubbles. The pressure is not lower than ambient in the horizontal tube. The bulb has higher than ambient in it when squeezed as does the entire tube. there is NO accelerating air at the top of the "T", therefore no pressure drop.

See this Millersville page for a better explanation. http://www.millersville.edu/physics/experiments/093/index.php