There is a test of the collapse of a magnetized neutron star (case 3) in this preprint: http://arxiv.org/abs/arXiv:1208.3487.
Here is some of the text and figures from that paper that describe how the magnetic field is expelled:
- Magnetized Collapse to a Black Hole
Our final and most comprehensive test is represented by the collapse
to a BH of a magnetized nonrotating star. This is more than a purely
numerical test as it simulates a process that is expected to take
place in astrophysically realistic conditions, such as those
accompanying the merger of a binary system of magnetized neutron stars
[26, 27], or of an accreting magnetized neutron star. The interest in
this process lays in that the collapse will not only be a strong
source of gravitational waves, but also of electromagnetic radiation,
that could be potentially detectable (either directly or as processed
signal). The magnetized plasma and electromagnetic fields that
surround the star, in fact, will react dynamically to the rapidly
changing and strong gravitational fields of the collapsing star and
respond by emitting electromagnetic radiation. Of course, no
gravitational waves can be emitted in the case considered here of a
nonrotating star, but we can nevertheless explore with unprecedented
accuracy the electromagnetic emission and assess, in particular, the
efficiency of the process and thus estimate how much of the available
binding energy is actually radiated in electromagnetic waves. Our
setup also allows us to investigate the dynamics of the
electromagnetic fields once a BH is formed and hence to assess the
validity of the no-hair theorem, which predicts the exponential decay
of any electromagnetic field in terms of Quasi Normal Mode (QNM)
emission from the BH.
...
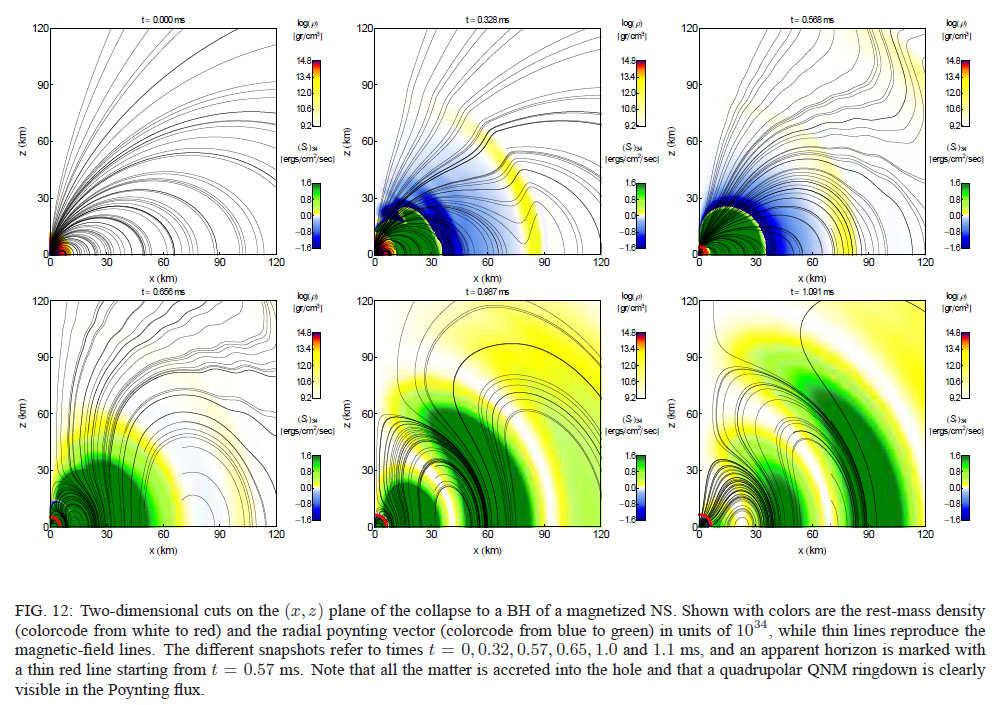
As the collapse proceeds, the restmass density in the center and the
curvature of the spacetime increase until an appararent horizon is
found at t = 0.57 ms and is marked with a thin red line in Fig. 12.
As the stellar matter is accreted onto the BH (the rest-mass outside
the horizon $M_{b, out} = 0$ is zero by $t \geq 0.62$ ms), the external magnetic
field which was anchored on the stellar surface becomes disconnected,
forming closed magneticfield loopswhich carry away the electromagnetic
energy in the form of dipolar radiation. This process, which has been
described through a simplified non-relativistic analytical model in
Ref. [52], predicts the presence of regions where |E| > |B| as the
toroidal electric field propagates outwards as a wave. This process
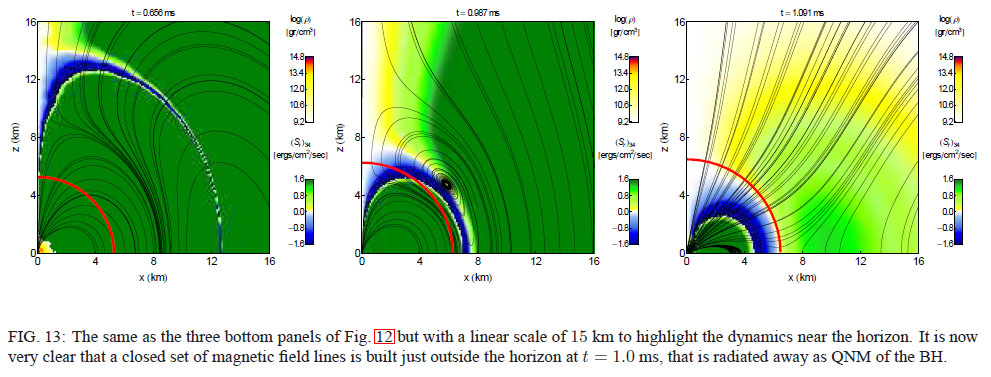
can be observed very clearly in Fig. 13, which displays the same three
bottom panels of Fig. 12 on a smaller scale of only 15 km to highlight
the dynamics near the horizon. In particular, it is now very clear
that a closed set of magnetic field lines is built just outside the
horizon at t = 1.0 ms, that is radiated away. Note also that our
choice of gauges (which are the same used in [61]) allows us to model
without problems also the solution inside the apparent horizon. While
the left panel of Fig. 13 shows thatmost of the rest-mass is
dissipated away already by t = 0.65 ms (see discussion in [62] about
why this happens), some of the matter remains on the grid near the
singularity, anchoring there the magnetic field which slowly evolves
as shown in the middle and right panels.