Intercept a moving object

Question

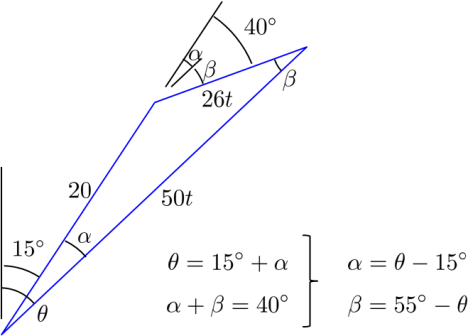
Object A can move at 50km/h, wants to intercept object B (currently $15^{\circ}$, east of north from A) moving at 26km/h, $40^{\circ}$ east of north. What angle should A take to intercept B? AB is 20km apart

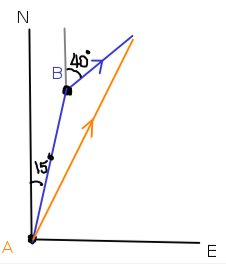
The provided answer looks like:

---

Choose x axis along 20km distance.

$26t \sin{(40-15)} = 50t \sin{\theta}$

$\theta = \sin^{-1}{\frac{11}{50}} = 12.7$

$15 + 12.7 = 27.7$

---

I took a different approach and used $\cos$ and got a different answer ... why is that?

$26t \cos{(40-15)} = 50t \cos{\theta}$

Answer 1

Your equation is almost correct.

Updated

Using x-axis along AB

Taking the x-axis along AB yields

$$ 50 t \sin (\theta-15^\circ) = 26 t \sin(40^\circ) $$ $$ \sin (\theta-15^\circ) = 0.52 \sin(40^\circ) $$

$$ \theta = 34.527^\circ $$

$$ \cos (\theta-15^\circ) = \sqrt{1-\sin^2 (\theta-15^\circ) } $$

and the y-axis perpendicular to AB

$$ 50 t \cos(\theta-15^\circ) = 20 + 26 t \cos(40^\circ) $$

$$ t = 0.735 $$

Using x-axis along AC (interception pt)

taking y-axis perpendicular to AC

$$ 50 t = 20 \cos(\theta-15^\circ)+26 t \cos(55^\circ-\theta) $$ $$ t = \frac{20 \cos(\theta-15^\circ)}{50 - 26 \cos(55^\circ-\theta)} $$

taking x-axis along AC $$ 20 \sin(\theta-15^\circ)=26 t \sin(55^\circ-\theta) $$

which when expanded you need to solve an equation of the form $$A\cos \theta + B \sin \theta = C$$ for $\theta$ with the same results as above.