Will "A" experience max static friction if "A" is connected to "B" which is on the verge of slipping?

Question

I'm going to explain the question by stating a problem.

Homework Problem

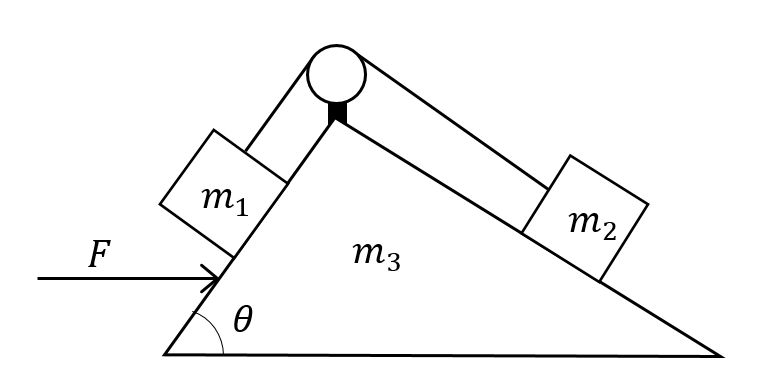
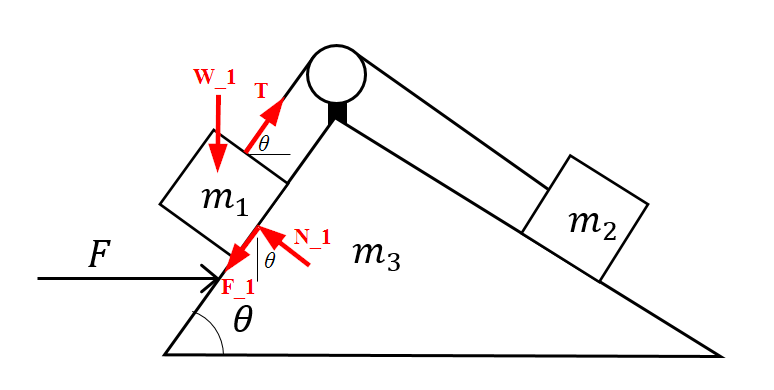
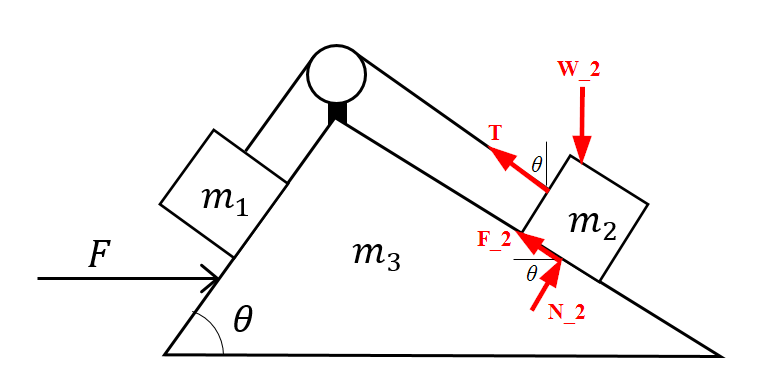
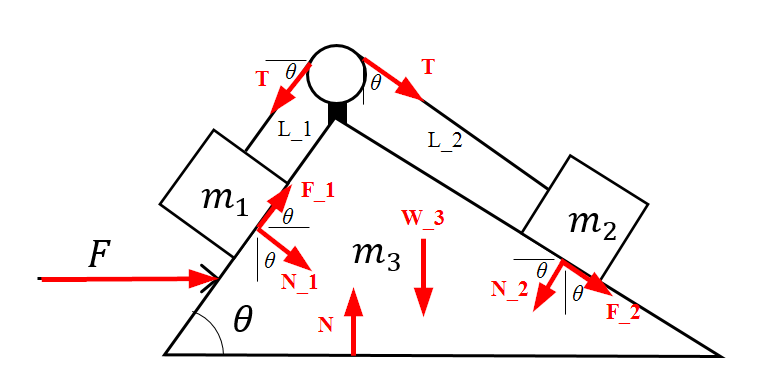
Consider this system:

How big horizontal force $F$ should be, so that the mass $m_1$ would be on the verge of slipping upwards? (Friction coefficients between objects is $\mu_s$ but $m_3$ has no friction with the ground. Ropes and the pulley are perfect ie. weightless, frictionless, non-streching).

My Attempt

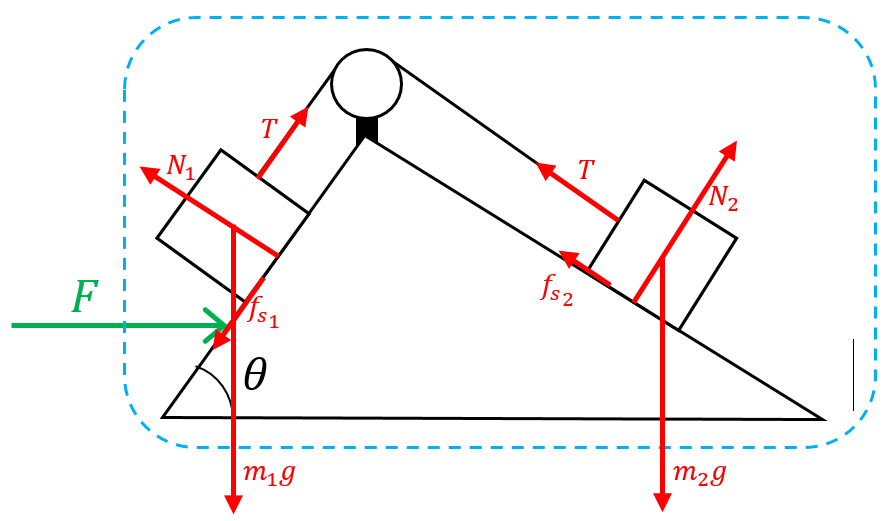
Firstly I drew important forces:

$m_1$ and $m_2$ are not moving on $m_3$. Therefore all the objects have the same acceleration $a$ which is easily found: $$a_x=\frac{F}{\Sigma m}$$ $$a_y=0$$ Now write equations of motion for $m1$ and $m2$: $$[m_1,x]: T\cos\theta-N_1\sin\theta-{f_s}_1\cos\theta=\frac{F}{\Sigma m}m_1$$ $$[m_1,y]: T\sin\theta+N_1\cos\theta-{f_s}_1\sin\theta-m_1 g = 0$$ $$[m_2,x]: -T\sin\theta+N_2\cos\theta-{f_s}_2\sin\theta=\frac{F}{\Sigma m}m_2$$ $$[m_2,y]: T\cos\theta+N_2\sin\theta+{f_s}_2\cos\theta-m_2 g=0$$ Since $m_1$ is on the verge of slipping upwards, ${f_s}_1=\mu_s N_1$ and re-write equations: $$[m_1,x]: T\cos\theta-N_1\sin\theta-\mu_s N_1\cos\theta=\frac{F}{\Sigma m}m_1$$ $$[m_1,y]: T\sin\theta+N_1\cos\theta-\mu_s N_1\sin\theta-m_1 g = 0$$ $$[m_2,x]: -T\sin\theta+N_2\cos\theta-{f_s}_2\sin\theta=\frac{F}{\Sigma m}m_2$$ $$[m_2,y]: T\cos\theta+N_2\sin\theta+{f_s}_2\cos\theta-m_2 g=0$$ At this point we are left with 4 equations and 5 unknowns ($T$, $N_1$, $N_2$, ${f_s}_2$, $F$).

Now I'm wondering if this is an indeterminate problem or I am missing something? I have thought of finding more equations (elasticity?) but no luck.

I asked two professors to solve this and they could do because they took it for granted that ${f_s}_2=\mu_s N$ which I don't seem to understand why should be necessarily the case. After all my equations do have answers if ${f_s}_2<\mu_s N$ so what stops it from happening? I mean, how does one conclude from $m_1$ being on verge of slipping to $m_2$ experiencing ${f_s}_\max$? And if the argument is wrong, how to solve this problem?

What I think

I think that it has something to do with elasticity. I have realized that sometimes under/over-determinacies are caused by our assumptions:

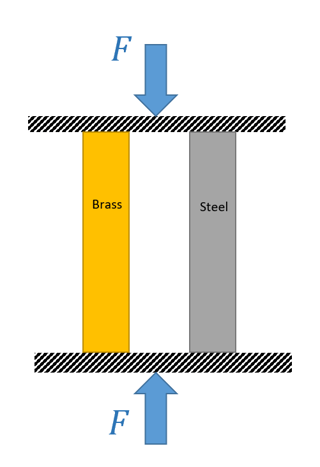
Example #1 (left): Find internal force in each rod. Under-determinate unless we take elastic deformations into account.

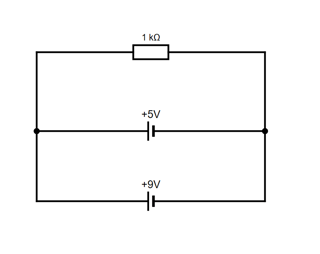
Example #2 (right): Find electric current in the resistor. Over-determinate unless we take internal resistance of batteries into account.

Motivating from these, I tried applying elastic equations:

$$T=-k\Delta l$$

Now, I don't know what to do next. My best guess is that I should stop here and say, $k\Delta l$ could be anything, so the problem is unsolvable unless they tell us the value of initial tension in string. Does this make any sense?

Close Related Problem

Skip this last section. I wanted to edit it out but Sammy's answer already points to this.

When writing this question, another indeterminate problem came into my mind which I found related to this one (not sure tough). This is not my main question. Take this system which is in static equilibrium:

What is ${f_s}_1$ and ${f_s}_2$?

I think we are facing some similar indeterminacy here.

Answer 1

In your 2nd problem,

Case 1: 0 < F $\le \mu$_s2m₂g
f_s1 = 0 and f_s2 = F

Case 2: $\mu$_s2m₂g $\le$ F $\le \mu$_s1m₁g + $\mu$_s2m₂g
f_s1 = F - $\mu$_s2m₂g and f_s2 = $\mu$_s2m₂g

Case 3: F > $\mu$_s1m₁g + $\mu$_s2m₂g
I think we all know, there will be dynamic friction instead.

To answer your 1st question,
As you have asked to find maximum F, in that case you should take f_s2 as f_s2,max.
If, instead, you had asked to get minimum F, I think you should take f_s2 as zero.

Answer 2

Quick summary of a long answer:

• This is a statics problem with 4 unknowns and 4 equations: $T$ is an internal force that is not required for the solution
• $T$ is either positive, meaning the blocks 1 and 2 are pulling away from each other; or zero, meaning they are moving towards each other ($T \ge 0$ because you can't push on a rope)
• If $T = 0$, then the two blocks can be treated independently
• If $T > 0$, then the blocks must move together (this is the case assumed by the OP's professors), but $T$ does not need to be found
• To determine whether $T$ is zero or positive, consider the force $F$ that would cause either block 1 or block 2 to move in isolation, and the direction of that movement: if it is towards the other block then $T = 0$, otherwise $T > 0$ and they move together

---

As is often the case, the problem can be simplified conceptually by changing the reference frame. The forces on each block can be decomposed into normal and frictional components and the accelerations are recast as inertial forces. In the figure below, the plane defining the top and left of block 3 is shown as a straight line and the acceleration and gravitational forces are rotated appropriately:

Notes:

• Acceleration $a$ is used in place of applied force $F$ for convenience ($a = F / (m_1+m_2+m_3)$)
• Line tension $T$ is an internal force that does not need to be found; we only need to confirm that it is non-negative (no pushing on ropes)
• The original question asked when block 1 would slip up (right in this diagram), but in reality the block will slip down/left: the solution presented here assumes motion down/left
• Block 1 may slip without any external force/acceleration depending on $m_1$, $m_2$, $\mu_s$, and $\theta$.

The equations for force equilibrium in the "n" direction are:

$$ \begin{array} {} N_1 = m_1 g \cos\theta - m_1 a \sin\theta \ge 0 && (1) \\ N_2 = m_2 g \sin\theta + m_2 a \cos\theta \ge 0 && (2) \\ \end{array} $$

Note that the normal forces must be greater than or equal to zero. Otherwise, the block has lost contact with the surface. The limiting accelerations before each block loses contact are:

$$ \begin{array}{} a_{1n} \le {g/\tan\theta} && (3) \\ a_{2n} \ge -g/\tan\theta && (4) \\ \end{array} $$

The equilibrium equations in the "f" direction depend on the state of the line between the blocks. If it is in tension, then the blocks must move together in the friction direction. If not the blocks move independently because you can't push on a rope. Start by assuming the blocks move independently:

$$ \begin{array} {} F_1 = m_1 a \cos\theta + m_1 g \sin\theta && (5) \\ F_2 = m_2 a \sin\theta - m_2 g \cos\theta && (6) \\ \end{array} $$

At the onset of slipping of block 1 or block 2, we know that $F_1 = \mu_s N_1$ or $F_2 = \mu_s N_2$, respectively. Subsituting the limiting friction into (5) and (6) provides the accelerations that would cause slipping on either block in isolation:

$$ \begin{array} {} a_{1r} = g\left[\frac{\mu_s\cos\theta - \sin\theta}{ \mu_s\sin\theta + \cos\theta} \right] && (7) \\ a_{2r} = g\left[\frac{\mu_s\sin\theta + \cos\theta}{-\mu_s\cos\theta + \sin\theta} \right] && (8) \\ \end{array} $$

If $a_{1n} \lt a_{1r}$, then block 1 loses contact will lose contact with the surface rather than sliding. There will be no friction against block 1 because $N_1=0$, so it will move when friction is overcome on block 2. That is, block 1 moves when $a \ge a_{2r}$.

Otherwise, since block 1 is moving away from block 2, we know the line between the blocks will be in tension. Friction must be overcome on both blocks before either moves ($F_1 = \mu_sN_1$ and $F_2=\mu_sN_2$). This is the case assumed by the OP's professors. Here both blocks slip at the same acceleration $a_{12}$, which is calculated below:

$$F_1 + F_2 = m_1a_{12}\cos\theta + m_1g\sin\theta + m_2a_{12}\sin\theta - m_2g\cos\theta$$

$$\mu_s\left[N_1 + N_2\right] = a_{12}(m_1\cos\theta + m_2\sin\theta) + g(m_1\sin\theta - m_2\cos\theta)$$

After a bunch of algebra...

$$ \begin{array}{} a_{12} = g\frac{(m_1\sin\theta - m_2\cos\theta) - \mu_s(m_1\cos\theta + m_2\sin\theta)} {\mu_s(-m_1\sin\theta + m_2\cos\theta) - (m_1\cos\theta + m_2\sin\theta)} && (9) \end{array} $$

Both blocks move together when $a \ge a_{12}$.

---

The original question asked when block 1 would be on the verge of slipping. We could also consider block 2:

• With positive $F$, $N_2$ will always be greater than zero ($\therefore$ do not need to consider equation 4)
• If $a_{2r} < a_{1r}$, then block 2 will slip towards block 1 and will move independently (because there will not be tension in the line between them)
  • Block 2 will therefore move when $a \ge a_{2r}$
• If $a_{2r} \ge a_{1r}$, then block 1 will slip away from block 2, the line will remain in tension, and the solution in equation 9 will apply

Answer 3

Your professors are correct about your 1st problem. If $m_1$ is on the point of moving upwards then $m_2$ must be capable of moving downwards, because $m_1$ cannot move unless $m_2$ also moves. So the limit of static friction on $m_2$ must have been reached already.

In the 2nd problem also, if either block is on the point of moving then the friction force on both blocks must have reached the static limit, because one cannot move without the other. If the blocks are not on the point of moving then the problem is indeterminate. We can say that $F=f_{s1}+f_{s2}$ but we cannot say how the force $F$ is divided between $f_{s1}$ and $f_{s2}$.

Answer 4

You have three free body diagrams, and you forgot to add the resultant on the pulley acting on body 3. All bodies move with the same horizontal acceleration $$\ddot{x} = \frac{F}{m_1+m_2+m_3}$$ and zero vertical acceleration.

$$ \begin{bmatrix} -\sin\theta & -\cos\theta \\ \cos\theta & -\sin \theta \end{bmatrix} \pmatrix{N_1 \\ F_1} + \pmatrix{T \cos\theta \\ T \sin \theta} + \pmatrix{0 \\ -m_1 g} = m_1 \pmatrix{\ddot{x} \\0} $$

$$ \begin{bmatrix} \cos\theta & -\sin\theta \\ \sin\theta & \cos \theta \end{bmatrix} \pmatrix{N_2 \\ F_2} + \pmatrix{-T \sin\theta \\ T \cos \theta} + \pmatrix{0 \\ -m_2 g}= m_2 \pmatrix{\ddot{x} \\0} $$

$$ \begin{aligned} \pmatrix{F \\ N-m_3 g} = m_3 \pmatrix{\ddot{x}\\0} & + \begin{bmatrix} -\sin\theta & -\cos\theta \\ \cos\theta & -\sin \theta \end{bmatrix} \pmatrix{N_1 \\ F_1} + \pmatrix{T \cos\theta \\ T \sin \theta} + \\ & + \begin{bmatrix} \cos\theta & -\sin\theta \\ \sin\theta & \cos \theta \end{bmatrix} \pmatrix{N_2 \\ F_2} + \pmatrix{-T \sin\theta \\ T \cos \theta} \end{aligned} $$

This looks like 6 equations with 8 unknowns $F,\,T,\,N,\,\ddot{x},\,N_1,\,N_2,\,F_1,\,F_2$ but because of the no-slip condition you also have $F_1 = \mu_s N_1$ and $F_2 = \mu_s N_2$.

Now we have the following 6 equations (in the same sequence as above)

$$ \begin{aligned} (T-\mu_s N_1) \cos\theta - N_1 \sin \theta & = \frac{m_1}{m_1+m_2+m_3} F \\ N_1 \cos \theta + (T-\mu_s N_1) \sin \theta -m_1 g & =0 \\ N_2 \cos \theta - (T + mu_s N_2) \sin \theta & = \frac{m_2}{m_1+m_2+m_3} F \\ N_2 \sin\theta + (T+\mu_s N_2) \cos\theta - m_2 g & = 0 \\ F + (N_1+\mu_s N_2 + T) \sin\theta - (T+N_2 -\mu_s N_1) \cos\theta & = \frac{m_3}{m_1+m_2+m_3} F \\ N-m_3 g -(T+N_1+\mu_s N_2) \cos\theta - (T+N_2-\mu_s N_1) \sin\theta & =0 \end{aligned} $$

The last equation doesn't matter because it is used in the end to find the normal reaction $N = g (m_1+m_2+m_3)$ with the floor.

Use equation (#5) for $$ F = \frac{m_1+m_2+m_3}{m_1+m_2} \left( (T+N_2-\mu_s N_1)\cos\theta - (T+N_1+\mu_s N_2)\sin\theta\right) $$

Use equations (#2) and (#4) to solve for $$\begin{aligned} N_1 & = \frac{m_1 g-T \sin \theta}{\cos\theta-\mu_s \sin\theta} \\ N_2 & = \frac{m_2 g - T \cos\theta}{\sin\theta+\mu_s \cos\theta}\end{aligned}$$ and use in either (#1) and (#3) to solve for $T$. Both equations give the same result:

$$ T = \frac{g\,m_1 \,m_2 (1+\mu_s^2)}{(m_1+\mu_s m_2)\cos\theta + (m_2-\mu_s m_1)\sin\theta} $$

Now you have back substitute to solve for everything. Finally, the force $F$ is estimated with

$$ F = g (m_1+m_2+m_3) \frac{ (m_2-\mu_s m_1)\cos\theta - (m_1+\mu_s m_2) \sin\theta}{(m_1+\mu_s m_2)\cos\theta + (m_2-\mu_s m_1)\sin\theta} $$

Summary:

The assumptions needed are that all parts move in unison, and that for mass #1 to slip, also mass #2 needs to slip since they are connected.