Types of Complex Resistor Networks where series and parallel law combination of resistor doesn't hold

Question

What are the types of complex resistor networks where the series and the parallel law doesn't apply?There are some instances where we simply apply Wye delta conversion because that is the only way a circuit can be simplified.How to detect these kinds of networks?

Answer 1

You can use you basic equivalent resistor formulas ($R_S=R_1+R_2+R_3\dots$ or $\frac{1}{R_p}=\frac{1}{R_1}+\frac{1}{R_2}+\frac{1}{R_3}+\dots$) to replace two or more resistors in a that are all in series or all in parallel. A trick I find helps identifying this case is trying to draw a circle (well actually any closed curve) around the resistors in question. If you can draw your circle so that there are just two wires crossing the circle (think of it as one wire going in and one coming out) you should be able to replace the resistors inside with a single equivalent resistor.

Where you would use the delta or Y conversion is if you are trying to simplify part of a network that you've circled that has three wires crossing the circle. Clearly this can not be replaced by a single resistor but if three resistors in the circle are arranged as a delta or Y you can convert to the other configuration using the applicable formulas.

I hope the examples in the diagrams below help. Notice in the classic bridge circuit (third diagram) there is no way to circle two or more resistors and only have two wires crossing the circle (short of circling the whole network or course).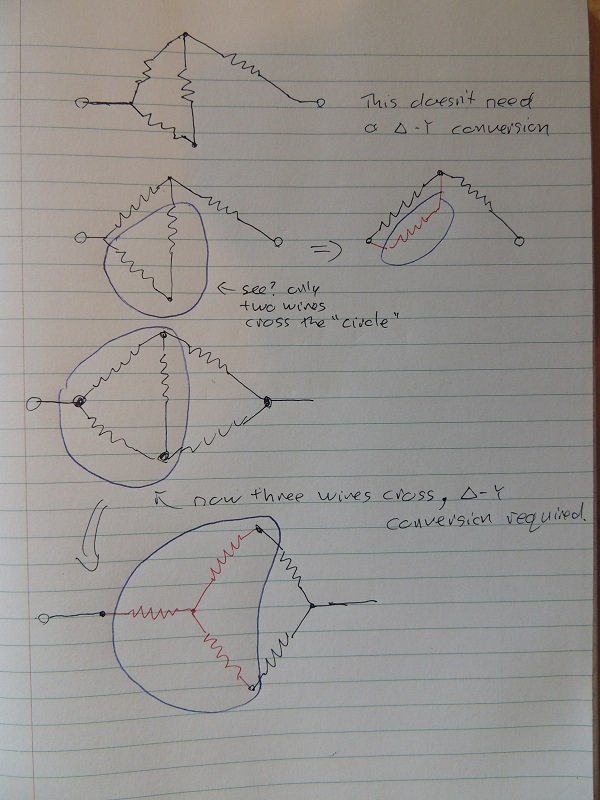

Answer 2

Laws for parallel/series resistances don't hold when resistances aren't linear (i.e. V-I-graph isn't a line through the origin).

Altough it is question able if such components should be called resistors.

EDIT:
An example to make things clear:
Suppose you have two incandescent light bulbs and you are measuring 100mA at 100V across each, i.e. a (well defined!) resistance of 1kΩ for each bulb.
Now if connected in series and with 100V across you would not measure a current of 50mA but considerable more.
Therefore the "law of series combination" (e.g. "current halves if two identical resistances connected in series") does not hold for light bulbs. It is not the case that resistance (=quotient of voltage and current) of light bulbs is not well defined, it is just not independent of voltage (or current). The reason is that V-I relationship in light bulbs is not linear (it goes through the origin but it is not a line; it would also not work out if it was a line, but not going through the origin (e.g. a Thevenin voltage source)).