Suppose an electron is moving through empty space at speed v.
It produces an electric field because it is a charge. But this field changes as it moves. Changing electric field must give rise to magnetic field.
However, the moving electron also constitutes a transient current through various points in space. This must produce some more magnetic field curling around the electron as it moves.
So, the magnetic field here is due to both changing electric fields and currents. Is this true?
The electric $\:\mathbf{E}\:$ and magnetic $\:\mathbf{B}\:$ parts of the electromagnetic field produced by a moving charge $\:q\:$ are :
SOURCE 1
From Jackson's 'Classical Electrodynamics', 3rd Edition, equations (14.14) and (14.13)
\begin{align} \!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\mathbf{E}(\mathbf{x},t) & = \frac{q}{4\pi\epsilon_0}\left[\frac{\mathbf{n}-\boldsymbol{\beta}}{\gamma^2(1 - \boldsymbol{\beta}\boldsymbol{\cdot}\mathbf{n})^3 R^2}\vphantom{\dfrac{\dfrac{a}{b}}{\dfrac{a}{b}}} \right]_{\mathrm{ret}} + \frac{q}{4\pi}\sqrt{\frac{\mu_0}{\epsilon_0}}\left[\frac{\mathbf{n}\boldsymbol{\times}\left[(\mathbf{n}-\boldsymbol{\beta})\boldsymbol{\times} \dot{\boldsymbol{\beta}}\right]}{(1 - \boldsymbol{\beta}\boldsymbol{\cdot}\mathbf{n})^3 R}\right]_{\mathrm{ret}} \tag{14.14}\\ \!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\mathbf{B}(\mathbf{x},t) & = \left[\mathbf{n}\boldsymbol{\times}\mathbf{E}\right]_{\mathrm{ret}} \tag{14.13} \end{align} where \begin{align} \boldsymbol{\beta} & = \dfrac{\boldsymbol{\upsilon}}{c},\quad \beta=\dfrac{\upsilon}{c}, \quad \gamma= \left(1-\beta^{2}\right)^{-\frac12} \tag{01a}\\ \dot{\boldsymbol{\beta}} & = \dfrac{\dot{\boldsymbol{\upsilon}}}{c}=\dfrac{\mathbf{a}}{c} \tag{01b}\\ \mathbf{n} & = \dfrac{\mathbf{R}}{\Vert\mathbf{R}\Vert}=\dfrac{\mathbf{R}}{R}\equiv\dfrac{\mathbf{r'}}{r'}=\dfrac{\mathbf{r'}}{\Vert\mathbf{r'}\Vert}\equiv \mathbf{e}_{r'} \tag{01c} \end{align}
SOURCE 2
From Feynman's Lectures, Volume II 'Mainly Electromagnetism and Matter', New Millennium Edition, equations (21.1) \begin{align} \mathbf{E} & = \frac{q}{4 \pi \epsilon_0} \left[ \frac{ \mathbf{e}_{r'}}{r'^2} + \frac{r'}{c} \frac{d}{dt} \left(\frac{\mathbf{e}_{r'} }{r'^2}\right) + \frac{1}{c^2} \frac{d^2}{dt^2} \mathbf{e}_{r'} \right] \tag{21.1-Feynman}\\ c\mathbf{B} & = \mathbf{e}_{r'}\boldsymbol{\times}\mathbf{E} \nonumber \end{align}
Without looking at the details it seems from the second equations, those giving $\:\mathbf{B}\:$ from $\:\mathbf{E}\:$, that the former is produced by the latter. But this is not the case : the electromagnetic field is an entity and its separation to the electric and magnetic parts depends upon the observer's inertial frame of reference.
Note that these equations are derived from the so called Liénard-Wiechert potentials which in turn are produced from the Maxwell's equations in empty space
\begin{align}
\boldsymbol{\nabla} \boldsymbol{\times} \mathbf{E} & = -\frac{\partial \mathbf{B}}{\partial t}
\tag{02a}\\
\boldsymbol{\nabla} \boldsymbol{\times} \mathbf{B} & = \mu_{0}\mathbf{j}+\frac{1}{c^{2}}\frac{\partial \mathbf{E}}{\partial t}
\tag{02b}\\
\nabla \boldsymbol{\cdot} \mathbf{E} & = \frac{\rho}{\epsilon_{0}}
\tag{02c}\\
\nabla \boldsymbol{\cdot}\mathbf{B}& = 0
\tag{02d}
\end{align}
with electric charge and electric charge current densities
\begin{align}
\rho(\mathbf{x},t) & = q\cdot \delta\left(\mathbf{x}-\mathbf{x}_{q}\right)
\tag{03a}\\
\mathbf{j}(\mathbf{x},t) & = q\cdot \delta\left(\mathbf{x}-\mathbf{x}_{q}\right)\cdot\dfrac{\mathrm d\mathbf{x}_{q}}{\mathrm d t}
\tag{03b}
\end{align}
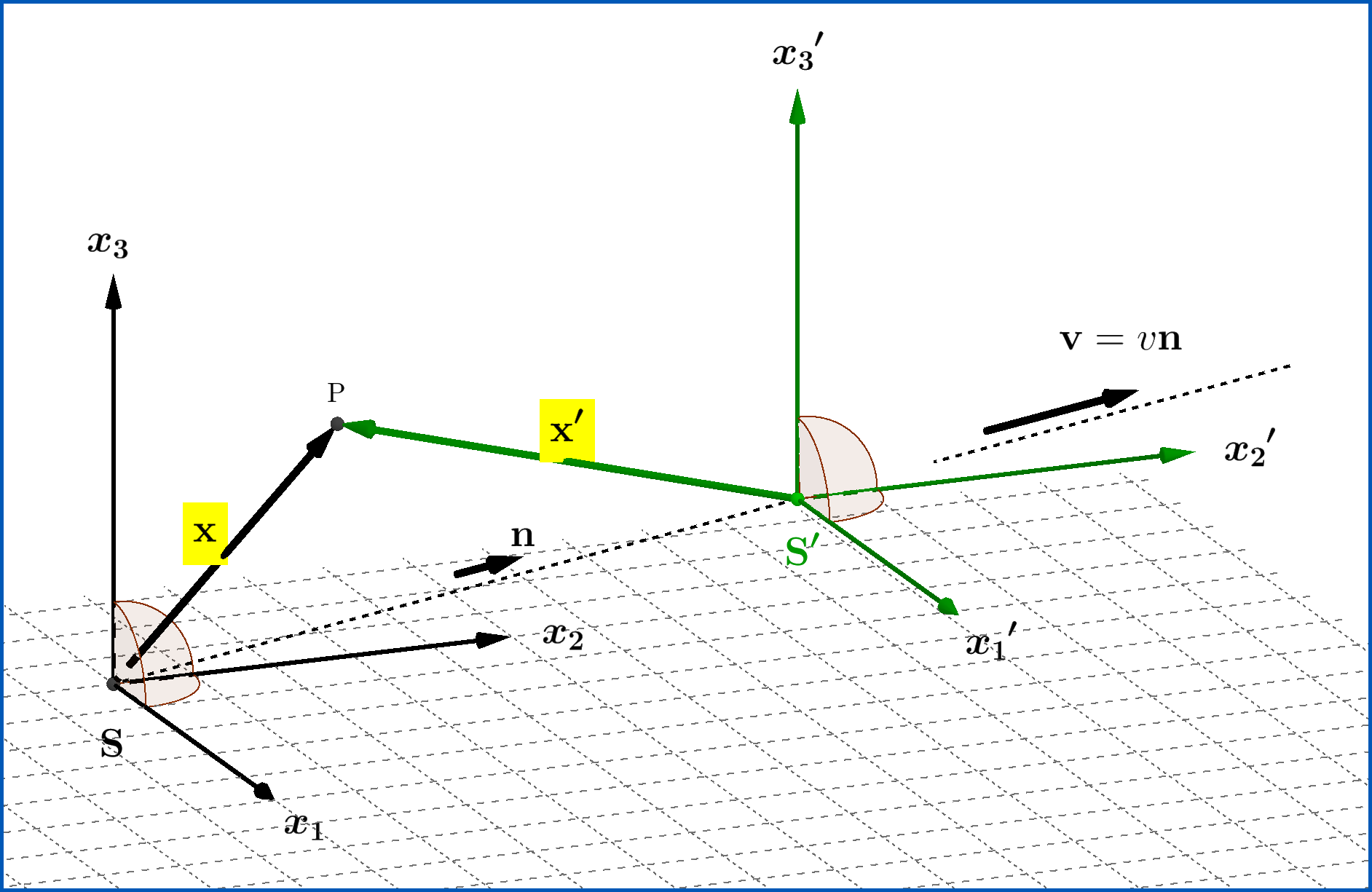
The fact that the electromagnetic field is an entity is more clear if we take a look at its transformation. So let $\:\mathrm{S}\:$ and $\:\mathrm{S}'\:$ two inertial frames of reference and the frame $\:\mathrm{S}'\:$ moves uniformly with velocity $\:\mathbf{v}=\upsilon\mathbf{n}, \upsilon \in \left(-c,+c\right)\:$ with respect to $\:\mathrm{S}$, see Figure in the bottom. Then from $\:\mathrm{S}\:$ to $\:\mathrm{S}'$ \begin{align} \mathbf{E}' & =\gamma \mathbf{E}\!-\!\left(\gamma\!-\!1\right)\left(\mathbf{E}\boldsymbol{\cdot}\mathbf{n}\right)\mathbf{n}+\,\dfrac{\gamma}{c}\left(\mathbf{v}\boldsymbol{\times}c\mathbf{B}\right) \tag{04a}\\ c \mathbf{B}' & = \gamma c\mathbf{B}\!-\!\left(\gamma\!-\!1\right)\left(c\mathbf{B}\boldsymbol{\cdot}\mathbf{n}\right)\mathbf{n}\!-\!\dfrac{\gamma}{c}\left(\mathbf{v}\boldsymbol{\times}\mathbf{E}\right) \tag{04b} \end{align} From equations (04) the six scalar components bind together more strongly in the so-called electromagnetic field tensor \begin{equation} F=F^{\mu\nu} \begin{bmatrix} \hphantom{-}0 & -E_1 & -E_2 & -E_3 \hphantom{-}\vphantom{\dfrac12}\\ \hphantom{-}E_1 & \hphantom{-} 0 & -cB_3 & \hphantom{-} cB_2 \hphantom{-}\vphantom{\dfrac12} \\ \hphantom{-}E_2 & \hphantom{-} cB_3 & \hphantom{-} 0 & -cB_1 \hphantom{-}\vphantom{\dfrac12} \\ \hphantom{-}E_3 & -cB_2 & \hphantom{-} cB_1 & \hphantom{-} 0 \hphantom{-}\vphantom{\dfrac12} \end{bmatrix} \tag{05} \end{equation} transformed under the Lorentz Transformation $\:\Lambda\:$ as follows \begin{equation} F'=\Lambda F \Lambda \tag{06} \end{equation} or by tensors as \begin{equation} F'^{\alpha\beta}=\Lambda^{\alpha}_{\mu} F^{\mu\nu}\Lambda^{\beta}_{\nu} \tag{07} \end{equation}
The magnetic field is not "due to" the changing electric field as in there is a causal effect based on a fundamental physics law. Nor is the magnetic field "due to" the current.
In the frame-of-reference of the electron (i.e., moving along with the electron at exactly its speed, so the electron seems to be at rest from that viewpoint), there is only the static electric field, no moving charge, no changing electric field, no current.
The magnetic field is how an observer from another frame-of-reference (for example the laboratory in which the electron is seen to be moving at speed v) observes the charge together with its electric field due to Einstein's special relativity.
Refer to this video on Youtube: "How Special Relativity Makes Magnets Work"
Announcing the whereabouts of the electron - using the radiating electric field
An interesting illustration of the electric field from an electron is described here: The Electromagnetic field in Space-time (by Ed Lowry).
From the "bullet" idea mentioned there, where the electric field can be illustrated as a series of points or bullets sent off in all directions with regular intervals at the propagation speed of the electric field in the media. In its simplicity it brilliantly displays how the electric field is perceived. It is relativistic.
In reality it is just information traveling in straight lines sent out from a moving object.
Fig 1. The field from a static electron as dots sent off in all directions.
From a static electron an observer will sense a diverging constant electric field.
Fig 2. The field from the electron at constant speed.
If the electron moves past an observer at constant speed, the field will be straight lines but skewed in direction of motion. The deformation or tension of the field now causes a proportional magnetic field. I bet, at a point the time derivative of the varying magnetic field, induces the exact same electric field that created it.
Fig 3. The electron is moved back and forth like in an radio antenna
And finally, if the electron accelerates or oscillates the electric field will display having a wave form and for the first time except when the electron was created energy is not only stored as flux energy, but the energy also moves outwards in the field.
Applied in 3D space and coloring the electric curl, this would look even more spectacular.
Here is a YouTube animation: Electric field simulation from moving electron
