Split moments of inertia into vectors - Right thing to do?

Question

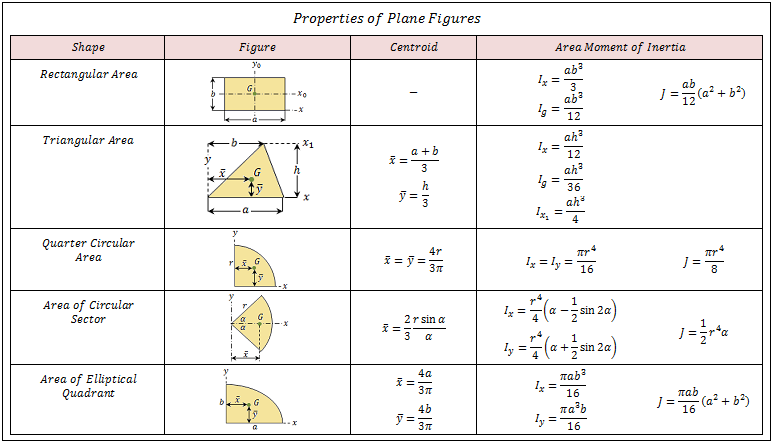
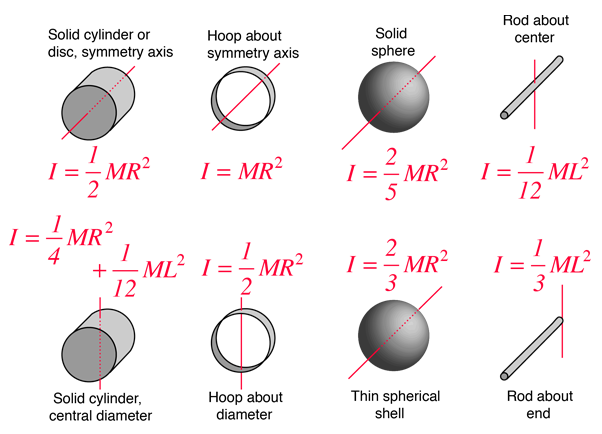Let's say that we have the moment of intertia:

$$J = mR^2$$

And the mass $m$ is on the $(x, y)$ position. Can I say that moment of intertia is then:

$$J = mR^2 = m\sqrt{x^2 + y^2} ^2 = m(x^2 + y^2)$$

The same if I got two masses $m_1, m_2$ and $m_1$ is on position $(x_1, y_1)$ and mass $m_2$ is on the position $(x_2, y_2)$. The moments of intertia are then:

$$J = m_1R_1^2 + m_2R_2^2 = m_1\sqrt{x_1^2 + y_1^2} ^2 + m_2\sqrt{x_2^2 + y_2^2} ^2 = m_1(x_1^2 + y_1^2) + m_2(x_2^2 + y_2^2)$$

Is this true? Or is it true, but it's a very bad way to do that formulation. Especially if the masses $m_1 , m_2$ are on a robotic arm.

Answer 1

This is what you already have with $R^2$, the implication being that it involves a central axis.

If you do what you are doing, your moment of inertia would involve this:

Answer 2

By component

You can add the components of two mass moment of inertia tensors if they are expressed on the same point, and on the same orientation.

So when resolved on the origin each mass $m_i$, located at $\pmatrix{x_i & y_i}$, contributes to the total mass moment of inertia, a $m_i (x_i^2+y_i^2)$ amount.

The total MMOI is

$$ I_{zz} = \sum_i m_i (x_i^2+y_i^2) $$

As a tensor

A a 3×3 tensor each mass $m_i$, located at $\pmatrix{x_i & y_i}$, contributes the following amount

$$ {\rm I} = \sum_i m_i \left[ \matrix{ y_i^2+z_i^2 & -x_i y_i & -x_i z_i \\ -x_i y_i & x_i^2+z^2 & -y_i z_i \\ -x_i z_i & -y_i z_i & x_i^2+y_i^2 } \right] $$