2
$\begingroup$

The Lennard-Jones potential describes long-range attraction and short-range repulsion. In both solids, liquids, and gases there are attractions and repulsions.

  1. Does it mean that Lennard-Jones form of potential is applicable to any phase of a substance?

  2. If yes, does the attractive and repulsive parts of the potential have the same origin in all phases?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
4
$\begingroup$

Let's start by saying that the LJ potential is a very, very crude approximation to a real atomic potential: the $r^{-6}$ term can have some sort of justification because we can say that it comes from dipole-dipole interactions, but the exponent $12$ of the repulsive term is completely made up and has no theoretical justification whatsoever.

But most importantly, the LJ potential is spherically symmetric, so it is only good to approximate the behavior of atoms or spherically symmetric molecules. Thus, it can be a decent approximation for noble gases, but for must substances it is a very poor choice: we just use it because it has a very simple mathematical form.

This said, the answer to your question is that, since the potential describes the interactions between the atoms, of course it will be the same in any phase. Below, you can see the phase diagram of a LJ system (source): as you can see, all three phases are present. This diagram was obtained by means of a computer simulation by varying temperature and pressure, but the interaction potential was left unchanged.

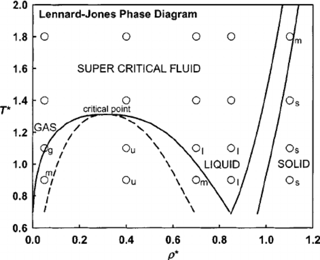
enter image description here

The origin, or better the justification, of the repulsive and attractive part is always the same, like lemon wrote: Pauli exclusion principle for the repulsive part and dipole-dipole (van der Waals) forces for the attractive term. These are an approximation of the interatomic potentials, so, once we take them on trust, there is no reason why they should change during a phase transition.

A phase transition is a macroscopic change in the properties of the system, but the laws governing the microscopic dynamics are always the same.

Improve this answer
$\endgroup$
0
$\begingroup$

Another additional note is that the Lennard-Jones potential allows to model "non-equilibium" phases, or phases that are not shown in the diagram above. For example, in the solid/liquid/gas diagram the simulations are performed for a fixed temperature. But one can generate a Lennard-Jones "glass" by rapidly cooling the system, that is, by rapidly decreasing the reference temperature (as one would do in a glass factory). The properties of this simulated phase seem to explain many observations of glasses (transition point, viscosity, speed of sound, bulk modulus, and so on..).

Improve this answer
$\endgroup$
2
  • $\begingroup$ How do you define the "reference temperature" if the system is out of equilibrium? $\endgroup$
    – Quillo
    Commented Jul 19, 2023 at 7:34
  • 1
    $\begingroup$ The value of the temperature used for the thermostat. This would depend on the algorithm used for the thermostat (Nose-Hoover, Berendsen, and so ..). A system at "equilibiurm" like a liquid or gas would converge to that reference temperature (the mean kinetic energy converges to the reference value). $\endgroup$
    – YoussefMabrouk
    Commented Jul 19, 2023 at 7:37

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.