Condition for book falling off edge of table before it begins slipping

Question

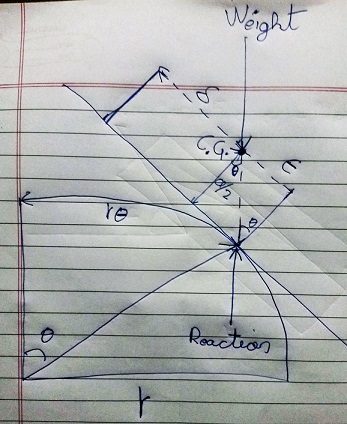
Consider book modeled by a rectangular prism with a square base of length $L$ and a height $e$. The book is placed on a table whose edge is parallel to an side of the rectangular base such that the center of the square base is a small distance $\delta$ from the edge of the table. In other words the overhang off the book is $L/2+\delta$. Call the

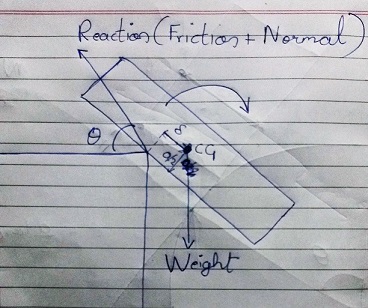
This configuration is unstable - the book will begin rotating about the pivot point. The forces acting on the book are gravity at its center of mass, the normal force at the pivot point which is perpendicular to the surface of the book, and the friction at the pivot point which acts in the radial direction. Note that the normal and friction forces change direction during the rotation.

The coefficient of static friction between the book and the table is $\mu_s$.

A priori depending on the parameters of the problem, the book can rotate about the pivot until the book leaves the surface of the table, or the the book can begin slipping before it leaves the table.

Call the angle which the book forms with the horizontal $\theta$ (so $\theta_0=0$) and define the corresponding polar coordinate unit vectors $\vec{u_r}$ and $\vec{u_\theta}$.

Suppose we have derived, based on the mechanics of the book's rotation (using moment of inertia etc) the expressions for the normal force $N\vec{u_\theta}$ and the friction force $f\vec{u_r}$ in function of $\theta$.

1. What is the condition for the book to fall off the table?

2. What is the condition for the book to begin slipping off the table?

I am not asking at what angle will the book fall off or being slipping etc, I am just asking for the condition so that I can do the calculations myself.

I guess the answers are:

1. $N=0$

2. $|f|=\mu_s|N|$

but I am not sure.

If this is indeed the condition, then I have found that it is impossible for the book to leave the table without first slipping, no matter the parameters of the problem.

Answer 1

I don't have any decent illustrating software so please bear with my hand made drawings. Apologies for the inconvenience

As can be seen in the sharp corner case, there is always a non-zero moment created by the weight and net reaction force(I have taken the height of the book to be $a$ so it's CG is at $a/2$):

for this moment to be cancelled out, both the weight and reaction force should coincide at the CG. Also they should be equal and opposite for no net acceleration. This second case is possible if the table's edge has a finite radius. It is illustrated in this picture:

(note that I haven't drawn the full book here, only the relevant part). If you wish you can solve it yourself from here but I'll post the rest of the solution anyway for the benefit of others. We can decompose the Reaction force $R$ into the normal and tangential directions to the table. \begin{align*} \text{normal component:} &\; R \cos\theta \\ \text{tangential component:} &\; R \sin\theta \end{align*} Since the tangential component is nothing but the frictional force, it should be equal to $\mu_s$ times the normal force: \begin{align*} R \sin\theta &= \mu_s R \cos\theta \\ \mu_s &= \tan\theta \tag{1} \end{align*}

This is the same familiar condition as it would be on an inclined plane. But the line of forces also have to pass through the CG. For this observe that the length $\delta + \epsilon$ is equal to the arc length of the amount that it has rolled $r\theta$. $$\delta + \epsilon=r\theta$$ also observe from the geometry that $\epsilon= \frac a2 \tan\theta$. Therefore $$\delta + \frac a2 \tan\theta=r\theta \tag{2}$$ substituting eqn $(1)$ in $(2)$ we get:

$$\delta + \frac a2 \mu_s=r \tan^{-1}\mu_s\\ r=\frac{\delta + \frac a2 \mu_s}{\tan^{-1}\mu_s}$$

If $r$ is smaller than the above value then the book will fall off the table. In this case,the book will roll up till the angle of repose (given by $\tan^{-1}\mu_s$) and after that the book will simultaneously slip and rotate off the table.