Physical significance of the terms of acceleration in polar coordinates

Question

How do I get an idea, or a 'feel' of the components of the acceleration in polar coordinates which constitute the component in the eθ direction?

from what I know, $\vec a= (\ddot{r}−r\dot{θ}^2) \hat e_r + (r\ddot{θ}+ 2\dot{r}\dot{θ}) \hat e_θ$, where $\hat e_r$ and $\hat e_θ$ are unit vectors in the radial direction and the direction of increase of the polar angle, θ.)

The two components in $\hat e_r$ direction - $\ddot{r}$ and $r\dot{θ}^2$ - are the usual acceleration along radius vector and the centrifugal force experienced. But what is the significance of the other two terms?. Is there any day-to-day or a common situation where we experience the Coriolis force and the other term?

I can memorize the formula and use it, but I will truly 'understand' its significance only if I can 'feel' the terms.

Answer 1

$\ddot{r} \hat e_r$: usual radial acceleration

$-r\dot{θ}^2 \hat e_r$: centripetal acceleration

$r\ddot{θ}\hat e_θ$: This is the Euler acceleration. It is an acceleration due to a change of angular velocity (at fixed $r$). Example taken from the linked wikipedia article: on a merry-go-round this is the force that pushes you to the back of the horse when the ride starts (angular velocity increasing) and to the front of the horse when the ride stops (angular velocity decreasing).

$2\dot{r}\dot{θ} \hat e_θ$: Coriolis acceleration

Answer 2

I can only answer part of your query: the Coriolis force does not enter into this. The form you have comes directly from expressing $\ddot{x}$ and $\ddot{y}$ in terms of derivatives aof $r$ and $\theta$ through the geometrical change \begin{align} x(t)&=r(t)\cos(\theta(t))\, ,\qquad y(t)=r(t)\sin(\theta(t)) \end{align} followed by converting $$ \hat x=\cos(\theta(t))\hat r- \sin(\theta(t))\hat \theta\, ,\quad \hat y=\sin(\theta(t))\hat r+ \cos(\theta(t))\hat \theta\, . $$ Thus the various bits and pieces in polar coordinates come purely from the change of coordinate system, not the inertial or non-inertial nature of the reference frame, in which you can set whatever coordinate systems you want.

Because the orientation of $\hat r$ and $\hat \theta$ depend on the position in space, it is not easy to get intuition into their components.

Answer 3

Here is a full explanation. I am changing the notation $\hat{e_r} \to \hat{r}$, $\hat{e_\theta} \to \hat{\theta}$.

We know that $\textbf{R} = r \hat{r}$. Hence, $\textbf{V} = \dot{r} \hat{r} + r \dot{\theta} \hat{\theta}$. Let us also denote by $\textbf{V}_r = \dot{r} \hat{r} (= \textbf{V} \cdot \hat{r} \hat{r})$, and $\textbf{V}_\theta = r\dot{\theta} \hat{\theta} (= \textbf{V} \cdot \hat{\theta} \hat{\theta})$. It is clear (I hope so) why is that- the change of $\textbf{R}$ is attributed to the change along $\hat{r}$ (which is \dot{r}), and by the change of the direction of $\hat{r}$, which is $r\dot{\theta}$.

Deriving this, we see that $\textbf{a} = (\ddot{r} - r \dot{\theta}^2) \hat{r} + (r\ddot{\theta} + 2 \dot{r} \dot{\theta}^2) \hat{\theta}$.

Now for the acceleration, Let us split it into the change of $\textbf{V}_r$ and of $\textbf{V}_\theta$ (and then the change of $\textbf{V}$ will be their sum, and it is the acceleration).

Let us look first at $\textbf{V}_r$:

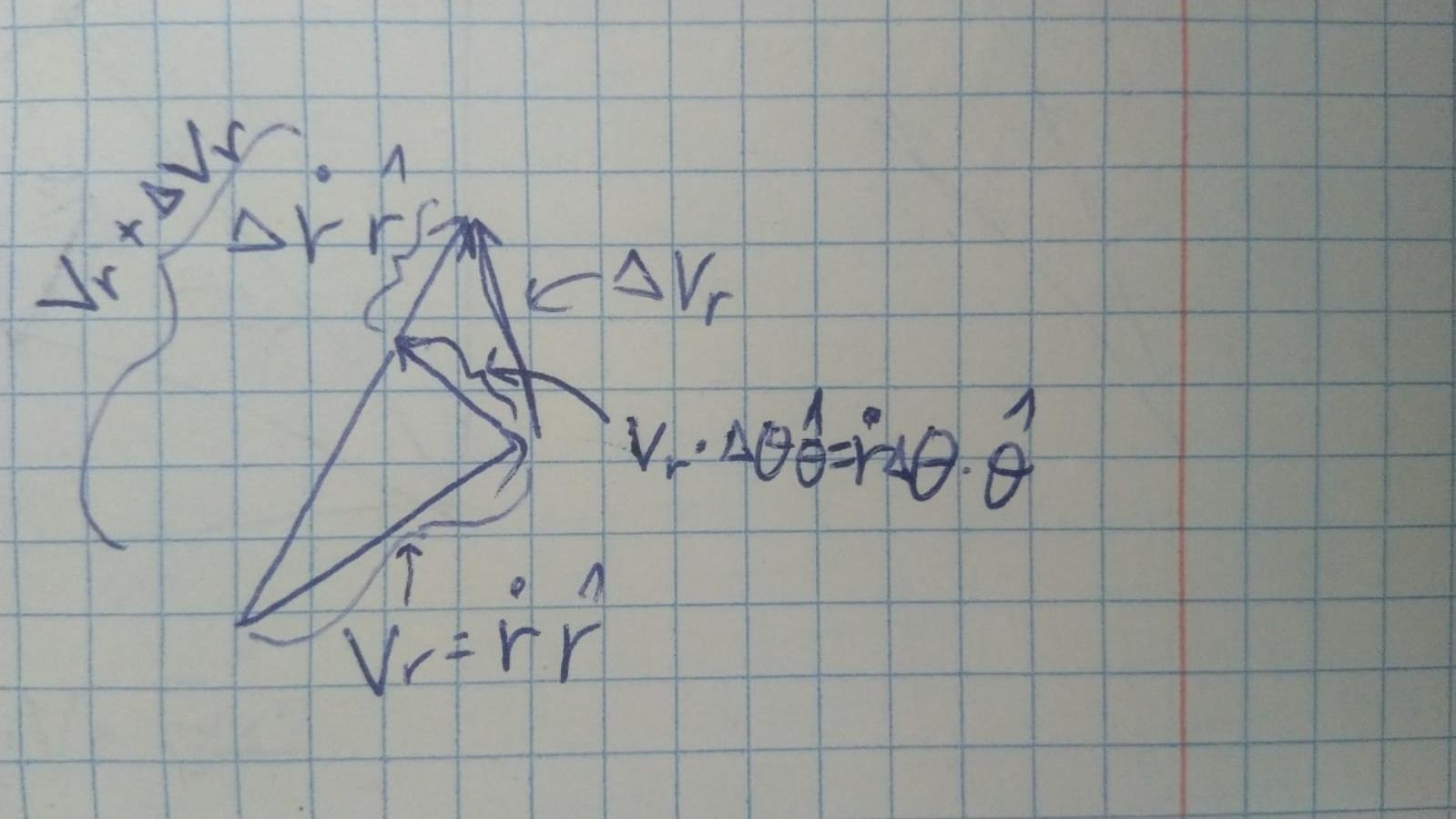
We can see that $\Delta \textbf{V}_r$ is the vector sum of the change along $\hat{r}$ direction which is $\Delta \dot{r}$, and the change caused by the change of direction of $\hat{r}$, which is in the direction of $\hat{\theta}$, with a length which is approximately the length $|\textbf{V}_r| \cdot \Delta \theta = \dot{r} \Delta \theta$ of the arc in $\hat{\theta}$'s direction. Overall we get $\Delta \textbf{V}_r = \Delta \dot{r} \hat{r} + \dot{r} \Delta \theta \hat{\theta}$ with $\Delta t$, $\Delta \theta$ infinitely small, therefore with dividing by $\Delta t$, in the limit we get $\dot{\textbf{V}_r} = \ddot{r} \hat{r} + \dot{r} \dot{\theta} \hat{\theta}$.

Now for the more interesting $\Delta \textbf{V}_\theta$:

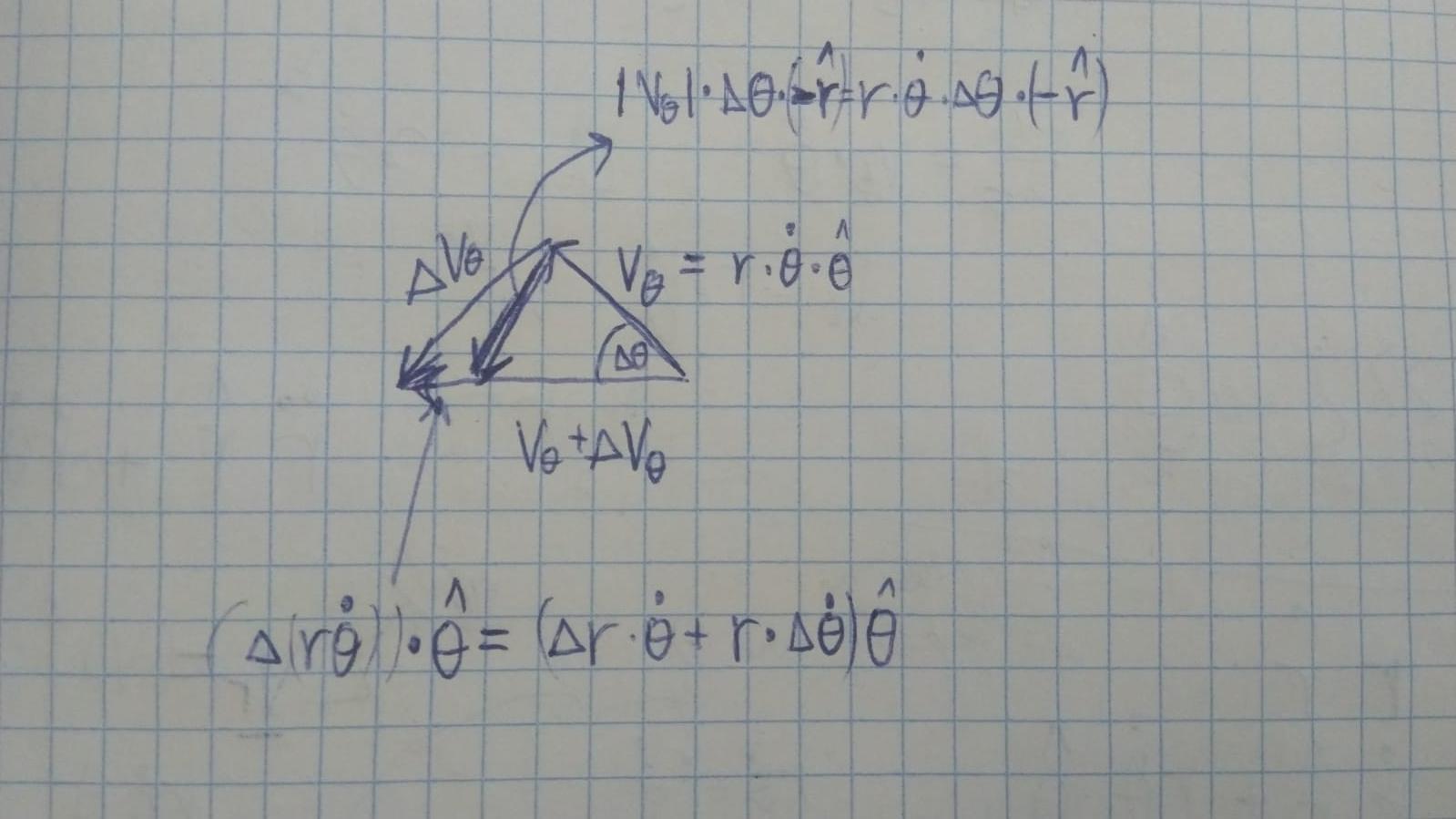
We can see that $\Delta \textbf{V}_\theta$ is the vector sum of the change caused by the change of direction of $\hat{\theta}$, and the change along $\hat{\theta}$. The change caused by the change of direction of $\hat{\theta}$ is the approximately an arc in the direction of $-\hat{r}$ in with length $|\textbf{V}_\theta| \cdot \Delta \theta = r \cdot{\theta} \Delta \theta \cdot (- \hat{r})$.

The change along $\hat{\theta}$ is of length $\Delta (r \dot{\theta}) = \Delta r \dot{\theta} + r \Delta \dot{\theta}$, which can be explained as the cange caused by the increase/decrease in the angular velocity, summed with the change caused when we increase/decrease the length $r$, which causes the velocity along the arc (which is of length $r \dot{\theta}$ to increase/decrease to be $(r + \Delta r) \dot{\theta}$. With deviding by $\Delta t$ and looking at the limit, we get $\dot{\textbf{V}_\theta} = - r \dot{\theta}^2 \hat{r} + \dot{r} \dot{\theta} \hat{\theta} + r \ddot{\theta} \hat{\theta}$.

Overall we get the equation we needed. What is interesting is that the term $2 \dot{r} \dot{\theta} \hat{\theta}$ is splitted to two halves, one comes from the change in $\textbf{V}_r$ and the other from the change in $\textbf{V}_\theta$.

I hope that now it is more clear.

As a piece of general advice, deriving yourself the equations, multiplying by the $dt$ and trying the look at the $d(\text{stuff})$ geometrically is helpful sometimes.