Minkowski diagrams: when to project parallel to which axes

Question

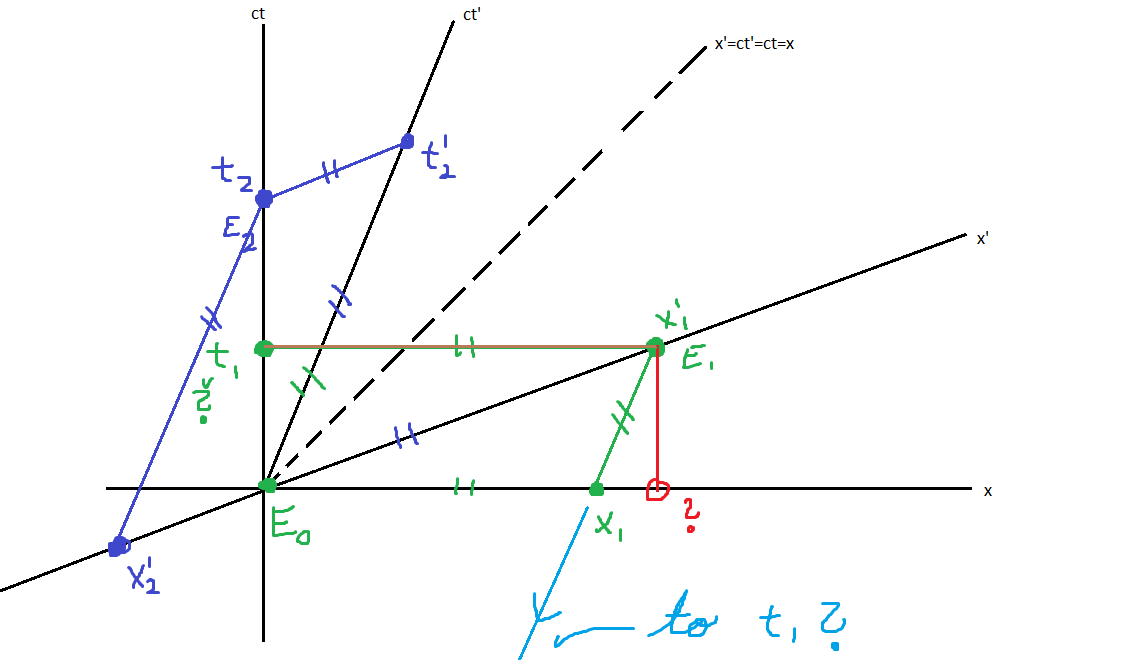
Before I start, I promise this is not a duplicate. I've read through all of the answered related questions, and none of them have quite the insight I'm looking for. Below I've attached a spacetime diagram with a couple of events labeled, as well as what I'm guessing to be their Lorentz transformed projections in the other frame. I take the prime frame to be the moving one, and the unprimed to be at rest.

So far, I've been solving problems just fine using these diagrams, paired with the explicit calculations, but I've seem to hit an intuitive wall when I started asking some odd questions.

My first questions is regarding $ E_1 $. This event happens at t'=0, but at a non zero x', and if $ c t=\gamma(c t'+\beta x') $, then naturally we have $ ct \neq 0 $. I'm however, unsure as to what the coordinates of this event will be in R. I think I have the spatial coordinate fine just from projecting parallel to the $ct'$ axis, and seeing where that line cuts the $x$ axis. I'm not clear on what the time coordinate should be either. My intuition based on what I did with the last coordinate, tells me to draw a line through $E_1$ parallel to the $x'$ axis, but that leads me to $t=0$, which is false. The blue line guess for a projection leads me to a negative value, contrary to what I should see based on the mathematics, and this leaves me with the brown line, which is parallel to the $x$ axis.

My confusion arises from the fact that, for events occurring in the same place in R, transforming the coordinates involved projecting parallel to $ct'$ and $x'$. I would think that to go from R' to R would then have to project parallel to the axes of R instead, but this doesn't seem to work when doing other problems.

I guess my main question is, when do I project parallel to the primed axes, and when do I project parallel to the unprimed axes? What would that red projection line correspond to? I though I had this understood and have been solving problems, but alas, I'm stumped now that I've got this on my mind.

Answer 1

I think you actually did it correctly without knowing which one is which one. (I will omit the factor c for simplicity)

Just for clarification: R denotes the unprimed coordinate system, R' the primed one. First, I will explain the technique generally before using your specific coordinates.

Reading the coordinates in R

The coordinates of $$E_1 = (t_1, x_1)$$ can be read of from the diagram just as in any other cartesian coordinate system. To read off the time component you can just go to the left, parallel to the $x$-axis until you cross the $t$-axis. This corresponds to the brown line you have drawn in your diagram.

To read off the spatial component $x_1$ you can go down, parallel to the $t$-axis until you cross the $x$-axis. The crossing point is your coordinate $x_1$, the red line projection.

Reading the coordinates in R'

To read off the coordinates in the primed system, $$E_1' = (t_1', x_1'),$$ the technique is almost the same, but since your axis are not horizontal and vertical anymore you can not just go to the left resp. go down anymore.

To read off the spatial component $x_1'$ you draw a line going through the event $E_1$ which is parallel to the $t'$-axis. The time component $t'$ can be read off by drawing a line parallel to the $x'$ axis.

Roughly speaking the most important thing is, when you read off the coordinates in one system you don't care about the axis in the other system.

Applied to the example

So the event $E_1$ in the primed system $R'$ is $$E_1' = (0, x_1')$$ As you mentioned the transformation to the system $R$ is given by $$ E_1= (\beta \gamma x_1', \gamma x_1') $$ The time component in $R$ is $t_1= \beta \gamma x_1'$. This is the coordinate you read off by following the brown line. The spatial component is $x_1= \gamma x_1'$. This corresponds to the crossing of the $x$-axis with the red line.

For the second event we want to describe the event in the system $R$ first and then read off its coordinates in the system $R'$. The event in the unprimed system is $$ E_2 = (t_2, 0).$$ Since we are transforming now in the other direction, meaning $R \to R'$ instead of $R' \to R$, the Lorentz transformation has a negative sign for the velocity $\beta$. $$ E_2' = (\gamma t_2, -\beta \gamma t_2) = (x_2', t_2') $$ Now the read off is done with parallel lines to the axis $x'$ resp. $t'$. This corresponds to the blue lines you have drawn. You can directly see the negative sign of $x_2'$ at the crossing of the left blue line with the $x'$-axis.

The Green projections

Suppose you have an event at the green $x_1$. This not the $x_1$ of the event $E_1$. So let's say you have an event at this point expressed in system $R$, $$E_3= (x_3, 0), $$ where $x_3$ is at the point you marked as $x_1$ in your diagram. Then, to read off the spatial component in the system $R'$ you can use the green projection, parallel to t'. Mind that $x_3'$ will be equal to the spatial component of $E_1'$, $$x_3'= x_1'$$ but the time component is different. You can find it by drawing a line parallel to the $x'$-axis, going through the event. Then you will see that it crosses the time axis at a negative time component, not at $t'=0$.

Answer 2

The event is a point in spacetime; then you can add a frame of references. In order to find the coordinates of an event, you have to construct a 'grid' of coordinates for a given frame of reference. This grid is constructed considering any point of an axis and drawing lines parallel to the other axis. This physically correspond to put other observers moving with the same velocity (this gives the grid parallel to $t$, ie constant $x$) and then taking lines of constant proper time of these observers (grid parallel to $x$, ie constant $t$).

Therefore, given a point in spacetime, if you want to find its coordinates in the $R$ frame, you just draw the usual cartesian grid:

And for $R'$ you draw the grid parallel to its axes:

Putting things altogether: