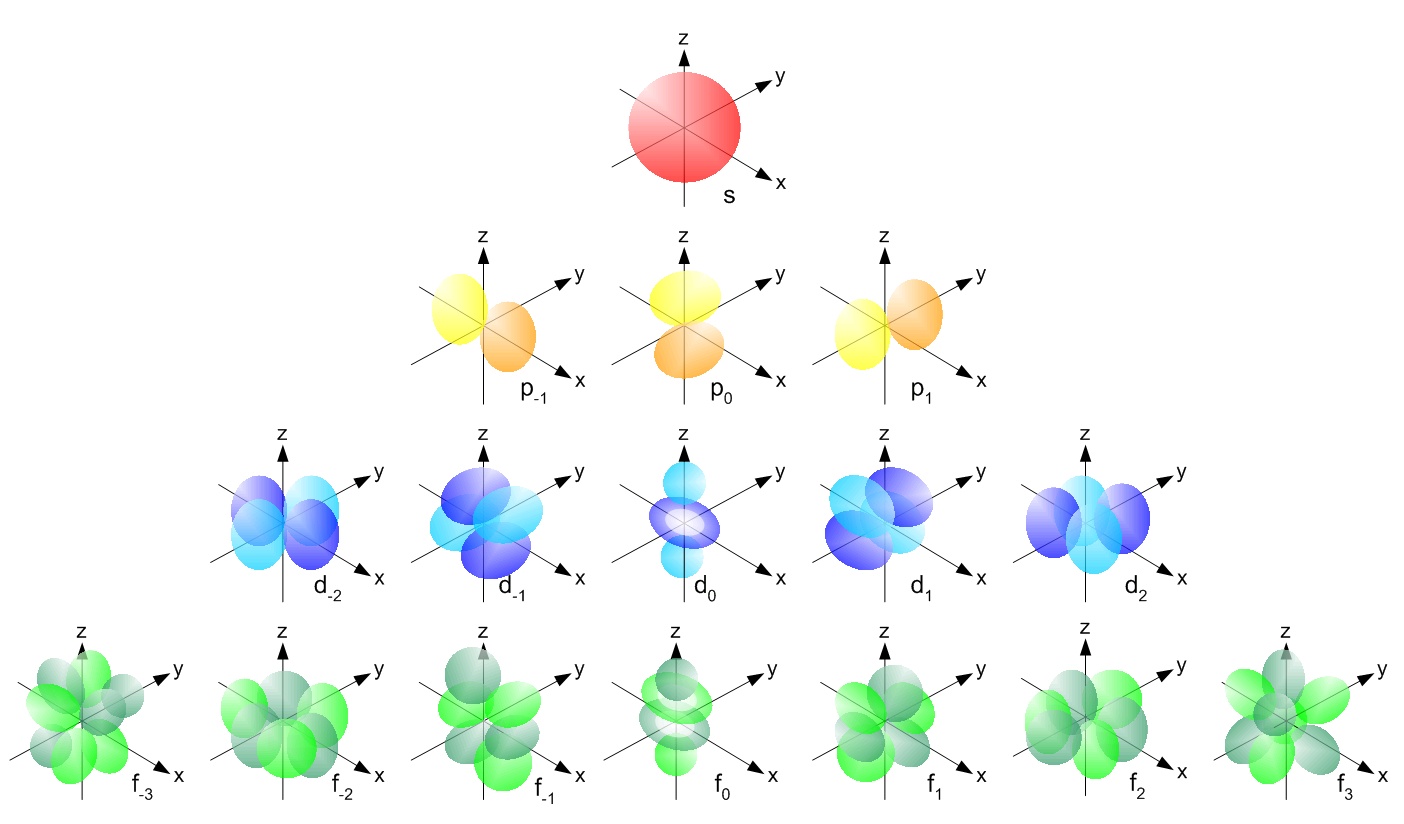
Atoms can be aligned in magnets to create magnetic fields. Does that alignment give an atom a north and south pole or certain atoms have a unique electron orbitals giving an atom a north and south pole? Do electrons orbit in their orbitals in a particular direction in those types of atoms when aligned? This answer gives a good example on electron orbitals around/near an atom and would these orbitals change with alignment?
$\begingroup$
$\endgroup$
4
-
4$\begingroup$ Electrons do not orbit atoms in the classical sense, see e.g. physics.stackexchange.com/q/20003/50583, physics.stackexchange.com/q/137207/50583, physics.stackexchange.com/q/9415/50583. $\endgroup$– ACuriousMind ♦Commented Jan 31, 2017 at 17:14
-
$\begingroup$ I know this but does any of these orbits change with magnetic material? $\endgroup$– MuzeCommented Jan 31, 2017 at 17:36
-
$\begingroup$ 1. What purpose does including the pictures in the question serve? 2. If you know this, might you consider changing the title and the question to reflect that? 3. It appears to me that you're trying to ask for the microscopic origin of ferromagnetism, but it's unclear why you might have the impression that the orbitals (not orbits!) change. See physics.stackexchange.com/q/95909/50583, physics.stackexchange.com/q/39299/50583 for discussions of magnetism in atoms. $\endgroup$– ACuriousMind ♦Commented Jan 31, 2017 at 17:46
-
1$\begingroup$ Jen, please read this article en.wikipedia.org/wiki/Electron_configuration. Because the electrons are like little magnets, then their orbitals (which are the places they are most likely be found and not the paths they follow, as they don't follow paths), can be changed by strong electromagnetic fields. $\endgroup$– user140606Commented Jan 31, 2017 at 19:01
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
3
Please remember that orbitals are the region's where electrons are most likely to be found, but the electron does not have a path, just a chance, a very high chance, of being found in the region.
Say one second, we measure where the electron is, and we find it in a region. Then two minutes later we look again, and we find it somewhere else, but where it goes between the times we look, we don't know.
The electron has to follow certain rules when it is part of an atom and these are:
- The electron has two states it can be in, call them magnetic up and magnetic down, (again, up and down are just to say they are different, they are not literally up or down, unless we set a coordinate system that references them that way). Thank you to Ruslan for pointing this out
I include a picture, which hopefully illustrates this.
Image Source: Electron Spin
In each orbital, only two electrons can fit in the orbital, one up and one down.
The atom has discrete energy levels around it, so the electron has to fit into a definite energy level, like a car that can only go at 20 and 40 mph, it cannot travel at 30 mph. That's very odd, but that the way quantum physics works.
The electrons all have a negative charge, so they want to stay as far apart from each other as possible, but the positive charge of the nucleus keeps them from drifting away.
Nearest the nucleus, there is a spherical shaped orbital and two electrons, an up and a down, can fit in. This is the ground state, with the lowest energy level.
At the next energy level, we can fit in 4 electrons, but they have to stay as far as apart as possible, so if you look at the diagram below, you understand why the orbitals are the funny shapes they are.
-
1$\begingroup$ Spin up/down are literally up and down: this naming assumes that you have your chosen $z$ axis directed up. $\endgroup$– RuslanCommented Jan 31, 2017 at 20:22
-
1$\begingroup$ @Ruslan thanks very much for that, the other answer(s) I think may be a bit "mathy" for the OP. I have edited in a picture, but I think the OP has a basic mental picture of the electron, that I don't want to reinforce. I want to be correct, so I do take your point. $\endgroup$– user140606Commented Jan 31, 2017 at 20:39
-
1
$\begingroup$
$\endgroup$
3
It is not quite right to think of the electron orbiting the nucleus, but rather than its wave function has angular momentum. The electron in the hydrogen atom has a wave function $\Psi(r,\theta,\phi~=~R(r)\Theta(\theta)\Phi(\phi)$ for the radial angular and azimuthal parts. The angular part has a solution according to Legedre polynomials with eigenvalues of the angular momentum squared $$ L^2|\ell,m\rangle~=~[\ell(\ell~+~1)~+~m^2]|\ell,m\rangle $$ where $m$ is the projection of the angular momentum along the $z$-axis. The $z$ axis is the direction of some "probe field," such as the magnetic field. One then has the interaction Hamiltonian $\hat L\cdot\vec B$. This will have values in $m~=~-\ell,-\ell+1,~\dots,\ell-1,\ell$. The lower value is lower in energy.
answered Jan 31, 2017 at 18:20
-
$\begingroup$ Can some orbitals be back/forth in a strait line, some on a con curve path and others on an infinity8 path ? $\endgroup$– MuzeCommented Jan 31, 2017 at 18:37
-
-
1$\begingroup$ There is not a path for the electron. In the treatment with path integrals there is a whole set of paths. With the image above the rotation is really a phase. It is not a direct reflection of the orbit of an electron in the sense of a classical particle. $\endgroup$ Commented Feb 1, 2017 at 1:03