I've been trying to fully understand energy density in terms of the equations that explain it. Unfortunately, the internet hasn't been very helpful in clarifying my misunderstanding. One website defines energy density(u) as energy(E) over volume(V):
u=E/V
I also noticed that pressure(P) can be defined in the same way:
P=E/V
This leads me to believe that they must be describing the same thing. However, I doubt my sources and my own basic knowledge of physics.
From a statistical mechanics point of view, the energy density is really defined as:
$$ u=\frac{E}{v} $$
The pressure however is the conjugate variable of the volume, thus:
$$ P=\frac{\partial E}{\partial v} $$
The two are the same only when the energy is linear in the volume. This indeed may depend on the momentum as written above for some systems, But may also depend on other things. Think for example what happens when a piston is compressing gas.
Yeah, where is the definition $ P=\frac{E}{V}$ from? It most definitely does not hold for all systems.
There are systems for which $ P=\frac{2E}{3V}$ (example: ideal classical gas) and systems for which $ P=\frac{E}{3V}$ (example: photon gas) and, generalizing these cases, systems for which $ P=\frac{sE}{3V}$ where the relation between energy and momentum is $E\propto p^{s}$ (independent of whether boson or fermions are in discussion).
So yes, they are closely related but they most definitely aren't one and the same thing.
There s also another aspect to energy density, that was not mentioned in previous answers. From "chemical" point of view you can say that for example gasoline has larger energy density that water (energy is "stored" in bonds between atoms and can be released by breaking some of these bonds).
I see why you are confused, especially based on your comment where you shared this link.
Just because the units are the same, it does not mean that they describe the same thing. Pressure can be rearranged to have the same units as energy density; but it doesn't represent the whole energy density of the system. It contributes, but there are many other factors which can add internal energy density without changing pressure.
The link you showed also says some other things that are complete oversimplifications to the point of being untrue.
If you are peeling an apple, then pressure is the key variable
That's definitely not true, for example. They are using pressure in an extremely generic way whenever the units work, when often it would be considered more as a distributed force (or in their really bad example, internal energy).
I came to this same question when thinking about the ideal gas equation, $PV= NkT$. If temperature is a kind of average kinetic energy per gas molecule, then the right side of the ideal gas equation is essentially number of molecules times average kinetic energy per molecule, which is the total kinetic energy in your system of gas. If one side of the equation represents total kinetic energy, it would make sense for the other side also to represent total kinetic energy, and the units of $PV$ bear that out. If you divide both sides by volume $V$, your left with $P = NkT/V$. So, not getting too concerned about $k$, which is a conversion factor between degree K energy units and Joule energy units, for an ideal gas you really can view pressure as kinetic energy density - average kinetic energy per unit volume, instead of average kinetic energy per molecule. The ideal gas equation seems to relate these two basies for considering how energy is distributed.
Gwhiz wrote:
"I came to this same question when thinking about the ideal gas equation, PV=NkT. If temperature is a kind of average kinetic energy per gas molecule, then the right side of the ideal gas equation is essentially number of molecules times average kinetic energy per molecule, which is the total kinetic energy in your system of gas. If one side of the equation represents total kinetic energy, it would make sense for the other side also to represent total kinetic energy, and the units of bear that out. If you divide both sides by volume, your left with P=NkT/V."
Indeed, there is a very close association with pressure and temperature, temperature and energy density, and thus pressure and energy density.
In statistical mechanics the following molecular equation is derived from first principles: P = n k_B T for a given volume.
Therefore T = (P / (n k_B)) for a given volume.
Where:
k_B = Boltzmann Constant (1.380649e−23 J·K−1);
T = absolute temperature (K);
P = absolute pressure (Pa);
n = number of particles
If n = 1, then T = P / k_B in units of K / m³ for a given volume.
Now, some may protest "Temperature does not have units of K / m³ !!!"... note the 'for a given volume' blurb. We will cancel volume in a bit.
We can relate velocity to kinetic energy via the equation:
v = √(v_x² + v_y² + v_z²) = √((DOF k_B T) / m) = √(2 KE / m)
As particle velocity increases, kinetic energy increases. As kinetic energy increases, kinetic energy over volume increases. As kinetic energy over volume increases, energy density increases.
Kinetic theory gives the static pressure P for an ideal gas as: P = ((1 / 3) (n / V)) m v² = (n k_B T) / V
Combining the above with the ideal gas law gives: (1 / 3)(m v²) = k_B T
∴ T = mv² / 3 k_B for 3 DOF
∴ T = 2 KE / k_B for 1 DOF
∴ T = 2 KE / DOF k_B
See what I did there? I equated kinetic energy to pressure over that volume, thus canceling that volume, then solved for T.
A fluid moving with fewer than 3 DOF has a higher temperature (for the same kinetic energy) than a fluid with 3 DOF. This is especially relevant in, for instance, high-pressure system pressure relief piping, where the stagnation temperature can be much higher than the static temperature.
You will note that the above aligns with the Bernoulli Principle.
This is how Sandia National Laboratories calculates temperature.
Based on your comment, I think what you are getting at is that if there is displacement $\delta x$ under pressure $p$ exerted over area $A$, such that reduction in systems's volume $A~\delta x$ takes place, then \begin{align} P=\frac{F}{A}=\frac{F~\delta x}{A~\delta x}=\frac{\textrm{Displacement work done}}{\textrm{Change in volume}} \end{align} However energy density is \begin{align} E=\frac{\textrm{Energy content}}{\textrm{Current total volume}} \end{align} There is no resemblance between the two.
When all else fails, do dimensional analysis.
Force: [M1 L1 T-2] /
Area: [M0 L2 T0] =
Pressure: [M1 L-1 T-2] /
Length: [M0 L1 T0] =
Pressure Gradient: [M1 L-2 T-2]
Energy: [M1 L2 T−2] /
Volume: [M0 L3 T0] =
Energy Density: [M1 L-1 T-2] /
Length: [M0 L1 T0] =
Energy Density Gradient: [M1 L-2 T-2]
In point of fact, the highest pressure ever attained by humankind at the time was done by increasing energy density via lasers in fusion experiments.
Yes, pressure and energy density are two forms of the same thing, just as pressure gradient and energy density gradient are two forms of the same thing.
Thus, just as, for instance, water only spontaneously flows down a pressure gradient (ie: downhill), energy only spontaneously flows down an energy density gradient. This is reflected in 2LoT in the Clausius Statement sense.
[EDIT]
https://i.imgur.com/QErszYW.gif
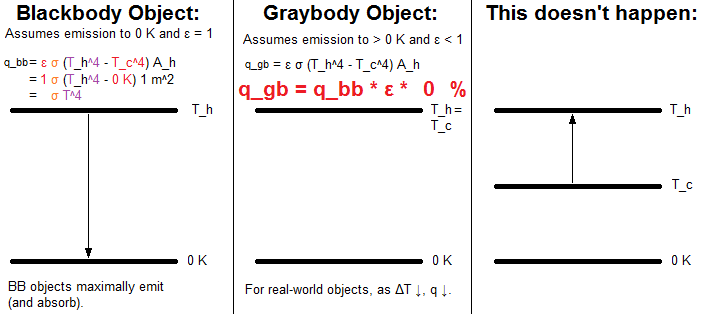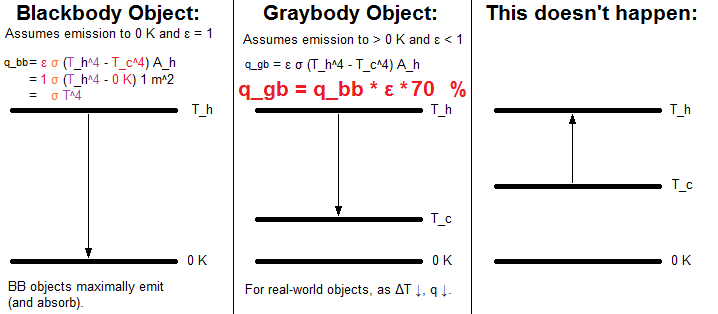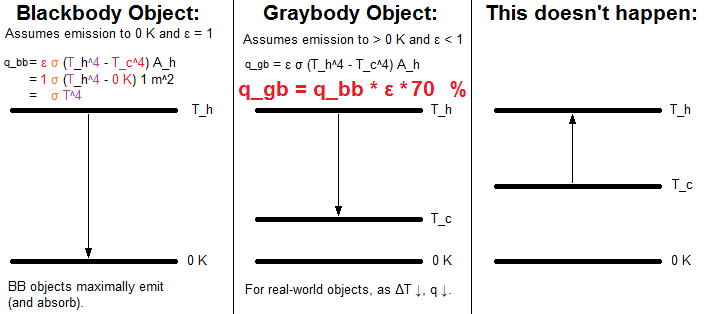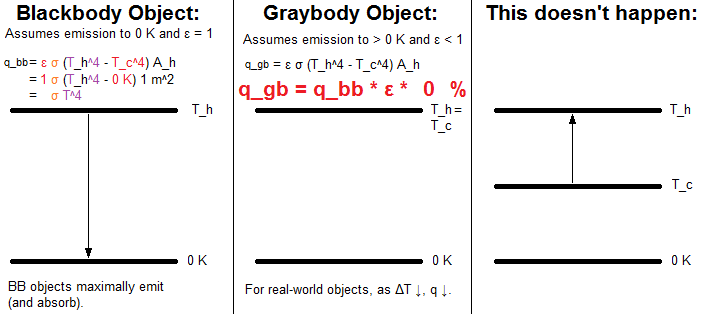
Idealized Blackbody Object (assumes emission to 0 K and ε = 1 by definition):
q_bb = ε σ (T_h^4 - T_c^4) A_h
= 1 σ (T_h^4 - 0 K) 1 m^2
= σ T^4
Graybody Object (assumes emission to > 0 K and ε < 1):
q_gb = ε σ (T_h^4 - T_c^4) A_h
The 'A_h' term is merely a multiplier, used if one is calculating for an area larger than unity [for instance: >1 m^2], which converts the result from radiant exitance (W m-2, radiant flux per unit area) to radiant flux (W).
Temperature is equal to the fourth root of radiation energy density divided by Stefan's Constant (ie: the radiation constant).
e = T^4 a
a = 4σ/c
e = T^4 4σ/c
T^4 = e/(4σ/c)
T = 4^√(e/(4σ/c))
T = 4^√(e/a)
q = ε σ (T_h^4 – T_c^4)
∴ q = ε σ ((e_h / (4σ / c)) – (e_c / (4σ / c))) Ah
Canceling units, we get J sec-1 m-2, which is W m-2 (1 J sec-1 = 1 W).
W m-2 = W m-2 K-4 * (Δ(J m-3 / (W m-2 K-4 / m sec-1)))
∴ q = (ε c (e_h - e_c)) / 4
Canceling units, we get J sec-1 m-2, which is W m-2 (1 J sec-1 = 1 W).
W m-2 = (m sec-1 (ΔJ m-3)) / 4
One can see from the immediately-above equation that the Stefan-Boltzmann (S-B) equation is all about subtracting the radiation energy density of the cooler object from the radiation energy density of the warmer object.
∴ q = σ / a * Δe
Canceling units, we get W m-2.
W m-2 = (W m-2 K-4 / J m-3 K-4) * ΔJ m-3
For graybody objects, it is the radiation energy density differential between warmer object and cooler object which determines warmer object radiant exitance.
Do keep in mind that a warmer object will have higher energy density at all wavelengths than a cooler object:
https://i.sstatic.net/qPJ94.png
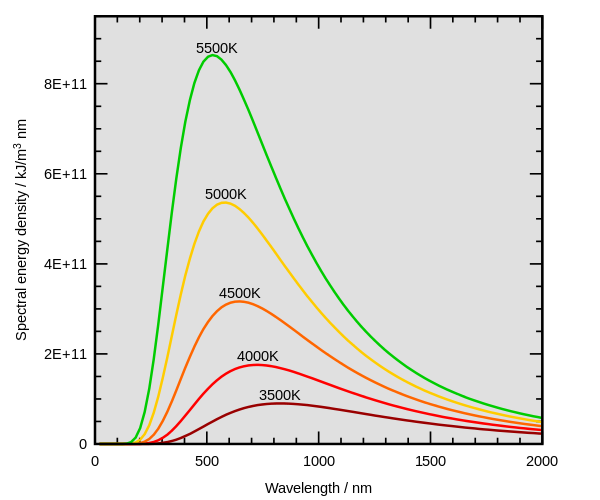
Warmer objects don't absorb radiation from cooler objects (a violation of 2LoT in the Clausius Statement sense and Stefan's Law); the lower radiation energy density gradient between warmer and cooler objects (as compared to between warmer object and 0 K) lowers radiant exitance of the warmer object (as compared to its radiant exitance if it were emitting to 0 K). The radiation energy density differential between objects manifests a radiation energy density gradient, each surface's radiation energy density manifesting a proportional radiation pressure.