This question is already filled with excellent answers. I have only thought of complimenting what the others have said with a bit of a historic and "human" perspective and train of thought. So to answer the question of exactly how spacetime curvature generates the effect of gravity, I will first take a small detour to the state of classical mechanics at the end of the XIX century.
Newton's laws of motion and the birth of Calculus have been pushing forward physics for over a century. Powerful elegant formulations have been invented, and the century of lights has even yielded the blossom of classical electromagnetism and classical thermodynamics. Even so, at the foundations of classical mechanics there are still some incongruencies, which have namely to do with the equivalence of masses problem, and the relativity problem, which are intimately related, as General Relativity would eventually show.
- Mass equivalence
Have you learned Newton's laws of motion like this?
$$F=m\,a \hspace{1cm} F=-\frac{GMm}{r^2}$$
And have you perhaps at a physics lesson used it, by equating the two, to figure out the acceleration of a free-falling body at the surface of the earth? Well, this wasn't always that trivial. Newton's laws were actually "written"(he actually didn't write formulas, it wasn't a habit at the time) like this:
$$F=m_i a \hspace{1cm} F=-\frac{GMm_g}{r^2}$$
Where $m_i,m_g$ denote the inertial and gravitational mass. This might seem like a superfluous difference, but it absolutely is not. If you actually read the Principia (Newton's famous book on his mechanics) you will encounter a bunch of crazy definitions of up to three kinds of force and two kinds of mass. This was because gravity and other forces were conceived as being different kinds of phenomena. So inertial mass is the ability of a body to resist for example a push, and gravitational mass is the ability to being gravitating towards a massive body. Now here's the crazy thing that no one could explain. They were actually numerically the same. We knew that from Galileo's experiments on inclined planes and other experiments on pendulums; that these two very different kinds of masses, associated with two different phenomena, were numerically the same, for some reason. However, no one took this as something significant enough to address it seriously. But it is actually a huge deal, and one of the reasons is that it points out to gravity being a different kind of force. Really think about this. The thing that regulates the strength at which gravity operates is the thing that measures the "strength" of motion. No other force is like this: gravity was the only force of which charge is the same as the "strength" of motion.
2.Galilean Relativity
Another feature of Newton's mechanics is that it obeys Galilean Relativity. Which yielded a fatal problem for Newton's second law. I don't know how familiar you are with calculus, but I will try to translate in the end in any case. Galilean relativity says that the velocity of two frames of reference add together. That is, if you are moving with respect to some reference frame at velocity v, your reference frame will be $x'=x-vt$, where $x$ is the original reference frame. That means that if you change frames in these conditions (constant velocity), in Newton's second law, you get:
$$F=m\ddot{x}=m(\ddot{x'}+\ddot{vt})=F'$$
In other words, the equations of motion in a boat with constant speed are the same at seashore, while standing still; no experiment you make can distinguish between rest and constant velocity motion. For that reason, we call these kinds of systems (where $F=F'$) inertial, because they are equivalent to being still, or inert. I want you to notice that there is a characteristic of inertial movement that is very geometric: inertial movement traces out straight lines. It is very important that you immediately associate straight lines with inertial movement. It is the first link between motion and geometry.
Now notice what happens if you are instead moving with constant acceleration $g$, or in other words $x'=x-v_0t-\frac{1}{2}g\,t^2$
$$F=m\ddot{x}=m\ddot{x'}+mg$$ wait what? I thought Newton said F is acceleration times mass. Now there's a second term?
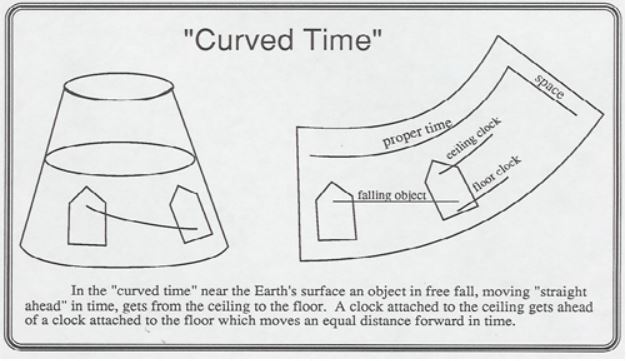
It turns out the second law is not valid for this kinds of systems. You have to introduce the effect of a "fictitious" force to account for the extra $mg$ term. This is highly distressing. We call these systems $non-inertial$, because they are not equivalent to being still. You can actually feel when you are moving. You feel that extra force right? Think of a car accelerating or a bus turning around a corner. You feel pulled against your car seat/ into the walls of the bus. Notice as well that non-inertial movement also has a geometric characteristic: curved lines. It is also very important that you associate non-inertial movement with curved paths. Anyway. This is the way they solved it back then.Add fictitious forces when the systems are not inertial, and call it a day: it will give the right results anyway. But like the mass equivalence problem, this one also points out to gravity being a different kind of force. When you are falling, you don't feel an extra force. Right? When you are falling, you feel weightless. This is because, in your frame, $m\ddot{x'}=-mg$ and so $F'=0$. So what gives? I thought accelerating frames were non-inertial? Why does a free-falling body have the properties of an inertial observer? Even more shocking! Gravitationally induced motion is curved, the trademark characteristic of non-inertial movement. How on earth can that motion be inertial?
Enter: Riemann, Gauss, and the advent of Differential Geometry
Around the middle of the XIX century, Riemann, a great german mathematician, published a paper that overthrew millenia of mathematics, Euclidean geometry. Riemann proposed a different geometry, a geometry of the differential, of calculus, of locality: a mathematical framework where you could represent and study the properties of curved surfaces of many dimensions. Gauss was also onto many of the things that Riemann discovered, beforehand, but didn't have as much courage to go against the current knowledge. He had been, however, thinking about something very relevant to both the problems we discussed earlier. He imagined he was a little bug, living in a flat piece of paper. He went about his day moving from point A to point B in a straight line. But what if the paper was curved up or down somewhere in the middle? Well, Bug Gauss would still move "in a straight line", as he locally wouldn't be able to tell he was in a curved space, as he was very small, but... he would be deflected around/towards the middle, depending on the sign of the curvature. Just like... gravity. In fact, it was overwhelmingly similar to the way gravity seemed to work. For example, how would you classify the motion of the bug? Inertial, or non-inertial? On one hand, the bug felt no acceleration during the proccess. No additional force (just like a free-falling observer). So maybe inertial? Buuut, on the other hand, his motion was curved... like a non inertial observer. Sounds familiar right? It is the same riddle gravity posed us! Inertial observers following curved paths!
Einstein and the happiest thought of his life
Glossing over all the work done on Special Relativity, the role of which, in General Relativity, is central, we arrive at the beginning of the XX century. Einstein is hoping to turn his theory of relativity into a theory of gravity, but he just can't seem to find a connection. Special Relativity is a success: however, it is only valid for inertial observers. One day, though, he does find it, in what he later described as the happiest thought of his life, which we have enunciated previously. A free-falling observer is equivalent to an inertial observer. A purely gravitational system experiences no forces whatsoever. When you fall, it's exactly the same as being still with gravity turned off. Only when you hit the ground do you feel a force. In this framework, Einstein finally was in a position to solve both gravity's problems. For the mass equivalence, well, of course they were equivallent! If a body in which only gravity applies is the same as an inertial body, either in straight line motion or still, then the inertial and gravitational masses have to be equivalent!! Give a push to the inertial body; the resistance is $m_i$ But whatever resistance is given by the inertial body has to be the same as the gravitational one, by this principle of equivalence that Einstein postulated. For the relativity problem, well, if the gravitational system is in some sense inertial, then it follows that some modified notion of inertial preserves a modified version of Newton's laws. It has to have the following features: the new modified notion of inertial has to acommodate curved paths; the new notion of force has to be null in these kinds of systems, from every reference frame. This might also sound familiar: this notion of inertial is precisely the same sense in which Gauss's bug in a curved plane was inertial: curved motion but no force. This is where the link from gravity to geometry accomplishes its final step. Construct a geometric structure, akin to the paper in which the bug lived in, such that the paths induced by gravity will be inertial. It had already been shown by the german mathematician Hermann Minkowski that a special structure composed of points of space and time (spacetime) worked very naturally with Special Relativity ( in a sense that I probably shouldn't explain here, as this answer is already too long) so this geometric structure was the perfect candidate. Make it curved, impose the constancy of the speed of light for all observers, make it coincide with Newton's laws of motion for small speeds and weak gravitational fields (the so called non relativistic weak field limit) and ta-da. You have General Relativity.
I have always found that learning things from a historical point of view improves greatly upon my understanding of a subject. We can see why we deal with some things instead of others. When you ask the question: how does spacetime generate gravity. There's a ton of questions already in there. Why do we even speak of spacetime in the first place? When people speak of it, it may seem like it's this metaphysical substance-entity that is invisible all around us. It is not. Spacetime is a concept invented to acommodate the equivalence between motion and geometry. Curved spacetime is a concept invented to acommodate the equivalence between gravity, and curved inertial motion. When we say that spacetime curvature generates gravity, this is really what we mean; that we as mankind have arrived at a model that describes gravity as inertial motion in a curved geometry, rather than non inertial motion in flat geometry, because it is more consistent with our previous models of the laws of physics, which had a few incongruencies. Had Newton defined force in a different way, or inertial in a different way, and we might not have invented spacetime as we know it; perhaps some other version, or none at all.
I know I have not explicitly answered the details of how gravity is a result of curved spacetime. All the answers did a pretty good job at that anyway; energy and momentum curve space time, matter follows geodesics (the generalization I was talking about of inertial path, that can be curved), all that stuff. But hopefully from this exposition you gained a little more insight into what "spacetime curvature generates gravity" means, which is:
Curved spacetime is the geometry in which gravity becomes, in a suitable sense, inertial.