Rolling ball travelling in tube with loop vs straight tube

Question

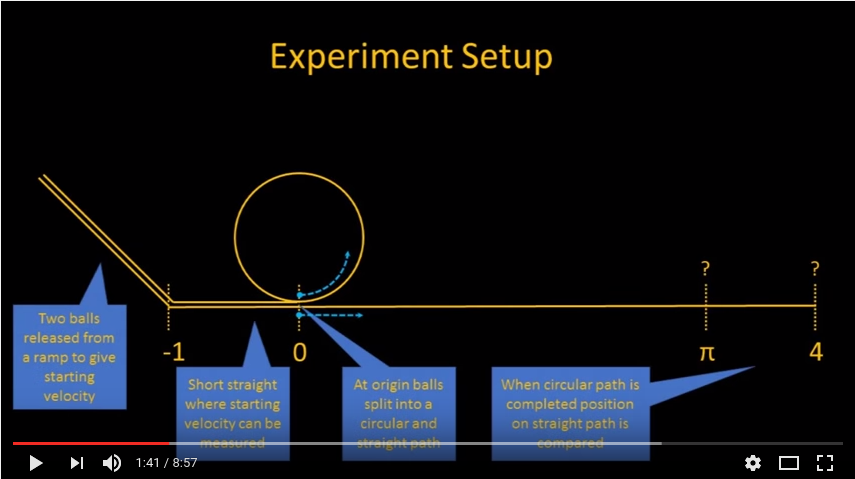
I found the following video on youtube which demonstrates a simple experiment whereby two balls are rolled in tubes. Both balls start on an incline to gain speed. Once the incline hits the table, one ball travels on a loop which is laid out on the table, while the other ball continues straight.

Here is a screenshot of the video showing the setup. Note that the schematic is misleading with respect to the orientation of the loop -- the loop is laid out on the table, not vertically as the schematic would lead you to believe (watch the video to fully understand).

The person who created the video has an explanation of the results which doesn't really make sense (he claims that 4 can be used in place of pi for modelling circular motion). I am not good enough at physics to figure out the correct formulas for the motion of the balls and the final result of the experiment.

What are the correct formulas for modeling this experiment?

EDIT: here are some measurements collected from later on in the video in case that helps someone:

tube outer diameter: 16mm
tube inner diameter: 12mm
metal ball diameter: 8mm
metal ball weight: 2.1 grams
launch ramp around 15mm high
circular path inner tube diameter: 16cm
circular path middle of tube diameter: 17.6cm
length of straight path(starting at point labeled "0" to point labelled "4") is 70.4 cm 

EDIT2: I found an answer by one "Robert Rust" in comments on another video in which he proposes a kind of complicated derivation taking in to account various forms of kinetic energy. I need to study more about reference frames and the like to determine whether it is reasonable or not, but it does match the experiment closely.

To get a proper understanding of the motion of the two balls, one can realize that the ball that enters the circle has a rotating reference frame. The ball in the straight line has a constant non rotating inertial reference frame. The rotating frame is made to be a circle, making formulas simpler and calculations easier.

A. The linear moving ball has linear speed V plus rotational speed W about its own centre. The moment of inertia of the ball about its centre is I = 2/5mr², where m is the mass of the ball, V is its forward velocity, W is its rotational speed, and r is the radius of the ball.

The total energy of the linear moving ball is:

1/2mV² + 1/2W²I = 1/2mV² + 1/2(V/r)²(2/5mr²) = 7/10mV² (A)

B. The velocity of the ball in the rotating reference frame (going around the loop) has two components: 1. one from the motion of the ball itself, and 2. another from the frame's own rotation (ball going in a loop - an orbit basically).

... [I am omitting portions of his explanation]

We can calculate the ball's energies in the loop:

1. Ball motion:

Linear Energy = 1/2v²m (a)

Rotational Energy = 1/2w²I = 1/2w²(2/5mr²) = 1/5(v/r)²mr² = 1/5v²m (b)

where v/r = w, the rotational speed of the ball around its own centre as it goes around the loop

2. Frame motion: Rotational Energy = 1/2Jw² = 1/2(mR²)(v/R)² = 1/2mv² (c)

where J = mR² = the rotational inertia of the ball going around the centre of the loop circle R = radius of circle (the ball is in orbit around the circle's centre)

Thus the total energy of the ball in the rotating frame of reference in the circle is:

(a) + (b) + (c) = 1/2mv² + 1/5mv² + 1/2mv² = 6/5mv² (B)

Ignoring friction due to centrifugal (centripetal) forces between the ball and the loop, energy will be conserved as the ball moves from the straight line to the loop line.

Thus from above, (A) = (B), or

7/10mV² = 6/5mv², where V = the speed of linear ball, and v = the speed of ball in loop.

or v =√( 7/12)V = 0.764V or v/V = 0.764 (d) BY CALCULATION USING PHYSICS

"Robert Rust"'s explanation closely matches my measured ratio of the velocities of ~0.79. Is this explanation complete nonsense or is there something too it?

EDIT3: Sammy Gerbil below explains why "Robert Rust"'s explanation is incorrect and shows several different factors which can be taken into account to explain the observed motion.

Answer 1

Updated Answer

Apart from rolling friction (suggested by Noah) there are other reasons which might explain some of the discrepancy more simply.

1. While rolling along the straight tube the ball bearing is at the lowest point. In the circular section it rises up the side of the tube because of centrifugal force. If moving fast enough it travels along the path furthest from the centre of the circle. The experimenter measured the diameter of the path from the middle of the tube, assuming the ball will be at the lowest point. So the calculation of the diameter is incorrect.

If the inner diameter of the loop is 160mm then the diameter of the path taken by the ball is 160+2(2+12)=188mm and not 176mm.

2. The ball has risen vertically when travelling in the loop, from the bottom to the side of the profile. It has gained gravitational potential energy and lost an equal amount of kinetic energy and therefore speed. The inner diameter of the tube is 12mm and the diameter of the ball is 8mm, so the CM of the ball rises by (12-8)/2=2mm when in the loop.

3. The launch ramp is stated to be about 150mm high. This should be measured at the same point of the tube, from the table to the raised up position. The ratio of the speeds of the ball in the straight section and loop is
   $\sqrt{\frac{150}{150-2}}=1.0067$
   This ratio could be higher if the actual height through which the ball drops is less than 150mm. For example, the experimenter may have measured the height from the table to the centre of the cross section of the raised tube. In this case the ball drops through a height of 142mm and the ratio of speeds becomes 1.0071.

In the time it takes the ball to go round the loop, the other ball in the straight tube should cover a distance of $188\pi \times 1.0067= 595$mm. This is still significantly short of 704mm, but it is an improvement on 176$\pi$=553mm. The uncertainty in ramp height could bring us closer. And there will be some contribution from rolling friction.

4. There may also be an error in measuring the inner diameter of the loop. The loop does not close - as you can see at the '0' mark - so it is not circular. It starts with a large diameter and ends with a smaller diameter. Which diameter has been measured?

5. Another possibility is that the experimenter tilted the table slightly to compensate for friction. A ball inserted into the tube at the LHS and given a small push then rolls to the RHS with constant speed instead of coming to a halt. When the ball is in the loop it is rolling uphill for half of its journey, gaining PE and losing KE and speed, making the time in the loop longer. This might be what Noah was alluding to when he wrote "Assuming there is no vertical tilt in the tube, ..."

---

Ralph Rust's method of calculation is invalid. The values of (a) and (b) are equal because these are different names for the same linear (or tangential) motion of the CM of the ball. So in the rotating frame he is counting this contribution twice.

---

Original Answer

Noah's suggestion that the ball travelling round the loop is slower because of increased rolling resistance is a good one. I cannot think of any obvious alternative explanation.

Rolling resistance is proportional to normal reaction. On the straight track the normal reaction is just $mg$, the weight of the ball bearing. On the looped track there is also a centripetal force $\frac{mv^2}{r}$ where $r$ is the radius of the loop and $v$ is the speed at any point.

I shall assume that rolling resistance due to weight is negligible. This can be revised if the model does not fit the results. Then when going round the loop the centripetal force is equal to the normal reaction $N$, so the rolling resistance is $F=CN=C\frac{mv^2}{r}$ where $C$ is a constant which depends on the properties of the tube and the ball bearings.

The equation of motion when going round the loop is
$m\frac{dv}{dt}= -F= -Cm\frac{v^2}{r}$
$\frac{dv}{dt}= -C\frac{v^2}{r}$
which can be integrated to give
$\frac{1}{v}=\frac{C}{r}t+\frac{1}{u}$
where $u$ is the speed of the ball when it enters the tube. $u$ is also the constant speed of the ball bearing on the straight track.

Further integration using $v=\frac{dx}{dt}$ gives
$t=\frac{r}{uC}(e^{Cx/r}-1)$.
The time taken the go around the loop (a distance of $x=2\pi r$) is therefore
$T=\frac{r}{uC}(e^{2\pi C}-1)$.

The distance travelled by the other ball bearing during the time it takes to go around the loop is $uT$. If you have made measurements of $u$, $r$ and $C$ you can predict the distance $uT$.

The difficulty is that to find $C$ you need to measure $T$. However, if you have done that you might as well just use the measured valued because the predicted value is calculated from the measured value.

The only useful things you might do with this analysis are (i) check the model for rolling resistance by seeing how well the formula for $T$ fits with measurements, or (ii) using measurements of $T$ to find values of $C$, which will vary with the materials used.

For (i), measure $T$ for various combinations of $r$ and $u$. Plot $T$ against $\frac{r}{u}$. This should give you a straight line through the origin.

However, if you are not doing the experiment yourself then you can only rely on measurements from the video. Perhaps you can contact the guy in the video to ask for his recording of the experiment, to get a more accurate values for $u$ and $T$.

---

So this model cannot confirm the experimental result. You can only do that if you can find the value of $C$ before doing the experiment.

By the way, as Noah says, the purpose of the experiment (to show that $\pi=4$) is nonsense.

Answer 2

This is just a poorly done experiment.

(Also the fact that the length is pi is not a terribly important measurement. What he is really trying to say is that the time it takes a ball to roll around a circle is longer than it takes to travel the same distance (as the circumference) in a straight line.)

We can see that this is patently absurd: two particles moving with identical velocity (or, in this case $|v|$) should cover the same distance in the same time (putting aside relativity).

Assuming there is no vertical tilt in the tube, if the balls were either sliding or rolling on a perfectly rough surface, he would not observe the "straight" ball going faster.

The difference comes about because this is far from a perfect setup, and does not come close to either of these scenarios.

My guess is that the first order cause of this is (as you might guess) friction. There is absurd amount more friction in a bent tube: obviously the balls are not rolling perfectly so the balls are sort of being pushed up against the horizontal wall (the source of centripetal acceleration) which leads to an increase in the magnitude of normal force $\rightarrow$ increased friction (loss of energy/KE/reduced speed).

Another way of putting this is the horizontal velocity which both balls start with is not perfectly translated into the (continuously varied) velocity of the ball moving in a circle.