I am interested in finding pressure of neutron star! So. Please could any tell me how to choose central density for the inner and outer core of neutron Star. What numeric value should me in both core. Also what should be the polytropic index gamma for outer core.
$\begingroup$
$\endgroup$
1
-
1$\begingroup$ How can you have a "central density" for an "outer core"? $\endgroup$– ProfRobCommented Nov 8, 2016 at 7:37
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
2
The central density of a neutron star is unknown, but probably lies in the region of $\sim 10^{18}$ kg/m$^3$, depending on the details of the composition, the equation of state and of course the mass of the neutron star.
As an order of magnitude estimate, at these densities, the pressure approaches the maximum for a relativistically degenerate ideal fermion gas of $P \simeq \rho c^2/3 = 3\times10^{34}$ Pa.
By "outer core" I assume you mean the neutron fluid region. Here the density could reach somewhere between $3\times 10^{17}$ and $\sim 10^{18}$ kg/m$^3$ before something may (or may not) cause a phase change (hyperons, quark matter etc.). The pressure at the upper end of this range would approach that which I gave above. A more formal calculation could calculate the equilibrium composition of an n,p,e gas and then use the appropriate formula for an ideal Fermi gas to estimate the total pressure. Such a calculation, at a range of densities, also gives you an estimate of the adiabatic index. I find that the pressure of an ideal n,p,e gas is $7\times 10^{32}$ Pa at $3\times 10^{17}$ kg/m$^3$ (completely dominanted by non-relativistic neutrons), rising to $5\times 10^{33}$ Pa at $10^{18}$ kg/m$^3$. The adiabatic index $\gamma$, (where I define $\gamma$ in terms of $P \propto \rho^{\gamma}$), is approximately 1.6 over this range.
You have to realise though that this is an unrealistic equation of state at these densities. As the neutrons get closer together ($\leq 10^{-15}$ m) they must interact and this hardens the equation of state such that $\gamma \geq 2$, otherwise neutron stars of mass up to $2M_{\odot}$ couldn't exist! Indeed, one definition of the "inner core" might be where this hardening happens.
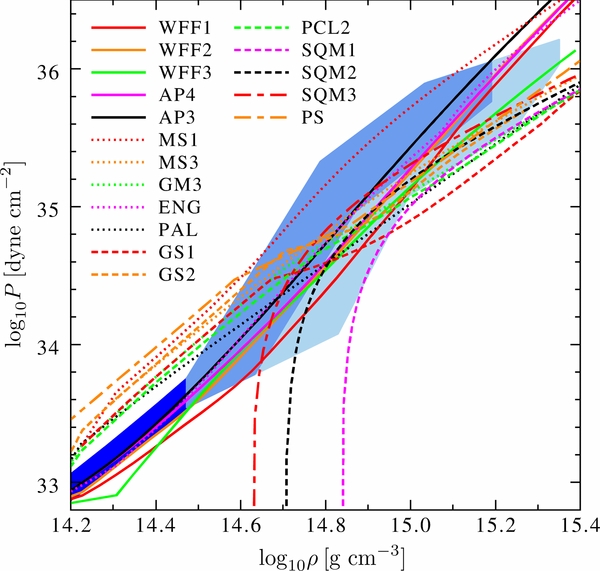
A recent paper by Hebeler et al. (2013) includes the following plot (in cgs units) which reviews the many possible equations of state in the density range I have discussed above. For reasons discussed in that paper, the authors favour the AP3 or AP4 equations of state which give higher pressures tan the simple n,p,e approximation. The slope of these graphs gives the the value of $\gamma \simeq 3.3$ for AP3 and AP4 for $\rho > 5 \times 10^{17}$ kg/m$^3$.
-
$\begingroup$ Hmm yes siR thanks! well what dou you think so if I take central density = 9. 99*10^14, central pressure 1. 385*10^35 , polytropic index gamma as 2. 8535 and integrate T. O. V EQUATION for presssure. Will its describe the outer core state which is mainly composition of neutron-protron fermi liquid + few% of electron fermi gas. $\endgroup$ Commented Nov 8, 2016 at 9:32
-
$\begingroup$ @umarkhan It will give you a result, but the outer parts of a neutron star would not be well characterised by such an equation of state. $\gamma$ is smaller in the n,p,e fluid below nuclear densities and in the crust. $\endgroup$– ProfRobCommented Nov 8, 2016 at 9:41