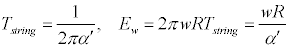Massless particles in a universe with compact extra-dimensions

Question

One common idea behind many extensions to the Standard Model (such as String Theory or Kaluza-Klein Theory) are small or hidden "Extra-Dimensions", that are compactified.

According to my understanding of Quantum Physics, this would result in each particle's wavefunction having a component into the direction of these extra-dimensions, and only discrete energy-states would be allowed (similar to electrons in an atom).

Now imagine a photon, which is considered to be a particle without rest mass in the Standard Model. Its wavefunction would also have components into the direction of the extra-dimensions. Consequently, it would have to occupy one of these energy states. So there would be some energy consisting out of the photon's standing wave in the extra dimension, which - according to my understanding - would behave just like a finite rest mass of the photon.

So how can there be particles without any invariant mass in a theory with compact extra dimensions?

Answer 1

Let the speed of light $c=1$ be one for simplicity. Recall the mass-shell condition e.g. in 5D Kaluza-Klein theory,

$$ E^2~=~{\bf p}^2 + m_{4D}^2, \qquad m_{4D}^2 ~=~ p_5^2+ m_{5D}^2.$$

So taking OP's argument to its logical conclusion, we see that a massless particle $m_{4D}=0$ (from a 4D perspective) has no Kaluza-Klein momentum $p_5=0$, and no 5D mass $m_{5D}=0$.

[We can repeat this argument ad infinitum: A massless particle $m_{5D}=0$ (from a 5D perspective) has no Kaluza-Klein momentum $p_6=0$, and no 6D mass $m_{6D}=0$, and so forth.]

Answer 2

The string tension Tstring is the energy per unit length of the string. If the string is wound w times around a circular dimension with radius R, then the energy Ew stored in the tension of the wound string is

The mass of an excited string depends on the number of oscillator modes N and Ñ excited in the two directions of propagation around the closed string, minus the constant vacuum energy. Kaluza-Klein compactification adds the quantized momentum in the compact dimensions, and the tension energy from the string being wrapped w times around the compact dimension, so that the total squared mass becomes

As an experimentalist skimming over theory I am satisfied that zero masses can exist even with compactified dimensions, since there exists a minus sign in the mass formula. :).

This also helps:

The theory gains extra massless particles when the radius R of the compact dimension takes the minimum value possible given the above symmetry of T-duality

One will have to do the maths to really convince oneself of the above statements, but that takes time and effort.