What are the differences between a $\psi$-epistemic ontological model and a $\psi$-ontic model of quantum mechanics, exactly?

Question

I am confused about the differences between a $\psi$-epistemic ontological model of quantum mechanics and a $\psi$-ontic one.

The way I understand it, a $\psi$-epistemic model says that every quantum state does not correspond to a physical state, and I understand this is to be interpreted as saying that quantum mechanics isn't a complete description of reality.

However, I also understand that an ontological model assumes that there is a way to assign a complete (deterministic) description of reality, and this seems to point to a $\psi$-ontic model, if I get that one correctly.

Where am I going wrong here, and how exactly should one interpret these terms?

Answer 1

You seem to be rather confused regarding those terms. The terms $\psi$-epistemic and $\psi$-ontic are mutually exclusive when describing an interpretation of quantum mechanics.

Both of these terms are possible characteristics for an ontological model: a description of the set $\Lambda$ of possible "complete descriptions of reality", which are often denoted by $\lambda$ and termed ontic states.

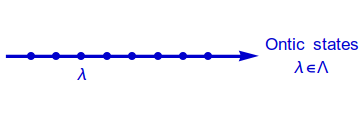
In other words, an ontological model is a descriptions of the things that "exist" in the real world. On that stage, there are two types: $\psi$-ontic models, where the wavefunction "exists", and $\psi$-epistemic models, where it doesn't.

More concretely:

• In $\psi$-ontic models, the wavefunction is a physical property of the "real" state of the world. That is, if I were to obtain a complete description of reality for the state of the system, then I can deduce the wavefunction $\psi$ from this state. Graphically, such models look like this:

  

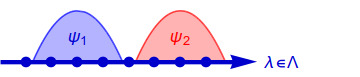
  Note, however, that this does not fully rule out interpretations of $\psi$ as a statistical quantity: it can still be a distribution over a set of real states, with each wavefunction corresponding to a distinct set of states.

  • As a subset of these models, if the real state of the system turns out to be fully informationally equivalent to the wavefunction, the model is called $\psi$-complete.

    

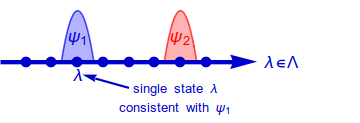
    In such a model, if I know the wavefunction, then I know all that there is to know about the system. This rules out e.g. hidden variables

• In $\psi$-epistemic models, the wavefunction is not a physical property, but rather a statistical quantity and really just a description of our state of knowledge about the system. More concretely, a model is called $\psi$-epistemic if it allows the existence of two different wavefunctions that are consistent with the same "real" state of the system.

  

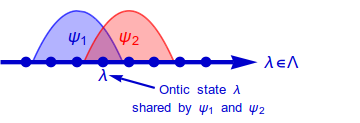
  In particular, this means that you cannot deduce the wavefunction from the ontic state of the world.

In terms of how you phrased it in the question,

[the way $\psi$-epistemic models should] be interpreted is that quantum mechanics isn't a complete description of reality,

that is correct but not quite there. In $\psi$-epistemic models QM isn't a complete description of reality, but that is also the case in $\psi$-ontic models that are not $\psi$-complete.

For more details, see the paper that (to my knowledge) introduced these terms with precise definitions:

Einstein, incompleteness, and the epistemic view of quantum states. N Harrigan and RW Spekkens. Found. Phys. 40, 125 (2010), arXiv:0706.2661.

---

^{Mathematica source for the graphics: Import["http://goo.gl/NaH6rM"]["https://i.sstatic.net/vtA9o.png"].}