0
$\begingroup$

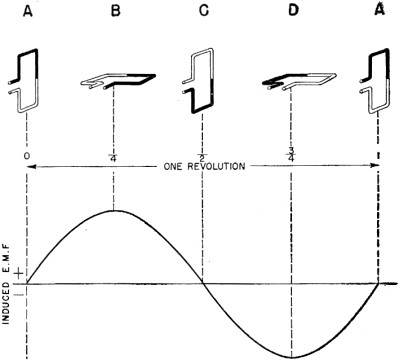
An example for a graph representing an a.c. generator (a rectangular coil of wire rotating in between a magnet) would be a sine graph, because the surface for magnetic flux are the arms of the coil or the conductors AB and CD. However, if the surface has been changed to the area of the rectangular coil, which is the area where the magnetic field of the magnets pass through, then would the graph still be the same?

If the graph is still the same, then my guess would be that when the coil of wire is vertical (the surface for flux is still the area of the coil), the magnetic flux would be at its maximum, and because of it being maximum, the rate of change of magnetic flux would become 0, because at that point, the magnetic flux cannot exceed the maximum over time. And, hence the induced e.m.f will also be 0. But then, it doesn't explain why the induced e.m.f will be positive at first, because there's a negative rate of change of magnetic flux from its vertical to horizontal position from 0 to 1/4 of a revolution graph

Improve this question
$\endgroup$
2
  • $\begingroup$ The question is unclear and IMO must be closed. The reason : What is the direction of the (let be uniform) magnetic flux density vector $\:\mathbf{B}\:$ ??? From left to right or from right to left ??? Which end of the coil defines as (+) and so the other as (-) for the e.m.f ??? The black end or the white end ??? Playing with the magnetic flux direction and/or the polarities of the coil ends you have positive or negative e.m.f at the time moment the coil from its vertical position is rotating clockwise. $\endgroup$
    – Voulkos
    Commented Jan 4, 2018 at 22:50
  • $\begingroup$ @Frobenius If this platform only answer google-able questions, then what's the point of the platform. If you intend to help the OP, your job is not that you understand what the underlying physics is, your job is try to pin point what misunderstanding the OP has. $\endgroup$
    – eliu
    Commented Jun 13, 2020 at 14:47

3 Answers 3

Reset to default
1
$\begingroup$

Your graph is a plot of emf vs time. The cosine depends on the angular position of the loop. The amplitude depends on the area.

Improve this answer
$\endgroup$
0
$\begingroup$

As long as the coil is planar the graphs will be the same irrespective of the shape of the coil.

You have forgotten the minus sign in $\mathcal E = - \frac {d\Phi}{dt}$ as a decreasing magnetic flux linkage leads to a positive emf.

Improve this answer
$\endgroup$
0
0
$\begingroup$

I believe, somewhere in your thought process, you made a mistake on +/- on something. Try to use this picture and follow your own thought process again enter image description here

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.