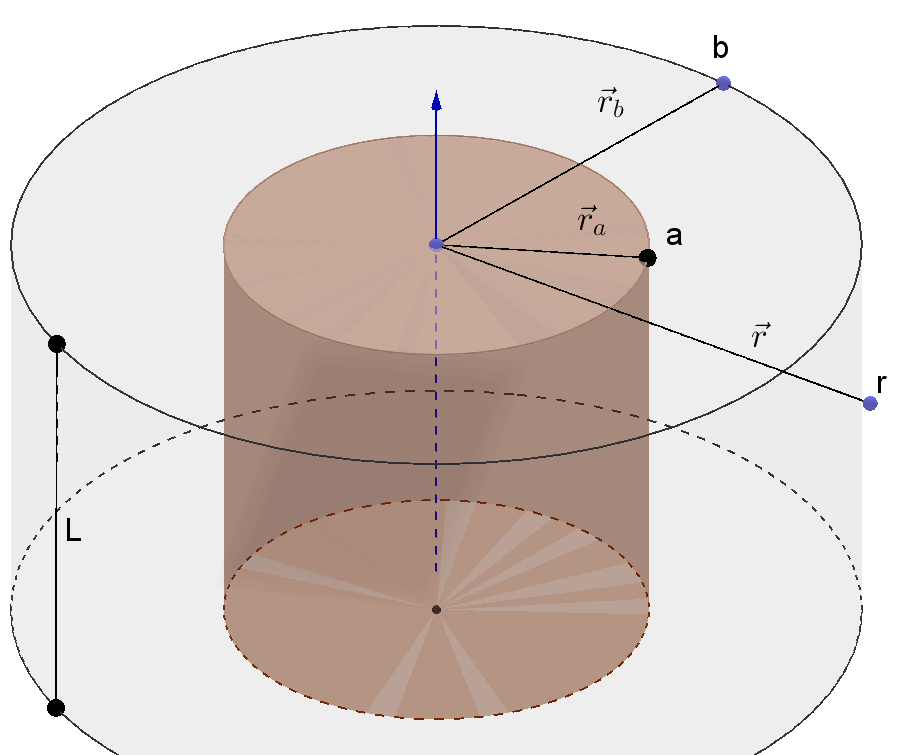
I having a problem with the line integral of electric potential.
I have a cylinder of radius $a$ and length $L$ with a uniformly surface charge. At point $b$ the potential is zero. I want to calculate the potential a $r$, i.e. $r>b$.
The integral is $\int_\gamma \vec E \,\, \cdot d\vec l$ and the electric field is $\vec E(\vec r)=\frac{Q}{2\pi r L\epsilon_0} \hat r$ (cylindrical coordinates, $\hat r=\hat x\cos\phi+\hat y\sin \phi$).
In multivariable calculus I use $\int_L \vec E \, \, \cdot d\vec r$ and parameterize the curve $L$ so $\int_L \vec E \, \, \cdot d\vec r= \int_P \vec E (\vec r(t)) \, \, \cdot \frac{d\vec r(t)}{dt}dt$.
But what is $d\vec l$?
I'm lost here, how can I find a curve to parameterize?