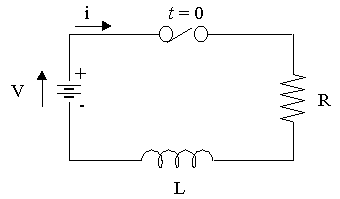
I am self-studying electromagnetism right now (by reading University Physics 13th edition) and for some reason I always want to understand things in a crystalclear way and in depth. Now look at this simple RL-circuit:
I am assuming the wire is superconducting and suppose at $t=0$ the switch is turned on. My problem is that I want to come up with this differential equation for this circuit: $V-I\cdot R=-L\cdot \frac{dI}{dt}$. I know that I can't use Kirchoff's voltage law $(\oint \vec{E}\cdot d\vec{l}=0)$, because there is a non-conservative electric field near the inductor. Therefore I use Farayday's law of induction: \begin{equation} \mathcal{E}=\oint_{\Gamma} \vec{E}\cdot d\vec{l}=-\frac{d}{dt} \int_{S} \vec{B} \cdot \hat{n}\,dA \end{equation}
where $\Gamma$ is a closed loop and $S$ is the open surface attached to the loop. Now, my textbook says:
In general, the total field $\vec{E}$ at a point in space can be the superposition of an electrostatic field $\vec{E_c}$ caused by a distribution of charges at rest and a magnetically induced, nonelectrostatic field $\vec{E_n}$. That is $\vec{E}=\vec{E_c}+\vec{E_n}$.
Inserting this in Faraday's law I get:
$$\oint_{\Gamma} \vec{E}\cdot d\vec{l}=\oint_{\Gamma} \vec{E_c}\cdot d\vec{l}+\oint_{\Gamma} \vec{E_n}\cdot d\vec{l}=-L\cdot \frac{dI}{dt}$$ Now by definition: $\oint_{\Gamma} \vec{E_c}\cdot d\vec{l}=0$ since $\vec{E_c}$ is conservative. This gives: $\oint_{\Gamma} \vec{E_n}\cdot d\vec{l}=-L\cdot \frac{dI}{dt}$, which is perfectly fine and consistent with my textbook. However it also gives (going counterclockwise in circuit): $\oint_{\Gamma} \vec{E_c}\cdot d\vec{l}=-V+I\cdot R=0$ which it shouldn't. I think my flaw is here, but I've tried to think about this this whole day with no succes.
I have my inspiration from Walter Lewin's video: http://youtu.be/LzT_YZ0xCFY?t=26m31s but I still can't get the equation by Faraday's law.
Any help is greatly appreciated.