2
$\begingroup$

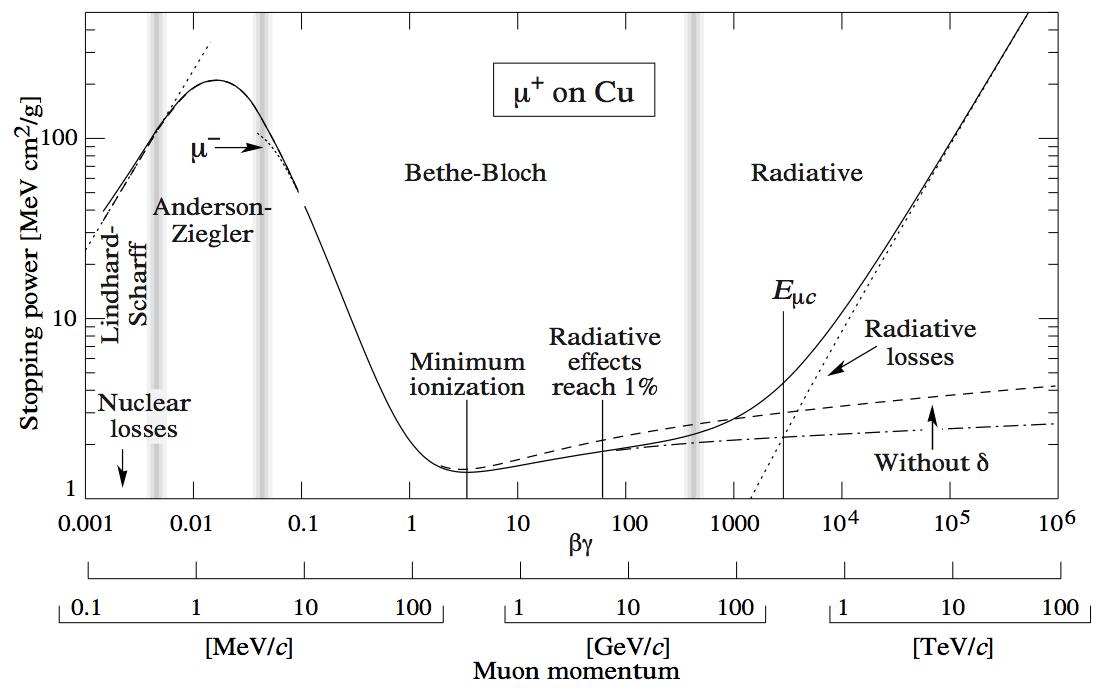
So I have this diagram of how the stopping power of muons changes with energy:

enter image description here

Depending on energy different equations are used to describe the stopping power variation. Now, currently I'm reading about external radiation therapy with charged particles (especially protons). And my teacher have stated that the stopping power of a proton can pretty much be described by the Bethe equation, since it holds for the ranges of energies that proton therapy uses (The diagram is probably a bit different for protons than for muons). And yes, that does make some sense I think. My question is then: The particles, it being tissue or any other type of matter, will eventually stop, i.e. having no energy at all. So in turn, wouldn't that mean that you would have to include Anderson-Ziegler, Lindhard-Scharff corrections in order to get the correct stopping power, or am I missing something (Assuming the diagram looks just a bit like the one above). Again, I've been told the Bethe equation is "good enough" for proton therapy in patients, so I am not sure if it also holds if I were to fire at an iron target or something.

Thanks in advance.

Improve this question
$\endgroup$
4
  • $\begingroup$ What is meant is that the particles are being stopped very abruptly once they fall below the minimum ionization threshold. The double logarithmic plot shows you that at momenta below 100MeV/c the stopping power of matter goes up very significantly, so not only do the particles have less momentum to lose, they are also losing it up to a hundred times faster (per unit distance). If you solve the (effective) equations of motion, this leads to an abrupt stop over a short distance that is pretty insensitive to details. $\endgroup$
    – CuriousOne
    Commented Jun 20, 2016 at 21:29
  • $\begingroup$ So basically it just means, that when the Bethe equations stops being valid, the energy of a particle is so low, that any real contribution (at least in the case of proton therapy) to transfer energy to the matter is insignificant ? $\endgroup$
    – Denver Dang
    Commented Jun 20, 2016 at 21:58
  • $\begingroup$ That's the usual assumption for many detectors and I have heard it (and this is hearsay) from colleagues who were involved in proton and heavy ion radiotherapy. Having said that, I don't claim any expertise and I never looked at the actual difference between e.g. a hypothetical scenario with nearly flat energy loss below the minimal ionization energy and the real mechanism. I think the better way to say this is not that it's not important, it's actually quite important, it's probably just not sensitive to the details. $\endgroup$
    – CuriousOne
    Commented Jun 20, 2016 at 22:05
  • $\begingroup$ I will keep that in mind :) Thank you very much. Post an answer if you wanna receive points ;) $\endgroup$
    – Denver Dang
    Commented Jun 20, 2016 at 22:10

1 Answer 1

Reset to default
3
$\begingroup$

During proton therapy, most of the damage is actually done in the last few mm before the beam stops - at the point called the Bragg Peak

Yes, the penetration distance is largely determined by the energy above a few MeV; as the particle slows down, it starts to dump more energy per unit length. Quoting from "The physics of protons for patient treatment" (Wroe, Slater and Slater):

Protons and other heavy charged particles come close to fulfilling this objective: they deposit most of their energy in a high-dose peak (known as the Bragg peak) at the end of their track (Fig. 1). This peak is created through an exponential increase in stopping power towards the end of the protons’ track. Hence, as a heavy charged particle (such as a proton) slows down, the amount of energy it deposits per unit length covered increases exponentially, creating a high-dose peak (2). The depth of this peak in a given material (such as a patient) depends on its initial energy; varying this energy allows the high-dose region to be placed at any depth.

And figure 1 from the paper:

enter image description here

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.