Total Angular Momentum of Hadrons

Question

Hadrons are made of 2 or 3 quarks. Quarks have spin 1/2, so Mesons can have spin S = 1 or spin S = 0, and Baryons can have spin S = 3/2 or spin S = 1/2. Is there orbital angular momentum of the quarks moving around each other in the Hadron? Is this accounted for when discussing the angular momentum of a free Hadron?

Answer 1

Quarks as elementary particles are quantum mechanical entities.

The same is true of electrons and protons. The hydrogen atom has the simplest quantum mechanical solution of how quantum mechanical particles are bound by attractive forces. This solution is a wave function, and it tells us that the electron is in a quantum mechanical probability locus around the center of mass, called an orbital. This on average reproduces the concept of the Bohr atom, where the electron is trapped in planetary like orbit in a quantized state. Where is the angular momentum? The angular momentum is in the quantum numbers of the quantized state. If the electron is in one of these states the whole atom is characterized by the corresponding J which is the sum of the m and l quantum numbers and characterizes the hydrogen atom's angular momentum.

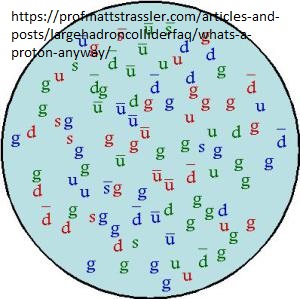
When coming to a nucleon and quarks the situation cannot be approximated by a planetary model in no way, as not only the potential is not a 1/r potential but proportional to r, the structure of a proton does not contain only the three quarks but also the gluon interactions and the quark antiquark pairs that make a soup. There are so many angular momenta possible that it is only conservation laws that can estimate the overall angular momentum of the soup, and thus of the nucleon or meson. Here is a sketch of how a proton is composed of quarks, not a planetary model indeed. :

It is an experimental fact that there exist spin states higher than 1/2, 0 or 1 in the table of resonances but the particular mechanicsm that will constrain the spin states and the angular momenta will have to depend on the particular form and approximations used in the model calculations. The terminology of "orbital angular momentum" may characterize the angular momentum quantum number still , but is only historically valid.

For example:

Quark Orbital Angular Momentum from Lattice QCD

We calculate the quark orbital angular momentum of the nucleon from the quark energy-momentum tensor form factors on the lattice. The disconnected insertion is estimated stochastically which employs the Z2 noise with an unbiased subtraction. This reduced the error by a factor of 4 with negligible overhead. The total quark contribution to the proton spin is found to be 0.30±0.07. From this and the quark spin content we deduce the quark orbital angular momentum to be 0.17±0.06 which is ∼34% of the proton spin. We further predict that the gluon angular momentum to be 0.20±0.07, i. e. ∼ 40% of the proton spin is due to the glue.

Answer 2

To perhaps give an answer in a slightly different way, it's complicated. One must keep in mind that the decomposition of the angular momentum into spin and orbital components is model dependent. In the language of the renormalization group, it is scheme and scale dependent.

If you probe a hadronic system at a given length scale (or, equivalently, energy) you will detect a certain content of quark-antiquark pairs. If you then probe with a shorter lendth scale (higher energy), you will see more quark-antiquark pairs, and they may have some angular momentum. So the fraction of angular momentum carried by the valence quarks relative to the fraction carried by the quark-antiquark sea depends on the momentum scale of your probe (scale dependence). It also depends on the details of how you cut off the missing physics (scheme dependence).

The same is true of an electron in a hydrogen atom. However, the dependence on the momentum scale is so weak that you can ignore it at any relevant scale.