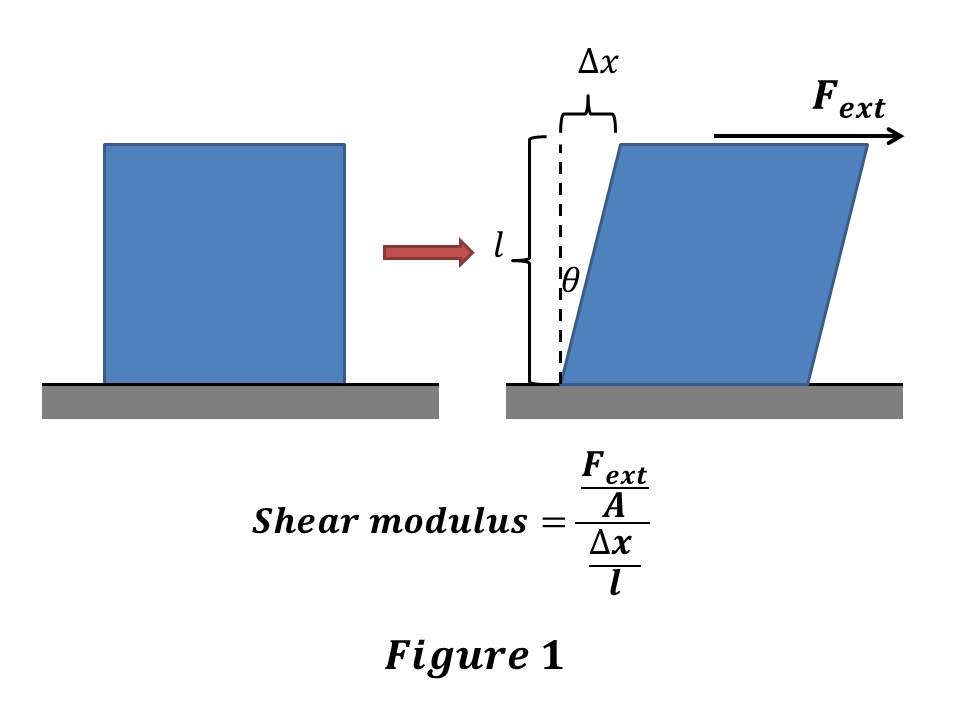
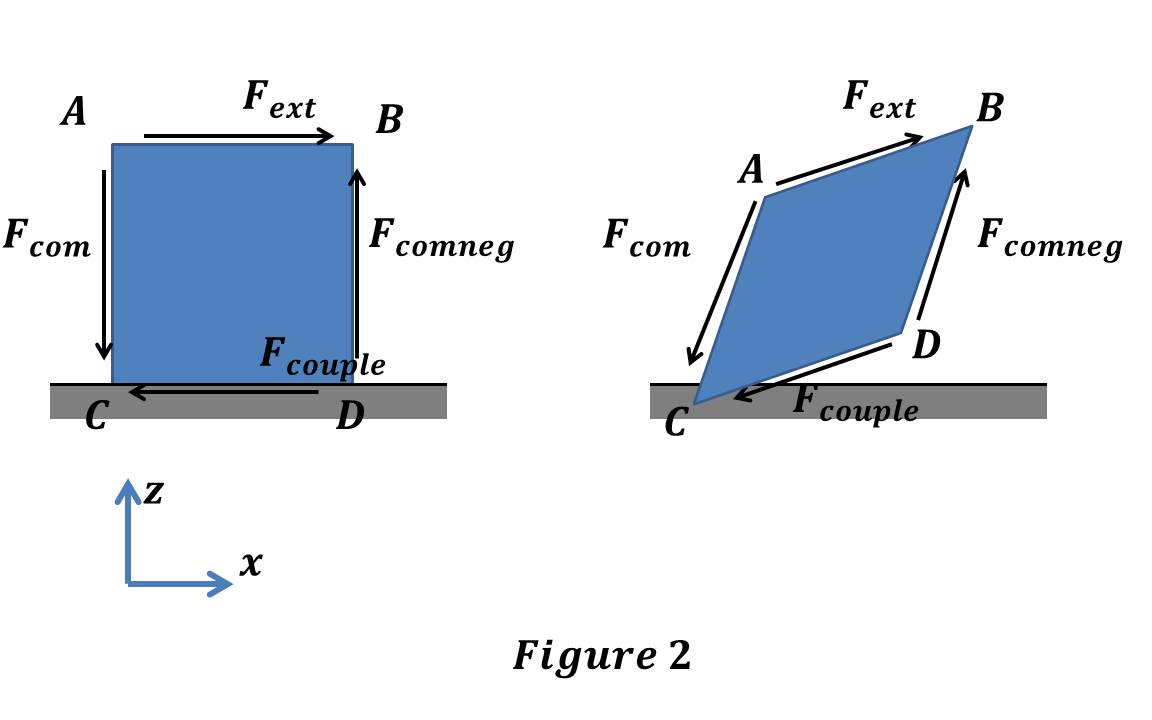
I am a self-learner of continuum mechanic. I am confused about simple shear stress in situation similar to figure 1,
in case $F_\textrm{ext}$ is caused by external perturbation by i.e., human, what are the forces that involve in this scenario? In my mind, there are
$F_\textrm{ext}$ is caused by external perturbation
$F_\textrm{couple}$ which $F_\textrm{couple}=-F_\textrm{ext}$
$F_\textrm{com}$ which are the complementary shear stress multiply with area
$F_\textrm{comneg}$ which $F_\textrm{comneg}=-F_\textrm{com}$ however, these three forces could result in figure 2, not figure 1.
Therefore, I think there should be constraint forces i.e., from human hand, surrounding material or floor that eliminate the force component in $z$ axis by reaction forces.
- $F_\textrm{reac}$ which equal to Force components in $z$ axis, $F_\textrm{reac} =-F_\textrm{com}$
Is that right?
in the case of dynamic deformation,it will not necessary that $F_\textrm{couple}$ equal to $-F_\textrm{ext}$ and $F_\textrm{comneg}$ equal to $F_\textrm{com}$. $F_\textrm{reac}$ equal to force components in $z$ axis (in case $F_\textrm{reac}$ is caused from surrounding continuum material) right?
I understand is, in this situation, the object's stiffness reacts to force in x-direction only ($F_\textrm{ext}$ and $F_\textrm{couple}$), not the force in z-direction ($F_\textrm{com}$ and $F_\textrm{comneg}$). Therefore $F_\textrm{reac} =-F_\textrm{com}$. Is this right?