1
$\begingroup$

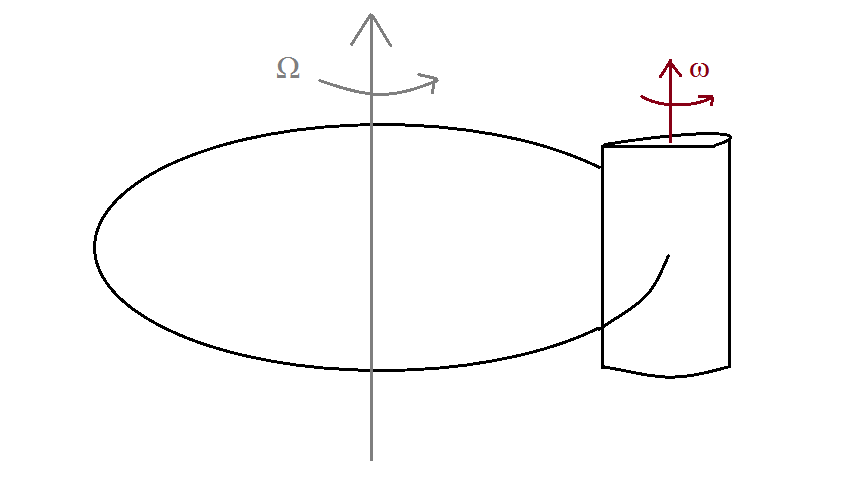
Consider a cylinder that rotates about a vertical fixed axis with angular velocity $\vec{\Omega}$ while rotating about a vertical axis passing through its center of mass with angular velocity $\vec{\omega}$.

enter image description here

Now the two angular velocities of rotation $\omega$ and $\Omega$ are not the same I suppose and there is no link between the two angular velocities, is that right?

Firstly what is the angular velocity of the rigid body? I suppose that it is

$$\vec{\omega^{*}}=\vec{\omega}+\vec{\Omega}$$

But why?

And then what is the moment of inertia with respect to the axis $z$ of the rigid body? If I use parallel axis theorem I get

$$I_{z}=I_{cm}+mR^2$$

Where $R$ is the distance from $z$. But that doesn't seem right. The cylinder is not rotating about its center of mass at the same angular velocity of rotation about $z$. So using $(1)$ give some incorrect results. For istance kinetic energy

$$K=\frac{1}{2} I_{z} \vec{\Omega}^2= \frac{1}{2}(I_{cm}+mR^2)\vec{\Omega}^2$$

But that's the kinetic energy of a body rotating about $z$ and center of mass with the same angular velocity $\vec{\Omega}$, and this is not the case.

I'm confused, can anyone help me with give suggestion about angular velocity and moment of inertia in problems where the rigid body is rotating at the same time about two different axes?

Improve this question
$\endgroup$
1
  • $\begingroup$ Is $\omega$ the joint velocity (relative) or the absolute velocity of the cylinder? $\endgroup$
    – John Alexiou
    Commented Apr 20, 2016 at 14:43

3 Answers 3

Reset to default
2
$\begingroup$

It has already been somewhat answered in this other question of yours: Parallel axis theorem and Koenig theorem for angular momentum (see also my comment to the answer).

  1. Angular velocity, like translational velocity, has to be defined by reference to a frame. In your question, you state the angular velocity about the principal axis is $ω$. So its angular velocity is... $ω$. If you study the motion in the (non galilean) frame centered on the CM and having an axis pointing inwards to $z$, the angular velocity will be $ω-Ω$. This is reminiscent of the difference between stellar day and solar day.

  2. Moment of inertia is used for rotation around a point or axis. $I_z$ is as you say; but this would be of use only if the body were rotating around $z$. Which is not the case. The movement here is a combination of:

    • rotation about the principal axis,
    • circular translation (called revolution in astronomy) about $z$.

  3. To compute angular momentum or kinetic energy in combined motions like this one, use König's theorems.

Improve this answer
$\endgroup$
1
$\begingroup$

You can look at this situation in two ways. First in terms of absolute motions $\Omega$ and $\omega$, and secondly it terms of the relative motion $\dot{q} = \omega - \Omega$. The results are the same

  1. The cylinder is spinning with $\omega$ speed and translating with $\Omega R$ speed. The combined momentum and kinetic energy is

    • Linear Momentum: $$p = m R \Omega$$
    • Angular Momentum about CM: $$L = I_{cm} \omega$$
    • Angular Momentum about Z: $$L_z = L + R \times p = I_{cm} \omega + m R^2 \Omega$$
    • Kinetic Energy $$K = \frac{1}{2} m (R \Omega)^2 + \frac{1}{2} I_{cm} (\omega)^2$$

  2. The cylinder is spinning with relative speed $\dot{q}$ and translates with $\Omega R$ speed. The combined momentum and kinetic energy is

    • Linear Momentum: $$p = m R \Omega$$
    • Angular Momentum about CM:: $$L = I_{cm} (\Omega+\dot{q})$$
    • Angular Momentum about Z: $$L_z = L + R \times p =(I_{cm} + m R^2) \Omega + I_{cm} \dot{q}$$
    • Kinetic Energy $$K = \frac{1}{2} m (R \Omega)^2 + \frac{1}{2} I_{cm} (\Omega+\dot{q})^2\\ = \frac{1}{2} \left( (I_{cm}+m R^2) \Omega^2 + I_{cm} (\omega^2-\Omega^2)\right)$$
Improve this answer
$\endgroup$
3
  • $\begingroup$ Beware that your "2." still describes the movement in the "fixed" frame of reference, not the "revoluting" one, and that's why $p$, $L$, $L_z$, $K$ have the same value in your "1." and "2." $\endgroup$
    – L. Levrel
    Commented Apr 20, 2016 at 20:19
  • $\begingroup$ Equation on a non-inertial frame do not make sense. I always look at things on inertial frames, just at different locations or with different modeling choices (absolute vs. relative motion). $\endgroup$
    – John Alexiou
    Commented Apr 20, 2016 at 20:41
  • $\begingroup$ Saying this "does not make sense" is a bit exagerated. I agree that inertial frames are generally preferable, but sometimes it's just easier to study in the non-inertial one with additional pseudo-forces: e.g. you're not going to study ballistics in the geocentric frame! $\endgroup$
    – L. Levrel
    Commented Apr 21, 2016 at 8:03
0
$\begingroup$

Yes.. The two angular velocities are independent of each other...

It's basically like earth rotating around the Sun... Earth's speed of revolution around the Sun is independent of its speed of rotation about it's own axis....

I'm not very sure about your first equation where you add the two angular velocities...

As Leverl said, the two motions are completely different... You cannot define some "COMMON" moment of inertia...

And yeah, use Konig's theorems to solve the other two questions...

Hope this helps!!!

Regards,

Pradyoth Shandilya

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.