0
$\begingroup$

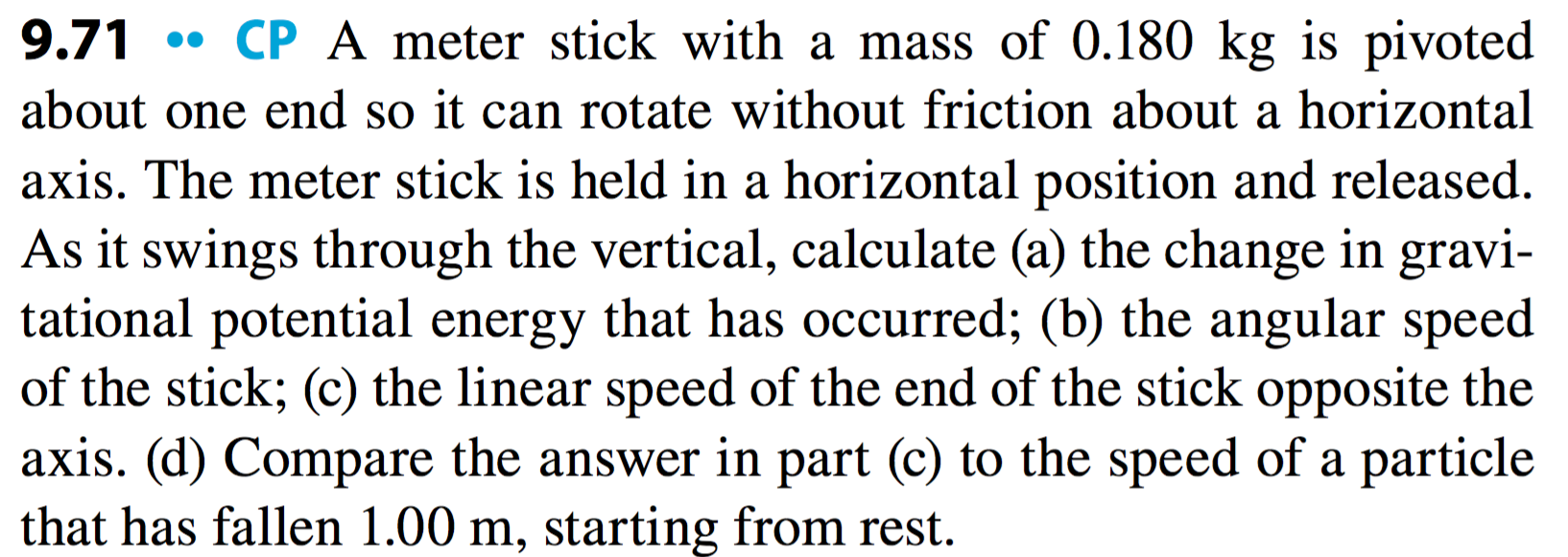
Full question:

enter image description here

My attempt:

So I tried $KE = \frac{1}{2}I\omega^2$.

Since this was a meter stick, I calculated $I = \frac{1}{3}mL^2 = \frac{1}{3}(.180 kg)(1m^2) = .006\;kg m^2$

Then I attempted to find $\omega$ by doing $\omega_f^2 = \omega_i^2 + 2\alpha\theta$ and did $\omega_f = \sqrt{2 (9.8) (\frac{\pi}{2})} = 5.54 rad/s$. Now, I calculate kinetic energy by doing,

$KE = \frac{1}{2}(.006\; kgm^2)(5.54 rad/s)^2 = .09207 J$

I figured this was the kinetic energy when the meter stick is in the vertical position of the swing, so then gravitational potential energy gets converted to kinetic energy, thus this should be the answer to a).

However, the answer to this problem is:

enter image description here

Alternatively, I tried calculating gravitational potential energy to be equal to $mgh$, so $ (.180 kg * 9.8 m/s^2 * 1 m) = 1.764 J$, and subtracting previous result gets 1.67 J, which is still not correct.

I then considered that maybe I'm suppose to use the center of mass as the mass for gravitational potential energy, so I tried (.06)(9.8)(1m) = .588 J, which is still not quite right, so now I am out of ideas.

Improve this question
$\endgroup$
3
  • $\begingroup$ Your centre of mass idea is right, but its the full mass 0.180 kg over 0.5 metres. Which is the -0.882J you need. $\endgroup$
    – Tom Andersen
    Commented Apr 10, 2016 at 19:57
  • $\begingroup$ Why is it $.5$ metres since the entire meter stick is swinging in a circle of radius 1 m? $\endgroup$
    – user40096
    Commented Apr 10, 2016 at 20:02
  • $\begingroup$ The end of the stick ends up 1 m lower. The centre of mass ends up 0.5 m lower. $\endgroup$
    – M. Enns
    Commented Apr 10, 2016 at 20:37

1 Answer 1

Reset to default
0
$\begingroup$

It has changed by $-0.882$J. It is $m*g*\Delta h$. $\Delta h$ is calculated from change in center of mass which is $-0.5 mts$ compared to datum level.

Improve this answer
$\endgroup$
5
  • $\begingroup$ why is the center of mass $-0.5 mts$ and what is $ts$ here? $\endgroup$
    – user40096
    Commented Apr 10, 2016 at 20:00
  • $\begingroup$ Consider the horizontal position COM and the vertical one. The change in height is negative and PE decreases and mts is metres. $\endgroup$
    – KakaS
    Commented Apr 10, 2016 at 20:14
  • $\begingroup$ When the meter stick is held out horizontally, the y-coordinate of the center of mass relative to one end of the meter stick is $1m$ and the x-coordinate is $.5 m$. In the vertical position, the y center of mass is $.5m$ and x-coordinate of the center of mass is $0m$. Ok, so i can see how the y center of mass changes by .5, then it would (9.8)(.180)(1m) - (9.8)(.180)(.5) = .882 J. Is this reasoning correct? $\endgroup$
    – user40096
    Commented Apr 10, 2016 at 20:19
  • $\begingroup$ Also, why is the velocity 5.42 rad/s and not 5.54 rad/s like I calculated? $\endgroup$
    – user40096
    Commented Apr 10, 2016 at 20:28
  • $\begingroup$ Yes, the coordinates system is perspective based. But remember the height is changed by 0.5 from initial to final, no matter what. So mg*(change in height) is change in PE. $\endgroup$
    – KakaS
    Commented Apr 10, 2016 at 20:33

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.