According to Wikipedia's description of torsion springs and according to my understanding of physics the energy of a torsional spring can be written as $$U=\frac{1}{2}k \varphi^2$$ where $k$ is a constant with units of $\rm N\,m/rad$.
I am freaking here because if the energy of a torsional spring is really $k \varphi^2$ than the units are $\rm (N\,m/rad) \cdot rad^2=Joule\cdot rad$. ??
What on earth am I missing here?
Radians are a pure number, so they do not contribute to your dimension considerations.
The units of the torsion constant are $\mathrm{Nm}$ which are equivalent to Joules.
You are correct that, in terms of units, $k$ should be [E]/rad$^2$.
So why is it given as [E]/rad? Sloppiness/convenience.
The unit [E]/rad is equivalent both in dimension and in value to [E]/rad$^2$, so it probably makes life easier for engineers to pretend that $k$ is the same for both the torque $\tau=k\theta$ and the energy $U=\frac{1}{2}k\theta^2$. But they are not, which is something you would have to keep in mind when converting from radians to degrees.
Another motivation for this sloppiness is that it's presumably an attempt to emulate the standard harmonic spring $f=kx$ and $U=\frac{1}{2}kx^2$, for which the $k$ values are indeed equal.
An angle is just the ratio of the length of a circular arc to its radius, so the radian has units of length/length, which means it's a dimensionless quantity.
In the SI system of units, the radian is a special name for 1 (see SI brochure), that is, $$\mathrm{rad}=1.$$
Therefore, $$[k] = \mathrm{N\,m/rad} = \mathrm{N\,m}$$ and $$\mathrm{J\,rad} = \mathrm{J}.$$
Since the last revision of the SI, the radian is no longer a supplementary unit: an angle is now defined as the ratio of two lengths, and the unit radian is now maintained for convenience. However, it's just a synonym of 1, and can be used (but it's not necessary) to convey, or to strengthen, the information that the quantity of interest is an angle.
Let's see how the units work out if we convert a linear spring (where we know everything) to a torsion spring, by attaching our linear spring to a stiff rod some distance $R$ from a pivot:
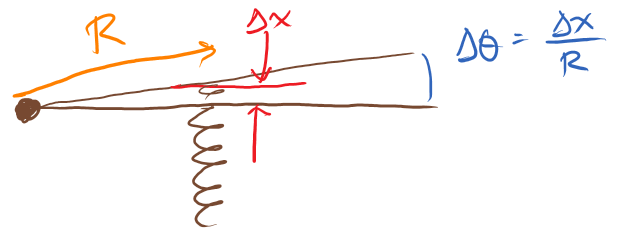
The (linear) force due to the spring is $\vec F = -k\Delta \vec x$, for spring constant $k$ having units of newtons per meter. The torque is $$ \tau = RF = -R \cdot k (R \Delta\theta) \equiv -\kappa \Delta\theta $$ So apparently the torsion spring constant $\kappa = kR^2$ has units of newton-meters, which is equivalent to newton-meters per radian, because the radian is a dimensionless ratio. If you mistrust the apparatus of calculus and would like to do a lot more work you could use the appropriate trig function $\sin\Delta\theta = \Delta x /R$; in that case only follow my argument in the small-angle approximation $\lim_{|x|\ll1} \sin x = x$.
The energy stored in the linear spring is \begin{align} U = -\int_0^{\Delta x} \vec F \cdot d\vec x = \frac12 k(\Delta x)^2 = \frac12 k(R\Delta\theta)^2 = \frac12 \kappa (\Delta\theta)^2 \end{align} which is exactly the same thing you get if you integrate the torque $$ U = -\int_0^{\Delta\theta} \vec\tau \cdot d\vec\theta = \frac12 \kappa (\Delta\theta)^2 $$ As another answer says much more succinctly: it all works because the radian, a ratio between two lengths, is dimensionless.
lemon asks in a comment elsewhere how you would convert $\kappa$ in each case if you were tied to a log in a sawmill and commanded either to use degrees or die a hideous bloody death. (My paraphrase; I would probably choose death, myself.) In that case you might grudgingly admit that the relevant constant in the torque equation has units of foot-pounds per degree, while in the energy equation you have picked up another angular factor so that the unit is b.t.u. per degree squared. I don't think there's anything profound about the coincidence that torque has units of energy; I do think there's something profound about the fact that we have invented the SI units to make these pointless problems go away.
