Problem with variation of velocity, doubt on the work energy theorem

Question

I think I did not understand the use of the work energy theorem. Here is a situation I am confused about.

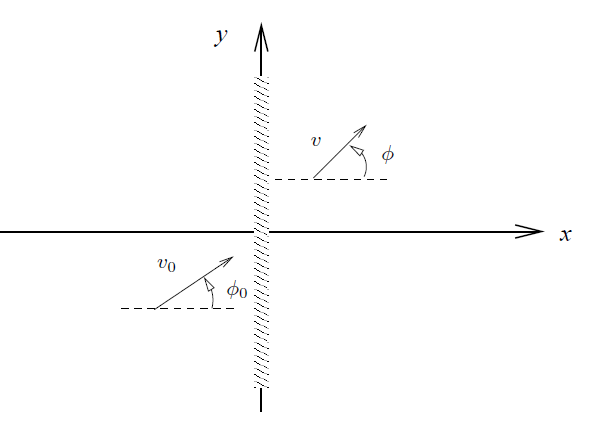
Consider an object moving in the $xy$ plane with a constant velocity $\vec{v_0}$ at an angle $\phi_0$, with no forces present at all. There is a region around the $y$ axis where there is a force $f$ that depends only on the $x$ coordinate, not on the $y$ coordinate or time. The force is always parallel to the $x$ axis ($f_y=0$). After the object passes through the region it has a (constant) velocity $\vec{v}$ at an angle $\phi$.

If $\mid\vec{v_0}\mid$, $\mid\vec{v}\mid$ and $\phi_0$ are given, how to determine $\phi$?

I can use the work energy theorem to determine the amount of work done by the force $W=\Delta K$. Nevertheless I can't see how to use it for a variation of the angle, since in that case the perpendicular component of the force (no work done) counts. So maybe that's not the way, but I don't know anything about the force, except that it is not constant, the amount of work done and the orientation, I don't see where to get information from.

Does anyone have suggestion or hints for this problem?

Answer 1

Decompose the initial velocity as $v_x = v_0*cos(\phi _0)$, $v_y = v_0*sin(\phi _0)$. Use these to calculate the initial kinetic energy.

Since the force does not act in the y direction, $v_y$ remains unchanged, but $v_x = v*cos(\phi )$ when the particle exits the force field, which is given in the problem statement.

So use these values to calculate the final kinetic energy; clearly the $v_y$ portion cancels, leaving the integral applied force in the x direction to be equal to difference in the remaining terms.

Answer 2

Well I see the question is about determining $\phi$, so you have nothing to do with the work energy theorem. Just use the conservation of the vertical component of velocity:

$v_0\sin(\phi_0)=v\sin(\phi)$

and solve the equation for $\phi$.