2
$\begingroup$

Consider a simple loop like so:

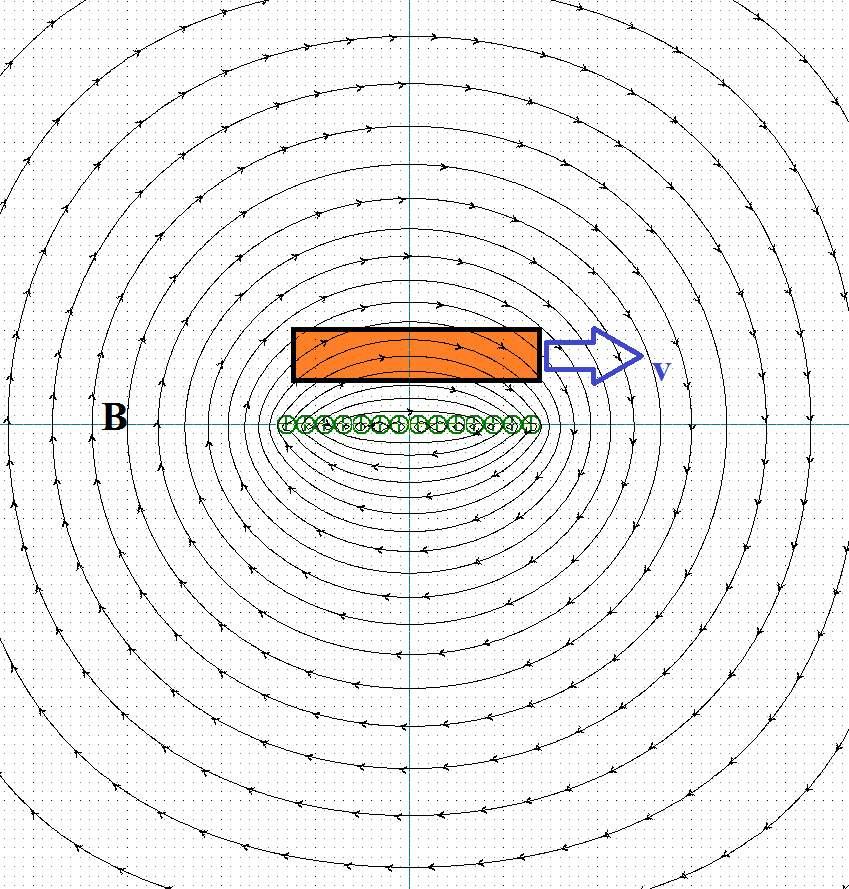
enter image description here

And in-front of this loop are a series of wires that would cover the whole are of the loop, these wires are parallel to one another, and they have current flowing downwards within them creating a uniform magnetic field, if this loop of wire is placed in-front of the wires, and moved to the right would there be an induced EMF?

Top view: enter image description here

What's confusing for me is that the magnetic field is entering the conductor on the left side, and also exiting it on the right side, how would the net magnetic field $B$ be with respect to the loop? For an induced EMF. Also, you could say that the velocity is "somewhat" parallel to the field lines, but not sure...

Improve this question
$\endgroup$
4
  • 2
    $\begingroup$ Not quite sure I understand the geometry you are proposing, but if the loop is parallel to the wires, then the flux trough the loop changes, so there is an induced emf and a current will flow. $\endgroup$
    – CuriousOne
    Commented Mar 1, 2016 at 3:40
  • $\begingroup$ Yes, the geometry isn't clear. Do any field lines cut the loop or is the loop lying on the plane of the your computer screen? (Sorry, no idea what could be used in place of computer screen) $\endgroup$
    – Yashas
    Commented Mar 1, 2016 at 4:47
  • $\begingroup$ It appears as if the food through the vertical cross section of the loop is changing from the diagram. Is that the case? $\endgroup$
    – Tamoghna Chowdhury
    Commented Mar 1, 2016 at 5:36
  • $\begingroup$ @CuriousOne Switching the position of the conductor to be behind of the wires would that change polarity of the induced EMF? Or it would be the same, due to the odd geometry, it's confusing to state so $\endgroup$
    – Pupil
    Commented Mar 5, 2016 at 1:10

1 Answer 1

Reset to default
2
$\begingroup$

Yes, there is an EMF. But it is not an induced EMF, it is a motional EMF.

Let's look at the top down picture. The left side of the picture has a magnetic force pushing upwards. The right side of the picture has a magnetic force pushing downwards. And in the middle you have the exact top and the exact bottom and there is no magnetic force there because the field and the velocity are exactly in the same direction there.

Thus when we compute the total EMF:

$$\mathscr E=\oint \left(\vec E+\vec v\cdot\vec B\right)\cdot \mathrm d\vec \ell$$ we get the magnetic part $\oint \left(\vec v\cdot\vec B\right)\cdot \mathrm d\vec \ell$ is non zero. The electric part of the EMF is related to Faraday's Law:

$$\oint \vec E\cdot \mathrm d\vec \ell=-\iint \frac{\partial \vec B}{\partial t}\cdot \hat n\, \mathrm d A.$$

And, when

  1. the wire is thin (so it should look like a horizontal line when viewed from above) and
  2. there are no magnetic monopoles, and
  3. the charges are confined to stay in the wires (e.g. by a Hall voltage)

then all three together give us:

$$ \oint \left(\vec v\cdot\vec B\right)\cdot \mathrm d\vec \ell= \iint \frac{\partial \vec B}{\partial t}\cdot \hat n\, \mathrm d A-\frac{\mathrm d}{\mathrm d t}\iint \vec B\cdot \hat n\,\mathrm d A.$$

That, plus Faraday gives us the universal flux law:

$$\oint \left(\vec E+\vec v\cdot\vec B\right)\cdot \mathrm d\vec \ell=-\frac{\mathrm d}{\mathrm d t}\iint \vec B\cdot \hat n\,\mathrm d A.$$

If you want to relate your EMF to the universal flux law note that while the flux is zero in the position you drew. It is about to be nonzero as soon as you move a bit to the right. So the time rate of change is nonzero. It's just that since the magnetic field isn't changing in time, the EMF is motional because it is all due to the motion of the wire in the current magnetic field.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Switching the conductor to the other side(back of the wires) would lead the same results, or the negative of both? $\endgroup$
    – Pupil
    Commented Mar 5, 2016 at 1:07
  • $\begingroup$ @XCIX Every equation I wrote would be the same: there is a sign convention that the $\hat n \mathrm d A$ points in the direction of my right thumb if the $\mathrm d\vec\ell$ points in the direction of my right fingers. And the current should go the same way. $\endgroup$
    – Timaeus
    Commented Mar 5, 2016 at 1:56

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.