Total internal reflection in a simple pane of glass - why can we see through?

Question

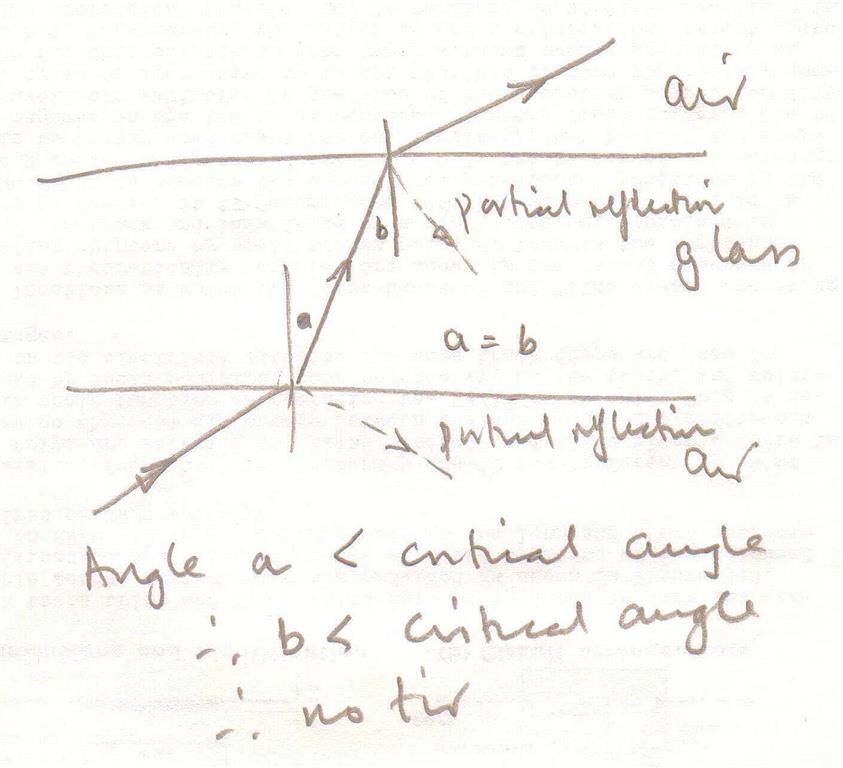
Consider looking through a glass window pane at high angles of incidence. At the front face of the pane, there is no total internal reflection since the light is traversing an air to glass interface. But at the back face, the light traverses a glass to air interface and so should undergo total internal reflection at some angle. The critical angle for glass to air is approx 42 deg, so, even accounting for refraction, why can we still see through a pane of glass at angles well beyond 42 deg?

Answer 1

The conditions for total internal reflection from an air-common glass interface are:

The light has to be travelling in the glass.
The angle of incidence has to be larger than the critical angle.

Answer 2

For a pane of glass, the two surfaces are parallel to each other. That means that the exit angle from the 2nd surface will be the same as the entrance angle of the first. Whatever light enters (and isn't absorbed) will exit: $$n_{air}\sin\theta_{1}=n_{glass}\sin\theta_{g1}$$ and $$n_g\sin\theta_{g2}= n_{air}\sin\theta_2.$$

A quick sketch of two parallel lines and a refracted ray will show you that $$\theta_{g1}=\theta_{g2}$$ for refraction angle at the first surface and the incident angle at the second surface inside the glass. That means $$\sin\theta_{1}=\sin\theta_{2}$$ and light which enters the glass on one side will never reach the critical angle inside the glass.