Do we know WHY there is a speed limit in our universe?
Your question is similar to:
"Do we know WHY there is a length limit?"
The same way we need finite lengths to measure size or interval between two points in 3D - Euclidean space, we need a finite speed of light to measure the interval between events in 4D - Minkowski space. Minkowski developed his theory to expand Maxwell's equation in four dimensions. In order for $s^2$ in Minkowski space, (2), to preserve its invariance, as an extension of the Pythagoras' Theorem, (1), which in three dimensions is:
$$
s^2 = x^2 + y^2 + z^2 \tag{1}
\,,$$
and in four dimensions becomes:
$$
s^2 = x^2 + y^2 + z^2 - {\left(ct\right)}^2 \tag{2}
\,,$$
$c$ needs to be not only finite but same for all reference frames, which is supported by the observed evidence that light (EM waves) speed was independent of observes's frame of reference.
The speed of light needs to have a limit, i.e. to be finite for the Special Relativity to work:
If you go back to the famous A. Einstein's thought experiments, specifically the one where there are two observers, one not moving, $A$, standing on a train station and another, $B$, moving standing in a train, that passes through the train station.
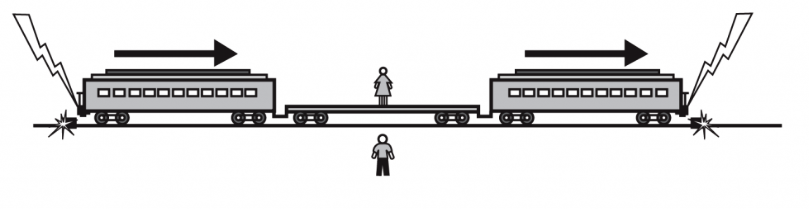
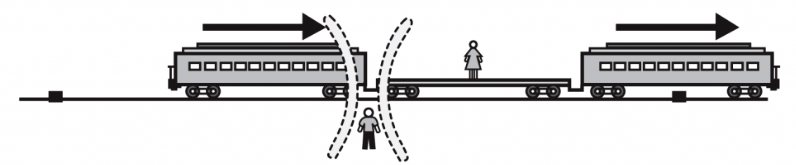
Now, when the train passes and $A$ and $B$ are right opposite each other, lightning strikes on both sides of $A$, at the same distance. $A$ sees them simultaneously:
However, because $B$ is moving relative to them, i.e. away from one and towards the other it, sees them successively:
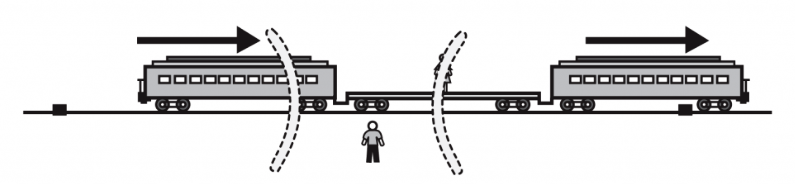
...right?
Well, NO, this would have resulted in the light being measured differently in different reference frames, something that was refuted by Michelson–Morley experiment that used Earth as the train:
$\hspace{100px}$ ,
,
and measured light speed in two perpendicular directions:
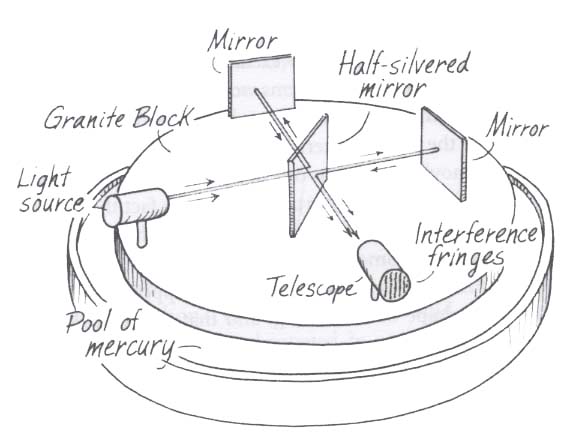
assuming that the light moving in the direction matching Earth's direction of movement would have to be smaller (similar to the person, $B$, who was on the train) than the other, assumption that was scientifically proved to be wrong.
Consequently, the speed of light is constant and anyone measuring it will find the same value, regardless of his speed or in other words speed of light is invariant. Invariance could be contrasted with relativity, for example, relativity of time, which by the way was used to describe why the two people, $A$ and $B$, observe the same speed of light, namely because the time of the moving person $B$ is ticking slower, in general, the larger the relative velocity between the two observers, the greater the difference of the ticking rate of their watches, i.e. time dilation.
Finally, time dilation could be observed in the presence of an object with mass that generates a gravitational field, or in terms of General Relativity in stretched space-time, that will cause the time of the observer located closer to the mass object to tick at a slower rate, i.e. time dilation and respectively, the observer located at a greater distance will observe his watch to tick with faster rate.
As you see time is relative, space stretches and speed of light is the constant, with a finite value that "holds them together" and "synchronizes them", defining event simultaneity. Moreover, with its help, we can define an invariant interval between two points in space-time, i.e. between two events. Space-time intervals depend on the temporal and spatial separations of the two points and they could be: time-like, light-like(time distance = space distance) or space-like(time distance < space distance). Thanks to that Special Relativity is a successful theory, with a long list of supporting experiment evidence.
Edit:
As a response to the first comment, which argues that time dilation is a result of the constancy of light speed, I will present you an example of the opposite, i.e. constancy of light speed can be shown as a direct consequence of velocity time dilation:
Let us consider a hypothetical clock called photon clock. In it, light is reflected back and forth between its mirrors and whenever the light strikes a given mirror,
the clock ticks once. If this clock is in inertial motion with respect to
an observer, then velocity time dilation will cause it, like all other kinds of
clocks, to tick slower. However, because the clock is moving, the light pulse
will trace out a longer, angled path between the mirrors. The net result of
velocity time dilation and increase in the path length is that the speed of
light in the moving photon clock remains equal to the speed of light in the
rest photon clock. In other words, the speed of light remains constant.
Moreover, Lorentz transformation (LT), which were derived by Joseph Larmor [1]
in 1897, and Lorentz (1899, 1904) [2], directly predicted time dilation. In fact, time dilation by the Lorentz factor was correctly predicted by Joseph Larmor (1897)[3] long before Einstein published his paper in 1905.
Your question tends to be a bit Philosophical, for all we know the value of $c$ could be related with a property of space-time itself, determined along with the other Fundamental Physical Constants during the Big Bang, which is difficult to observe and realize similar to why fish is not aware of all the water around it.
[1] Larmor, J. (1897), “Upon a dynamical theory of the electric and luminiferous medium”, Philosophical Transactions of the Royal Society
190: 205-300.
[2] Lorentz, Hendrik Antoon (1899), “Simplified theory of electrical and optical phenomena in moving systems”, Proc. Acad. Science Amsterdam I: 427-443; and Lorentz, Hendrik Antoon (1904), “Electromagnetic phenomena in a system moving with any velocity less than that of light”,
Proc. Acad. Science Amsterdam IV: 669-678.
[3] Larmor, J. (1897), “On a Dynamical Theory of the Electric and Luminiferous Medium, Part 3, Relations with material media”, Phil. Trans.Roy. Soc. 190: 205-300