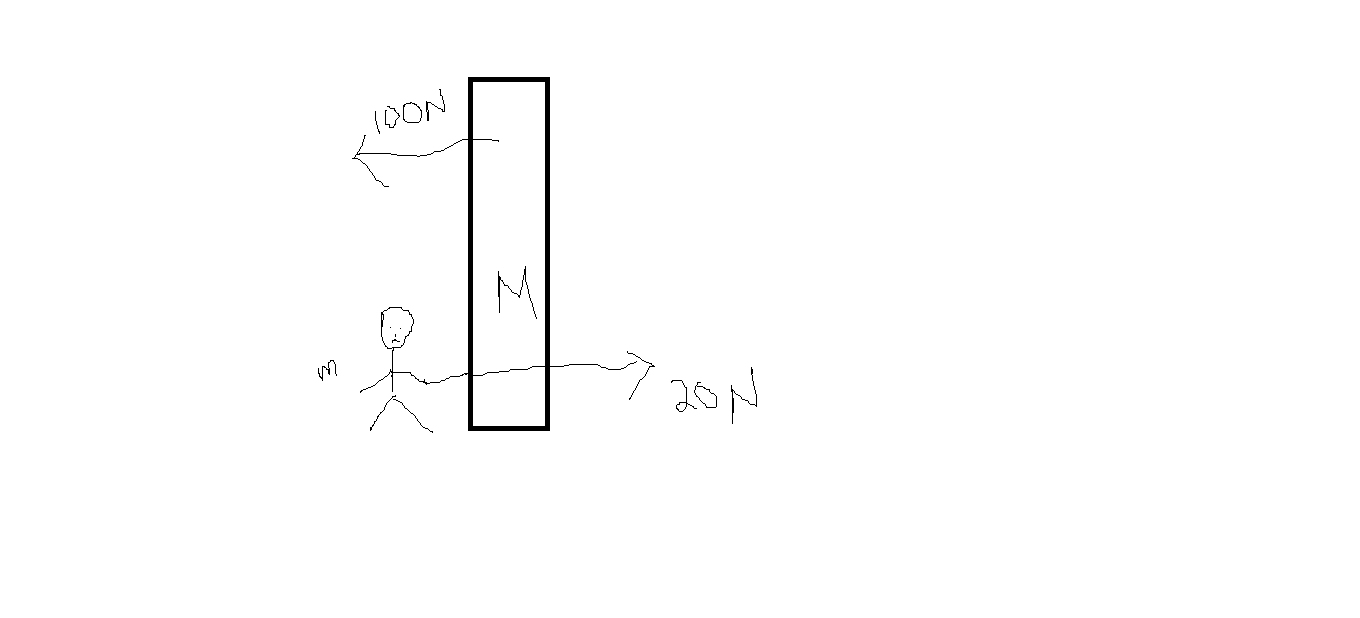
consider the following situation shown in the diagram below.A huge wall of mass M is moving to the left due to a constant force of 100N. Now suppose a man of mass m applies a force of 20N to the right.the man and the wall will move to the left with an acceleration of
(100-20)/(m+M) to the left. therefore the net force acting on the man is 80m/(M+m) which is being applied by the wall.Now since the man is applying a force of 20N on the wall according to newton's third law the wall should apply an equal force of 20N on the man. therefore we can say that 80m/(m+M)=20, however if we arbitrarily choose a random value for m and M the two values don't match..where am i going wrong?
$\begingroup$
$\endgroup$
2
asked Dec 30, 2015 at 12:01
-
$\begingroup$ Well in your very first calculation ,net force is 100N rather than 100-20N $\endgroup$– Sahil ChadhaCommented Dec 30, 2015 at 12:27
-
4$\begingroup$ Note: in English, sentences end with a period ".", and there is always a space after the period before starting the next sentence. Also, each new sentence starts with a capital letter, and the word "I" is always capitalized. Finally, this site supports mathjax. $\endgroup$– DanielSankCommented Dec 30, 2015 at 13:03
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
Look it this ways Let's say that a man with mass m is applying no force and is at rest, and initially there is only force acting on wall of mass M which is 100N and the two masses are not in contact with eachother, As the wall comes in contact with man they both start to move, with acceleration $a=\frac{100}{M+m}$, the force acting on the man is $F_m=ma$ and this force comes from the force which wall of Mass $M$ applies only on the man and than the total resultant force acting on the wall will be $F_w=100-F_m$ where here $F_m$ is reaction force which man is applying on the wall and as we can see from the total form of this force $F_m=\frac{100m}{M+m}$, the action-reaction pair of force will depend on both the masses and the forces which acting on masses, here man was initially applying no force before contact
Now, take your case a force of 20N is acting on man which in turns he apply on the wall total force acting system $80N$ and the system is moving with acceleration $a=\frac{80}{M+m}$
The best way to check how much is action-reaction force between man and wall is to solve, Force equation on both mass
- The resultant force acting on wall is $100-F_{r}=\frac{100M}{m+M}$
- The resultant force acting on man is $$F_r-20=\frac{80m}{M+m}$$ Now we solve both equation for $F_r$ $$F_r=60-40\frac{M-m}{M+m}$$ and we can see that it is not $20N$ but depend on both the masses and the forces acting on both masses
$\begingroup$
$\endgroup$
The acceleration of the system is determined by the sum of external forces. If the man is pushing against the floor with a force of 20N and some invisible hand is pushing against the wall with a force of 100 N, it does not follow that the force between man and wall is 20 N (or 100 N).
The correct analysis involves a diagram showing the two external forces and the force $F$ between man and wall. The acceleration of the man is due to net force $F-20$, and the acceleration of the wall is due to $100-F$. If those result in the same acceleration you can solve for $F$ with
$$\frac{(F-20)}{m}=\frac{(100-F)}{M}$$
And with $F$ found, $a$ follows.
$\begingroup$
$\endgroup$
You have over specified the problem. Either the motion of the man equals the motion of the wall and the force between them is unknown, or the force is known and the motion is unknown.
If the force applied by the man is $F$ then either
$$ \begin{cases} F = m a_{wall} & \mbox{man moves with wall with reaction}F \\ a_{man} = \frac{F}{m} \neq a_{wall} & \mbox{man applies force }F \end{cases}$$
answered Dec 30, 2015 at 14:58