Let's say we've got a fluid of heavy and light particles inside a cubical flask, which is initially shaken up so that the density of heavy particles is uniform everywhere. Let's also say that these molecules interact identically whether in contact with like or opposite particles (i.e. they don't naturally separate, like water and oil, and they don't naturally mix, like soap and dirt). Over time, the heavier particles will settle at the bottom of the flask. This seems like a decrease in overall entropy to me. However, since potential energy was unleashed when sinking in the flask, it still isn't obvious that this violates any fundamental principle.
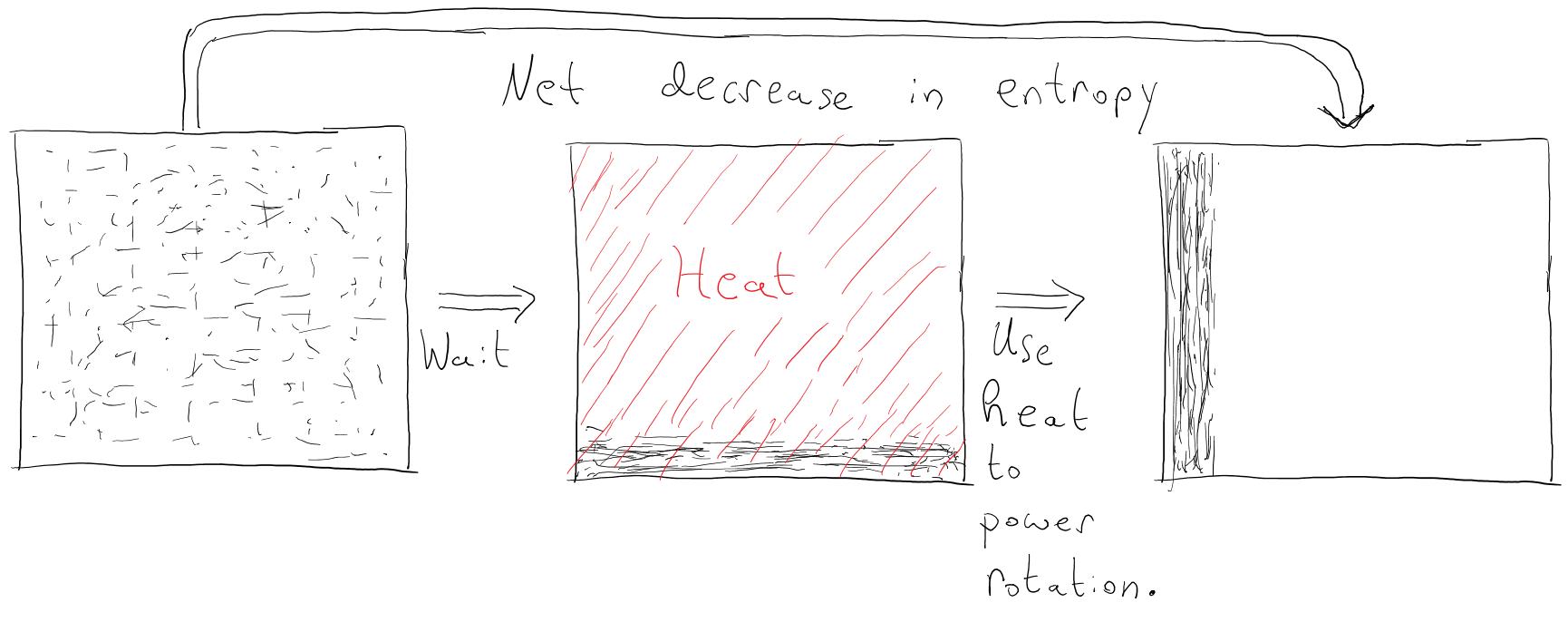
So, my next thought is whether or not there is a way to re-capture the heat dispersed while the particles decrease their potential energy through settling, and then use that heat to rotate the cube 90 degrees so that the particles will be level with where they started, which would use up the exact same amount of energy, since their center of mass would be at the same height:
(Aside: the particles would only remain on the left edge of the container for a moment - then they would again start settling back down to the bottom.) Since we want to re-capture as much energy as possible, let's use a Carnot engine. We'll situate our cube in a somewhat large, cold container. A thermally isolating barrier will be erected between the cube and the container, and only a Carnot engine will connect the two. We wait for the particles in the cube to settle down, hence giving off heat, which is initially trapped in the cube. We then turn on the engine, and extract a fraction $ \eta $ of the heat dispersed by the settling particles. The formula for $ \eta $ in a general Carnot engine is:
$ \eta = \frac{T_{H}^{*} - T_{C}}{T_{H}^{*}} = 1 - \frac{T_{C}}{T_{H}^{*}} $
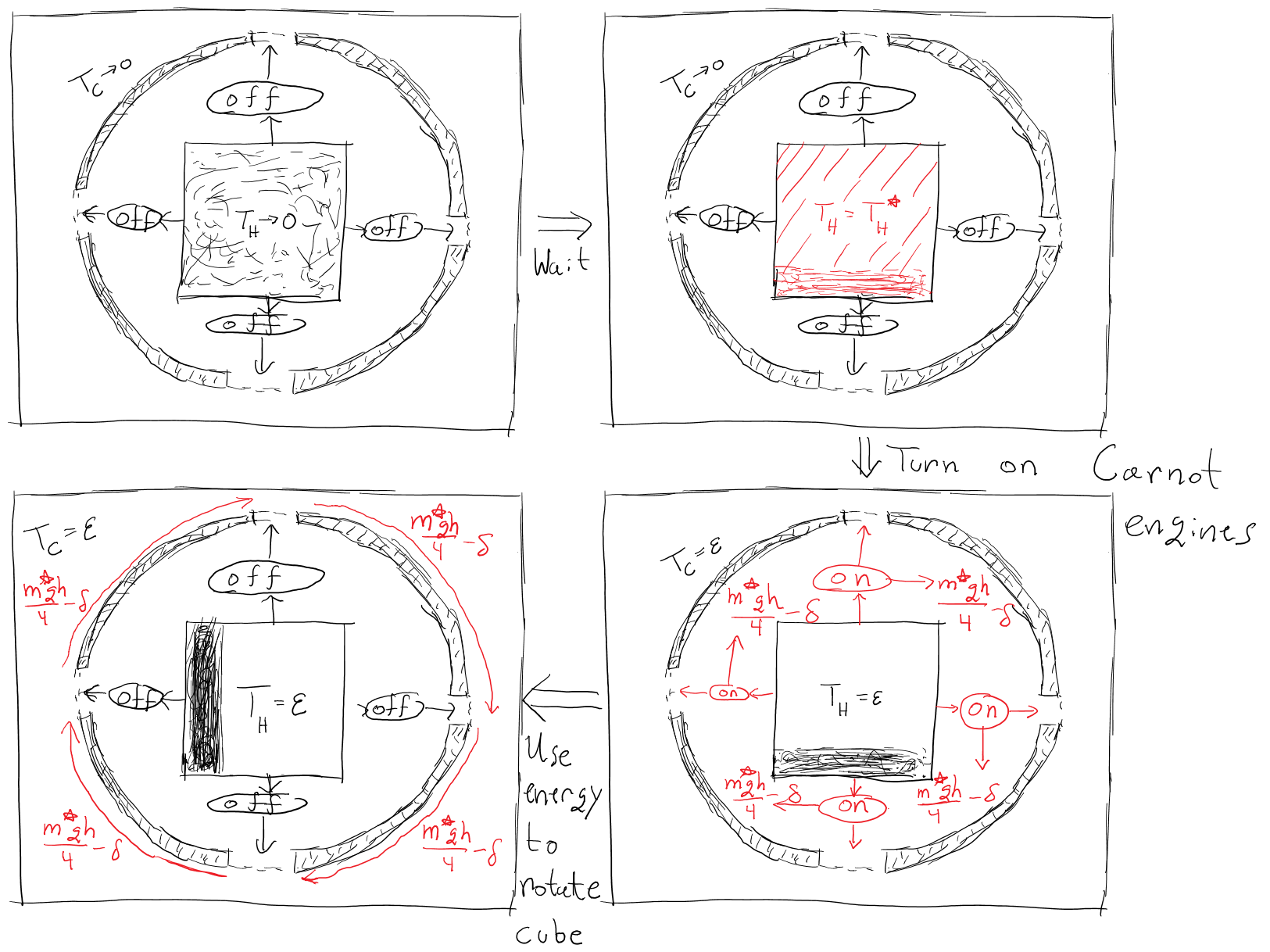
Where $ T_{H}^{*} $ is the heat of the cube after settling and $ T_{C} $ is the temperature of the outside fluid, which is assumed to be the same as the temperature of the cube at the start of the experiment. How good can $ \eta $ get? While it would be tricky/impossible to get $ T_{H}^{*} $ arbitrarily large, it wouldn't be too hard to get $ T_{C} $ arbitrarily small. If we take the limit as $ T_{C} $ goes to absolute zero, we'll get infinitesimally close to $ 100\% $ of our energy back! It doesn't matter that $ \eta $ isn't exactly $ 100\% $ - an infinitesimal loss of energy won't explain the finite drop in entropy. We can have, say, a very tiny spring handy to push the cube the last little bit. A diagram of how this machine would work might look like:
Where:
- The circular material is the friction-less insulator that separates the cube from the surrounding fluid.
- The square is our cubical flask.
- The dots are the heavier particles (I haven't drawn the lighter particles they displace)
- The four circles connecting the cube with the outside fluid are four Carnot engines. They are initially off. There are four so that the whole rotating chamber (everything inside the circular insulator) is equally balanced, except for the particles.
- red illustrates where (all but infinitesimal leaks of) the energy goes.
- $ \epsilon $ is the temperature of the cube and the surrounding liquid after the Carnot engines extract the heat from the inner cube. $ \eta $ isn't exactly $ 100 \% $, and hence some small energy will remain untapped.
- $ 4 \delta $ is the amount of energy not extracted.
- $ m^{*} $ is the mass of one of the heavier particles minus one of the lighter particles.
- $ g $ is the acceleration due to gravity.
- $ h $ is the average of the distances the particles fall - that is, half the side length of the cube.
(Thus, between these last three points, $ m^{*} g h $ is the amount of energy unleashed as the particles fall)
- The mechanism used to convert $ m^{*} g h $ energy into the rotation of the chamber isn't shown, but should be pretty basic.
To me, it seems that this machine finitely decreases entropy at an infinitesimal loss of energy. What's gone wrong with my reasoning? Any clarification will be greatly appreciated!