How the nucleon structure has been identified experimentally?

Question

It is known that nucleons (proton, neutron) are composed of partons (quarks, etc.). How was this identified experimentally? In particular, how it has been identified that nucleons comprise of more than one constituent?

Answer 1

As you may know, when particles are scattered off a target, what actually gets measured is the differential cross section $\frac{\mathrm{d}\sigma}{\mathrm{d}\Omega}$. This can basically be thought of as being related to the fraction of particles that come out of the collision in a particular direction. It's possible to calculate this quantity using quantum field theory, and we can then compare the results to the assumptions that go into that calculation.

For the proton specifically, we express the differential cross section in terms of parton distribution functions (PDFs). Each of these functions, written $f_{i/\text{p}}(x, Q^2)$, gives something akin to the probability that a collision will involve a parton (quark or gluon) of type $i$ with a fraction $x$ of the proton's momentum, when the colliding particle has squared transverse momentum $Q^2$. So the overall cross section for, say, an electron hitting a proton has the basic structure

$$\frac{\mathrm{d}\sigma_{\mathrm{ep}}}{\mathrm{d}\Omega} \sim \sum_{i}\int\mathrm{d}x\,f_{i/\text{p}}(x, Q^2)\frac{\mathrm{d}\hat\sigma_{\mathrm{e}i}}{\mathrm{d}\Omega}$$

In words, for each type of parton, you multiply the probability of finding that particular kind of parton with the cross section $\frac{\mathrm{d}\hat\sigma}{\mathrm{d}\Omega}$ for that particular interaction (which can be calculated from QFT). Then integrate the contributions from all possible values of $x$, and add that up for all possible parton types. That gets you the total cross section. (There's some other stuff involved which I'll leave out for simplicity.)

Now, since $\frac{\mathrm{d}\hat\sigma_{\mathrm{e}i}}{\mathrm{d}\Omega}$ can be calculated, and $\frac{\mathrm{d}\sigma_{\mathrm{ep}}}{\mathrm{d}\Omega}$ can be measured, if you do enough different kinds of collisions at different energies, you can reconstruct the PDFs. And since each PDF is related to the density of its particular type of parton within the proton, if you know them all you basically know the composition of the proton. (Specifically, the quantity $xf_{i/\mathrm{p}}$ basically tells you the momentum distribution of parton type $i$.) For example:

• Suppose $xf_{\mathrm{u/p}}$ was measured and turned out to be a sharp spike at $x=1$. That means that when something collides with a proton, it only ever interacts with an up quark that carries all the proton's momentum, and that in turn tells you that the proton contains just an up quark and nothing else.

• Suppose $xf_{\mathrm{u/p}}$ instead turned out to be a spike at $x = \frac{1}{3}$. That would mean that when something collides with a proton, the only up quarks it ever interacts with carry $\frac{1}{3}$ of the proton's momentum. This would be the case if, for example, the proton was a rigidly bound collection of 3 quarks, each carrying $\frac{1}{3}$ of the total momentum, in which case the binding particles (gluons) would carry very little of the proton's momentum.

  You would, of course, have to measure the other PDFs (for down quarks, strange quarks, etc.) to determine what, if anything else, was in the proton. This is how you'd tell the difference between compositions of, say, $\mathrm{uuu}$ and $\mathrm{uud}$: in the latter case $xf_{\mathrm{d/p}}$ would have a spike of half the size, whereas in the former case $xf_{\mathrm{d/p}}$ would be zero everywhere.

• On the other hand, suppose you find that $xf_{\mathrm{u/p}}$ is a bump. That tells you that the up quarks in the proton don't have a definite fixed momentum, but rather that they move around with varying velocities. This would be a strong indication that the proton acts kind of like a fluid, with multiple constituent particles bouncing off each other and sharing their momentum around in the process. The peak of the bump would tell you (roughly) what is the most likely momentum fraction for the up quarks to have.

  Again, you would have to measure the other PDFs to figure out what other kinds of particles are in this "fluid," and in what proportions - for example, if there are twice as many up quarks as down quarks, you would find that the bump in $xf_{\mathrm{u/p}}$ is twice the height of $xf_{\mathrm{d/p}}$.

It turns out (perhaps unsurprisingly) that this last case is most relevant for a real proton. Now, I couldn't find the picture I really wanted to illustrate this, but I did get to make the following graph using polarized structure function data from SLAC-143, and it makes a similar point.

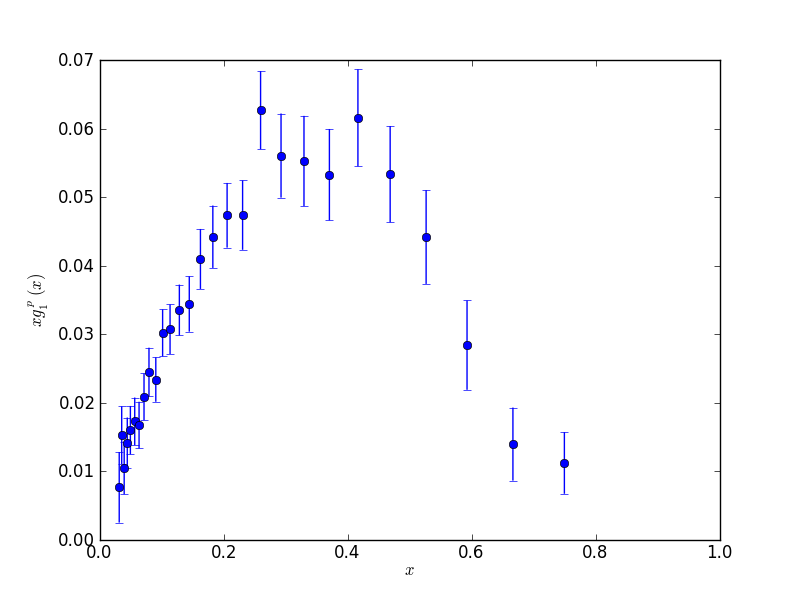
You can see that there's a wide bump centered around $x = \frac{1}{3}$. This indicates that the partons in the proton are most likely to be carrying a third of the proton's momentum each, and this is the sense in which one might say that there are 3 constituent particles. (Technically, because this is a polarized structure function, it's talking about the difference between particles with opposite spins. But the unpolarized equivalent would look roughly similar.)

Taking into account all the data that has been collected over the last 40 years or so, the current PDFs for the proton look like this:

As you can see, this combines some of the different features I discussed above. Most notably, there's a bump around $x = \frac{1}{3}$, and it's twice as large for up quarks as for down quarks, which means that there 2 valence up quarks and 1 valence down quark. But there is also a sharp rise in all the PDFs as you go to very small $x$, which is kind of like a spike. It indicates that there are a very large number of partons carrying very small fractions of the total momentum.

Answer 2

Matt Strassler goes into detail with LHC data here:

http://profmattstrassler.com/articles-and-posts/largehadroncolliderfaq/whats-a-proton-anyway/checking-whats-inside-a-proton/