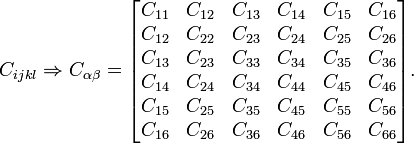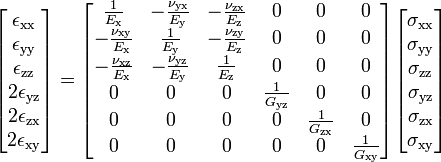Physical meaning of elastic constants of a monoclinic crystal

Question

For the elasticity of a material, Hook's law can be written in tensorial form as:

$$\sigma = \mathsf{C}\, \varepsilon$$

where $\sigma$ is the Cauchy stress tensor, $\varepsilon$ is the infinitesimal strain tensor, and $\mathsf{C}$ is the fourth-order stiffness tensor. This tensor can be reduced to an elasticity matrix (in Voigt notation) as:

(click on the image for the corresponding Wikipedia page).

Depending on symmetry of the material, this matrix can be simplified: for an isotropic system, this matrix reduces to two parameters; it has 3 independant elements for cubic symmetry. These elements have simple physical meaning, and for the general case of orthothropic materials (three orthogonal planes of symmetry), it can be expressed in terms of Young's moduli, shear moduli and Poisson ratios:

(again, click on the matrix for WP link.

---

Now the context is set, here comes the question. I deal with a crystal in the monoclinic system, whose elasticity matrix is of the form:

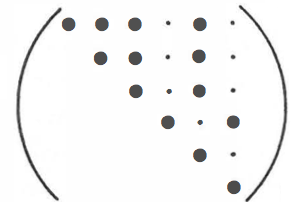
(Nye, Physical properties of crystals; only upper half of the symmetric matrix is written, small dots correspond to elements guaranteed to be equal to zero.)

I have calculated all terms of the matrix for my single crystal. How can I link them to physical properties that are easily understandable? (as I showed with Young moduli, shear moduli and Poisson ratios in the orthotropic case above)

Answer 1

I'd swap the meaning of x and y to make the sparsity structure more conspicuous (block diagonal). The I'd try to identify the paramaters as in the isotropic case, where they exist, and itnroduce new ones for the others by analogy (note that each column has the same denominator). Then consider special stress vectors that affect only few strain components, and interpret these in physical terms (do it first for the known constants so that you can see what to aim for). This gives you the meaning of the new constants.

Of course, finding out how others call them may be more difficult. On the other hand, it is usually easier to make a literature search after you have discovered something for yourself even though it is not new for the scientific community.

Answer 2

In that case what happens is that the moduli of longitudinal elasticity moduli $E_{\boldsymbol{n}}$, transverse elasticity moduli $G_{\boldsymbol{n}}$ and Poisson ratios $\nu_{\boldsymbol{n},\boldsymbol{m}}$ will depend in a complicated way on the constants $C_{AB}$ and the direction $\boldsymbol{n} = \alpha \boldsymbol{\hat{\imath}}+ \beta \boldsymbol{\hat{\jmath}}+ \gamma \boldsymbol{\hat{k}}$.

For uniaxial tensile test in direction $\boldsymbol{n}$, you have:

$$\begin{bmatrix} \sigma_x \\ \sigma_y \\ \sigma_z \\ 0 \\ 0 \\ 0 \end{bmatrix} = \varepsilon \begin{bmatrix} C_{11} & C_{12} & C_{13} & 0 & C_{15} & 0 \\ C_{21} & C_{22} & C_{23} & 0 & C_{25} & 0 \\ C_{31} & C_{32} & C_{33} & 0 & C_{35} & 0 \\ 0 & 0 & 0 & C_{44} & 0 & C_{46} \\ C_{15} & C_{25} & C_{35} & 0 & C_{55} & 0 \\ 0 & 0 & 0 & C_{44} & 0 & C_{66} \end{bmatrix} \begin{bmatrix} \alpha \\ \beta \\ \gamma \\ 0 \\ 0 \\ 0 \end{bmatrix}$$

and as a consequence the stress vector is $\boldsymbol{\sigma} = \sigma_x \boldsymbol{\hat{\imath}}+ \sigma_y \boldsymbol{\hat{\jmath}}+ \sigma_z \boldsymbol{\hat{k}}$. Then the longitudinal elasticity modulus (Young's modulus) in direction $\boldsymbol{n}$ is:

$$E_\boldsymbol{n} = \frac{\text{d}\sigma_\boldsymbol{n}}{\text{d}\varepsilon_\boldsymbol{n}} = \frac{\boldsymbol{\sigma}\cdot\boldsymbol{n}}{\varepsilon}= \frac{\sigma_x\alpha+\sigma_y\beta+\sigma_z\gamma}{\varepsilon}$$

For the Poisson's ratios, you can compute:

$$\nu_{\boldsymbol{a},\boldsymbol{n}} = -\frac{\text{d}\varepsilon_\boldsymbol{a}}{\text{d}\varepsilon_\boldsymbol{n}}$$

where $\varepsilon_\boldsymbol{a}$ is the projection of the strain vector along $\varepsilon_\boldsymbol{a} = \boldsymbol{\varepsilon}\cdot \boldsymbol{a}$. And so on.