Answering your question
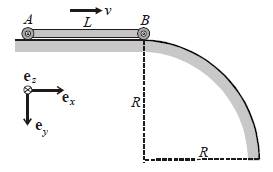
We can actually choose any "center of rotation" to calculate about. Suppose we describe this system by fixating on the point $A$ with $a$ being its position along the straight part of the track, specifically $a=0$ being the point in question where the wheel centered at $B$ enters the circular arc of the track. We'll say that from the perspective of $A$, point $B$ is an angle $\theta$ beneath the horizontal.
Using this as the center, the moment of inertia of the rod about its end is $I =m L^2/3$ and it experiences (assuming that normal force $N_B$ is indeed zero!) a constant torque $\tau = m~g~L/2$ (with the force $N_A$ not contributing because it goes through the center of rotation.)
Let me use dots for time-derivatives. At this particular moment the angular velocity $\omega = \dot\theta$ is zero because the beam has not yet started rotating, but the angular acceleration $\alpha = \ddot\theta$ is not zero because the pipe is beginning to change its angular velocity. More specifically, $\alpha = \tau/I = \frac32 g / L.$
So that's the simple answer. Yes, a velocity can be zero when an acceleration is not. It just can't stay zero, but it can certainly be zero. Something similar happens when you are rolling forward and throw your car into reverse; as you add gas, you reach a point where you are stationary relative to the ground (as you must: you go from travelling forwards to travelling backwards!) with a constant acceleration. Or, it's like throwing a ball into the air: eventually it comes to its maximum height, and at that particular instant its velocity is zero, transitioning from positive to negative: but afterwards it accelerates into a negative velocity.
How to finish this problem without a fixation on acceleration
For this sort of "constant acceleration" (albeit angular acceleration) we always see a dependence on the acceleration times $t^2/2$ to get the derivatives right; so in fact $\theta = \frac34~g~t^2/L.$ This is important because we can see that point $B$ is located at coordinates $[x, y] = [a + L~\cos\theta, -L~\sin\theta].$ The normal force is a constraint force: it stops a point from entering a region. To make the normal force zero we need a trajectory which is not passing inside the disk $$[L+r\sin\phi, -R + r \cos\phi]\text{ for }0\le \phi \lt 2\pi\text{ and }0\le r \lt R.$$
What we really care about here is $\phi \approx 0$ where the small angle $\phi$ can leads to the approximate formulas, $\phi$ in radians, of $\cos\phi \approx 1 - \phi^2/2,\;\sin\phi \approx \phi.$ Similarly I have carefully chosen that $\theta \approx 0$ at this place too, so that $\theta$ is also approximated that way.
Now if point $B$ is just barely not violating the constraints then it must mirror this boundary path for small angles and times, so that our approximate boundary $[L + R~\phi, -R~\phi^2/2]$ must approximately equal our approximate position of $B,$ which is $[a + L~(1 - \theta^2/2), -L\theta].$ Writing $a = vt$ and $\theta = \alpha t^2/2$ gives two equations:$$\begin{align}R \phi =&\; v t &+ O(t^4)\\
R\phi^2/2 =&\; L~\alpha~t^2/2 &+ O(t^6).
\end{align}$$We see that all of the higher-order stuff is easily neglected with just $\phi = vt/R$ in the first expression, but this can be substituted into the second expression to give $$v^2 / R = L \alpha.$$
This confirms just what your existing equation shows.