Contradiction in a simple torque/rotation problem - two ways of calculating external force don't agree

Question

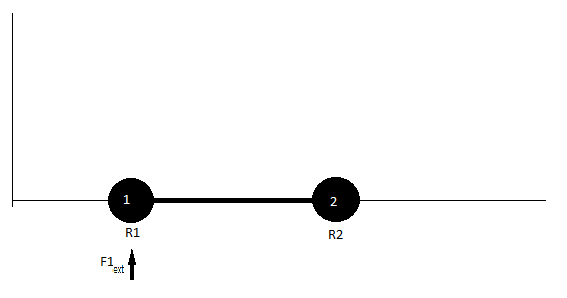
Suppose we have a rigid system of two point objects (both of unit mass) connected with a massless rod, with the objects horizontal on the x axis, at a distance $r_1$ and $r_2$ from the origin, respectively. A vertical force $F1$ is applied to the first object, and causes an angular acceleration $\alpha$ of the system around the origin.

The total force on the first object is $ma = 1 * (r_1 \alpha) = r_1 \alpha$, and similarly the total force on the second object is $r_2 \alpha$. So the total force on the system is :

$r_1 \alpha + r_2 \alpha = F1_{total} + F2_{total} = (F1_{int}+F1_{ext}+F2_{int}+F2_{int}) = F1_{ext}$, since $F2_{ext}=0$ and the internal forces cancel out. So

$F1_{ext} = r_1 \alpha + r_2 \alpha. \tag{1}$

On the other hand, we know that $T_{ext} = I \alpha$. The moment of inertia $I$ here is $m r_1^2 + m r_2^2 = r_1^2 + r_2^2$, and so $F1_{ext} \ r_1 = T_{ext} = (r_1^2 + r_2^2) \ \alpha$, from which follows that

$F1_{ext} = \alpha(r_1^2 + r_2^2)/ r_1 \tag{2}$.

But this is incompatible with $(1)$ above, since $r_1 \alpha + r_2 \alpha = \alpha(r_1^2 + r_2^2)/ r_1$ only when $r_1 = r_2$.

What is wrong with the above?

Answer 1

The 2nd solution you wrote down appears to be the correct solution. Offhand, I see two problems in the first solution. First, I think that a problem with the first attempted solution is that you made a subtle mistake in assuming that F=ma means that $F=mr_1α$. That seems like a plausible step at first but if you examine this step more closely you'll realize that it can't be correct to say that the acceleration of an object in this case is simply equal to the distance from the point of rotation times the angular acceleration. Suppose that the angular acceleration is zero (e.g., the masses are spinning around the origin at a constant angular velocity). According to your reasoning that would mean that the acceleration of the object is also zero. But you do know that an object spinning around a origin at a constant angular velocity doesn't have a zero acceleration, right? Rather, it has an acceleration vector which is of constant magnitude and always pointing toward the origin.

Another problem I see is that these are not freely moving masses being acted upon by only a force $F1_{ext}$. There are also the "hidden" forces of constraint which are forcing the masses to revolve around the point of origin.

Approaching this problem in terms of torques, moments of inertia, and angular acceleration looks like the most straightforward way of approaching this problem, and I believe that your 2nd solution is correct.