Is there any way to measure the one-way speed of light?

Question

Is there any way (practical or theoretical) to measure the one-way speed of light?

The two methods that come to mind are:

1. Stellar aberration, and

2. Using adiabatic clocks: synchronize clocks, then slowly move them apart

I think 2 is not really measuring one-way speed, although I can't work out exactly why.

Is 1 measuring one-way speed? In particular, if the one-way speed was not isotropic, would there be a different amount of stellar aberration if the telescope is pointed at stars that lie in exactly opposite directions?

Answer 1

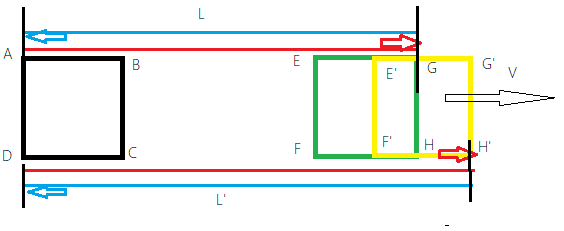
The „one way“ speed of light from a source to a detector cannot be measured independently of a convention as to how synchronize the clocks at the source and the detector. To synchronize these clocks one needs to know the one way speed of light, since it is the “greatest available speed” and no instantaneous transfer of signal is possible. So, there is a circular reasoning.

What can however be experimentally measured is the round-trip speed (or "two-way" speed of light) from the source to the detector and back again. Measured round trip speed of light is always equal to constant c.

A. Einstein‘s synchronization is a clock synchronization convention that assumes, that velocity of light in all direction is c or isotropic. It synchronizes distant clocks in such a way that the one-way speed of light becomes equal to the two-way speed of light.

H. Reichenbach's (or Reichenbach - Grünbaum) synchrony convention is self - consistent and admits that speed of light is different in different directions, while measured „round-trip“ speed of light is equal to c. For example, speed of light in one direction can be infinitely large and in the other infinitely close to c/2.

As soon as definition of simultaneity depends on the clock's synchronization scheme and is conventional, any one - way velocity of everything also depends on the same scheme and is conventional.

Therefore, considering aberration - in an inertial reference frame, in addition to the tilt angle of the telescope, one needs to know the speed of the laboratory. But clocks synchronization scheme would affect one – way speed of this laboratory. Hence, as soon as one determines the speed of the laboratory using Einstein-synchronized clocks, the tilt angle of the telescope will indicate that the speed of light is equal exactly to constant c.

Method Nr. 2 is known as slow clock transport and is equivalent to Einstein synchrony convention. Measured by means of this synchronization method one way speed of light will be equal exactly to constant c.

The first experimental determination of the speed of light was made by Ole Christensen Rømer. It may seem that this experiment measures the time for light to traverse part of the Earth's orbit and thus determines its one-way speed. However, the Australian physicist Leo Karlov showed that Rømer actually measured the speed of light by implicitly making the assumption of the equality of the speeds of light back and forth.

It is also not possible to "instantly" synchronize clocks by means of rigid rod, since absolutely rigid bodies do not exist and signal cannot move inside the rod faster than light.

Many experiments that attempt to directly probe the one-way speed of light have been proposed, but none have succeeded in doing so.

For example, from the center of a room, using identical catapults, one throws two identical clocks at the same distance. But, in a moving frame, these clocks will slow down to varying degrees. Even if one way speed of light is anisotropic, due to this discrepancy, the speed of light measured using these clocks will be exactly equal to constant c.

S. Marinov once proposed synchronization of clocks by means of a chain (or a conveyor belt). (See: S. J. Pokhovnik, „The empty ghosts of Michelson and Morley: A critique of the Marinov coupled-mirrors experiment“). But one has to keep in mind, that in a moving laboratory opposite sides of the chain would Lorentz – contract at different magnitude. It would lead to “desynchronization” of clocks and measured in this way the speed of light will be exactly equal to constant c.

R. W. Wood has considered a modification of Fizeau’s method for determining the speed of light, in which two toothed wheels are mounted at the ends of a long axle, and light is sent in one direction only (S. Marinov, M.D. Farid Ahmet employed this method). However, a stress – free relativistic twist of the axle would be an additional compensating factor. Measured by this apparatus one way speed of light will also be equal precisely to c (Herbert E. Ives, “ Theory of Double Fizeau Toothed Wheel”).

Max Jammer's „Concepts of Simultaneity“ presents a comprehensive, accessible account of the historical development of the concept as well as critique of many proposed experiments to measure the one way speed of light.

In rotating frames, even in Special Relativity, the non-transitivity of Einstein synchronisation diminishes its usefulness. If clock 1 and clock 2 (are equidistant from the center of the ring) on a rim of rotating ring are not synchronised directly, but by using a chain of intermediate clocks, the synchronisation depends on the path chosen. Synchronisation around the circumference of a rotating disk gives a non vanishing time difference that depends on the direction used. If one synchronizes clocks 1 and 2 by means of a flash of light from the center of the ring, measured by means of these clocks one – way speeds of light will be different clockwise and counterclockwise, but still satisfying Reichenbach‘s synchrony condition.

Lorentz‘s theory assumes that the speed of light is isotropic only in the preferred frame (Ether). The introduction of length contraction and time dilation for all phenomena in a "preferred" frame of reference, which plays the role of Lorentz's immobile aether, leads to the complete Lorentz transformation. Because the same mathematical formalism occurs in both, it is not possible to distinguish between LET and SR by experiment (see: Simulation of kinematical effects of the Special Relativity by means of classical mechanics in water environment)

Even though Reichenbach’s synchronization is more "universal", undoubtedly, from the practical point of view the Einstein – synchronization is the most convenient in inertial frames. The Lorentz transformation is defined such that the one-way speed of light will be measured to be independent of the inertial frame chosen.

Answer 2

How about using something which has well-defined speed but not at the speed of light? As an extreme example, suppose you have a conveyor belt, marked at regular intervals, and very well-calibrated speed. Then you know how long it takes for one tick-mark to go from the starting point to where the observer is. Synchronize the output pulse of light to a tick-mark, and record the time of arrival of both the light pulse and the tickmark.

I recognize that you'll need an extremely well-calibrated belt drive (and maybe a 20km belt :-) ), but perhaps one can extend the concept to, say, speed of sound through homogeneous rock.

Answer 3

One way speed of light measurement could be reduced to synchronizing distant clocks. Let's use Einstein's boxcar experiment with slight modification: Initially let's have a stationary boxcar and mark exactly points A, A', B, B'where A and B are on the embankment and A'and B' attached to the boxcar. at point A let's install a laser pointing directly to a mirror at A'(attached to the boxcar) which is reflecting the laser beam back to a photo sensor at A.Let's make the same arrangement at B and B'.If we move boxcar with non-relativistic speed (uniform )v from A to B (or from B to A) the mirrors at A'and B' will reflect the light at exactly the same moment, so we can synchronize the clocks at A and B. Accuracy of such synchronization would depend how precise A, A'and B and B' are marked, how big is the distance between A and B and how fast the boxcar is moving.

Answer 4

The way to do this (there might be other ways, but this is the one that does not involve scattering) is if we can use spacetime curvature itself to lead the photon back to our clock. Photons do follow geodesics, actually it is called a null geodesic.

Black holes have something very interesting around them, called the photon sphere. It is possible for a photon inside the photon sphere to come around the black hole.

A photon sphere1 or photon circle[2] is an area or region of space where gravity is so strong that photons are forced to travel in orbits. (It is sometimes called the last photon orbit.)[3] The radius of the photon sphere, which is also the lower bound for any stable orbit, is, for a Schwarzschild black hole: $${\displaystyle r={\frac {3GM}{c^{2}}}={\frac {3r_{\rm {s}}}{2}}}{\displaystyle r={\frac {3GM}{c^{2}}}={\frac {3r_{\rm {s}}}{2}}}$$ where G is the gravitational constant, M is the black hole mass, and c is the speed of light in vacuum and rs is the Schwarzschild radius (the radius of the event horizon) - see below for a derivation of this result.

What makes this very complicated is that many forget, that the current definition of the speed of light uses a local measurement. So we need to do a local measurement.

Now if we have a clock that hovers at the photon sphere, and shoot a photon around the black hole, and wait for the photon to come around, check the time passed on the clock, and know the circumference, them we can in theory check the real one way speed of light.

Answer 5

There is a way to check whether the light travels the same speed in one direction between the 2 mirrors of a resonant cavity. If the light travels at the same speed in both directions, the strength of the oscillating field will follow a simple sine wave pattern along the resonator.

If, on the other hand, the speeds are different, then we have different wave lengths on the different directions. We'd see the wave pattern fall and rise in amplitude along the resonator.

Answer 6

So taking a normal emitter, reflector, detector setup, can we do the following? Make the first measurement, and log the time. Put a medium that allows light through but "slows" it down slightly, put in front of the emitter side, take another measurement. Then take that same medium and put it in front of the detector side, take the last measurement, and compare.

Test "a" is the control with no medium. If light moves the same velocity in every direction, test b and c should give a different result from the control, if say there is a change when the medium is in front of the emission side, but no change at all in front of the detector side, then this means in one direction light moves at $\frac{1}{2}c$, and the other instantaneously, and vice versa.

Answer 7

One theoretical way of measuring the one-way speed of light one-way without the need for synchronizing clocks is to use the photon sphere of a black hole at $1.5R_S$. At this distance photons sent tangentially will travel around the hole and reach the emitting observer from the other direction. A light pulse can be sent and the time delay till arrival will tell the speed.

Note that in this case the local curvature of spacetime can be made arbitrarily small by using a more massive black hole (the $1.5R_S$ distance to the hole also increases). The curving of the light ray is a non-local phenomenon set up by the overall spacetime manifold, and as one approach the infinite mass limit one approaches the straight light beam limit. So were anything weird going on with one-way light measurements it would show up in the Schwarzschild solution.

Answer 8

It requires two different light measuring machines. One is the standard “how far there and back,” where a laser shoots (point a) shoots at a mirror (point b) which reflects it back to a mechanism on (point a) which stops a clock that’s activated when the laser is fired. The next step is to have a similar mechanism, but with a third point, thus that Laser (point x) shoots to a mirror (point y) which reflects to another mirror (point z) which reflects it finally back to (point x) which has the same clock mechanism. The distance between points x and y, y and z, and x and z should be the same distance as point a to point b. The mirrors should reflect at a 60 degree angle on the second contraption.

Activate the first one, and get the the two way measurement of the speed of light (distance from point a to b and back). Well call the time it takes “t” With this info, using the second machine gives a three way measurement with a different time. We’ll call this time (u).

Now the experiment can go two different ways depending on if the theory that light travels at different speeds depending on direction is true or not. If (t/u) =/= (2/3), then light travels at different speeds and it becomes harder to measure.

Assuming the former is false, light travels at the same speed. Simply do the equation (u-t) for your answer.

If you take the same triangle set of mirrors and place 6 of them side by side so that they form a hexagon, you can find how long it takes light to travel in each direction by comparing the touching sides of these “triangles.” How long light takes to travel through one of these triangles is the total perimeter. Since each triangle is sharing a side with another triangle at the same spot, that means that the sides of two touching triangles have to be equal, or their lasers are facing opposite directions. It requires a lot of filling in the blanks, but if you keep placing triangles next to eachother and recording what possible lengths they could be, you eventually get a pattern and can find which lengths are which. Now, you can simply just find the side length of any triangle and that is the one way speed of light, but only in the given direction it’s traveling in. You have to measure multiple sides to find the one way speed of light for different directions.

Answer 9

I think we can measure one way speed of light. we need to redefine simultaneity. My proposition is as follows: if a rigid body AB of length l is moving without any acceleration parallel to X axis and at time t0 its point A is at location x, then simultaneously its point B is at location x+l

Let's now design the experiment to synchronize distant clocks and measure one way speed of light:

Imagine four spaceships flying as perfect square EFGH towards (or away from) not moving (at least relative to each other) points ABCD, where AD is parallel to EF and distance EF equals AD. Points EG should be collinear with points AB and points FH collinear with CD. Making sure that ABCD (and EFGH) is a square is relatively easy, since 2-way speed of light is constant: we can measure (and correct, if necessary) distances BD and CA by sending light signals from B to D (and from C to A) and back Now at certain time (clocks at A and D can be pre-synchronized using Einstein convention, but it is not absolutely necessary) we can measure distance from A to G (L) and from D to H (L’) using light (laser) signal send from A to G (and reflected back to A) as well as distance from D to H. If the distance AG (L) equals DH (L’) signals from A and D had been sent simultaneously; if not, it would be easy to adjust the clocks so they are synchronized.

Please let me know if I made any wrong assumption Of course, theoretically it would be sufficient to have only the lines AD parallel to GH, but practically it could be difficult to make sure they are parallel to each other