0
$\begingroup$

The instantaneous speed of a point along a circular path is given by $v=\omega r$, where $$\omega = \frac{\Delta \theta}{\Delta t},$$ $s=\Delta \theta r$, and $v=s/t$.

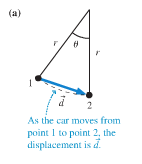
However, isn’t the displacement measured as in the following figure?

enter image description here

The relationship between normal speed $v$ and angular speed $\omega$ is related via arc length $s$. The displacement $d$ above is a straight line, not the arc length. So why is $v=s/t$ when it should be $v=d/t$? Would this not invalidate the equation $v=\omega r$?

Improve this question
$\endgroup$
3
  • $\begingroup$ I would suggest you revisit the definitions of average and instantaneous velocity. $\endgroup$
    – Declan
    Commented Aug 30, 2015 at 3:42
  • 1
    $\begingroup$ the distance traveled is $s$ and not $d$. $\endgroup$
    – John Alexiou
    Commented Aug 30, 2015 at 5:06
  • $\begingroup$ instantaneous velocity is taken in a time interval so small that displacement and distance can approximately be taken equal to each other. Hope this clears the confusion. $\endgroup$
    – Saad
    Commented Aug 30, 2015 at 7:08

1 Answer 1

Reset to default
0
$\begingroup$

The displacement d above is a straight line, not the arc length.

The point particle travels along the arc length, not along the straight line.

So why is v=s/t when it should be v=d/t?

If should not be d/t. It should be s/t. The value s is the distance travelled and t is the time.

Would this not invalidate the equation v=ωr?

It would if it were correct, but you are wrong to use d/t. So the equation is valid.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.