Why is there an energy gap in superconductors?

Question

I'm a little out of my depth here...

I'm trying to understand quasiparticle tunnelling in superconductor-insulator-superconductor junctions. Many books use the "semiconductor model" to explain this:

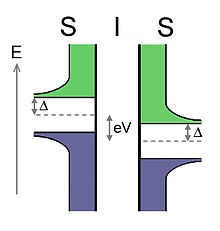
_{(source: wikimedia.org)}

These diagrams show the available quasiparticle states (with a large band gap due to the formation of Cooper pairs), the filled states, and the empty states.

My question with these diagrams is: shouldn't all the electrons exist as Cooper pairs? I assume that the lower band is filled with quasiparticles, since Cooper pairs would all be at the same energy level and quasiparticles obey Fermi-Dirac statistics, but I don't know where they're coming from.

Also, why is there an energy gap in the quasiparticle energy states? I understand that this gap corresponds to the energy needed to break Cooper pairs, but I don't understand why would you need to break Cooper pairs to raise the energy of quasiparticles.

Or is this "semiconductor model" not fully representative of the physics?

Answer 1

The lower part is not filled with quasi-particles. At zero Kelvin, in zero magnetic field and with zero disorder all free electrons condense and form the superconducting condensate. The semiconductor model now describes the breaking of Cooper pairs not as resulting in two electron-, but in one electron- and hole-like excitation. As you are potentially familiar with in semiconductors, one electron of energy $E$ above the Fermi level may be described by one hole with the same energy below.

Now, this is the equilibrium. Tunnelling processes however are very non-equilibrium processes! When you apply a bias across your junction you give rise to quasi-particle excitations, with result in a tunnelling current. That is why, even at 0 Kelvin, there is "electron" tunnelling...

Answer 2

My answer will be brief unfortunately. Here's how I think about it. Electrons in the band that is near the Fermi energy interact through some attractive force. The ground state of this system is the superconducting state, with all those electrons paired up and you have a superconducting condensate which is that "Cooper sea" Now we consider low-energy excitations of this state. Turns out this just comes about by breaking one of this Cooper pairs (by thermal excitation or otherwise). This costs an energy equal to the superconducting gap. So those quasiparticles, which are just the elementary excitations of the ground state (creation operators acting on the vacuum state), can be identified with electrons from broken Cooper pairs. Since the quasiparticles in this case are just electrons, they obey Fermi statistics. And they will tunnel across your barrier there to minimize the free energy of the system.