0
$\begingroup$

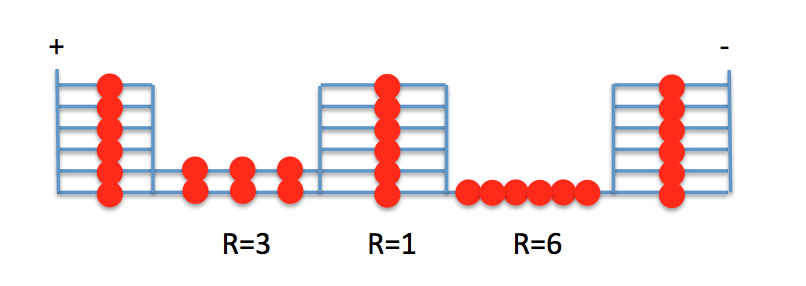
Potential difference = Current x Resistance where Resistance is a constant. Walking through a circuit I have 1 battery, a wire and 2 components. I start of with 6 volts at the battery and after the current goes through the 2 components I'm left with a 0 volts.

My problem is understand this statement: when an electrical charge goes through a change in potential difference then energy is transferred. Now, I'm trying to put this statement into the circuit described above.

I start of with a resistance of 1 ohm by the wire and 6 amperes which result in 6 volts. When I meet the resistor however the resistance increases to let's say 3. Does the current decrease at the same rate the resistance increases? So if the resistance goes down to 3 will the current be 2 so that in the end I have a potential difference of 6? If that's the case then why am I loosing 3 volts after the first component since there is no change in potential difference.

I guess my question can be shortened to whether or not the current decreases at the same rate resistance increases and vice-versa?

I'm pretty sure my question is not so well but here is the circuit described:

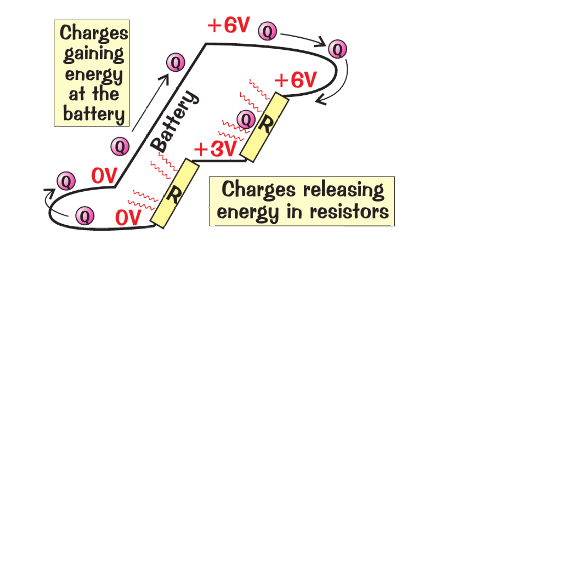
enter image description here

Is there any way you can walk me through what happens here? How does this relate to ohm's law?

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ What do you mean "1 ohm by the wire"? I don't see this 1-ohm resistor in your diagram. $\endgroup$
    – David Z
    Commented Mar 29, 2015 at 16:06
  • $\begingroup$ It was an example. I was trying to give units to the diagram in the hope to understand it myself. $\endgroup$
    – Bula
    Commented Mar 29, 2015 at 16:12

2 Answers 2

Reset to default
1
$\begingroup$

Trying to address this misconception:

I start of with a resistance of 1 ohm by the wire and 6 amperes which result in 6 volts. When I meet the resistor however the resistance increases to let's say 3. Does the current decrease at the same rate the resistance increases? So if the resistance goes down to 3 will the current be 2 so that in the end I have a potential difference of 6? If that's the case then why am I loosing 3 volts after the first component since there is no change in potential difference.

In a circuit as you drew, the current DOES NOT CHANGE around the loop. All the electrons that start at one end have to arrive at the other end. Instead, think of it as a road that starts out with multiple lanes, and then narrows. All the cars try to get across the narrow stretch, but they end up closer together (more collisions = more heat dissipation). I tried to illustrate with a diagram:

enter image description here

Fewer "lanes" = more electrons per lane = higher resistance = greater voltage drop and power dissipation. The total voltage drop is given by the battery - how it ends up distributed across the different components depends on their relative resistance. If the circuit comprises a number of series resistors $R_i$ then the voltage drop across any one of them is

$$V_j = V \frac{R_j}{\sum{R_i}}$$

in other words, it scales with the resistance of each component, precisely because the current is the same in each component.

Improve this answer
$\endgroup$
14
  • $\begingroup$ The diagram is brilliant $\endgroup$
    – Bula
    Commented Mar 29, 2015 at 18:03
  • $\begingroup$ The number of electrons that end up on a lane is amperes/resistance in this model right? $\endgroup$
    – Bula
    Commented Mar 29, 2015 at 18:09
  • $\begingroup$ What did you use to make the diagram? $\endgroup$
    – danimal
    Commented Mar 29, 2015 at 18:43
  • $\begingroup$ @Floris I have a question. You say that the current does not change. Do you mean that the number of electrons stays the same? I'm asking because I have been doing some reading and I have found the following statement: as resistance increases the current decreases $\endgroup$
    – Bula
    Commented Mar 29, 2015 at 19:26
  • $\begingroup$ @danimal - I used Powerpoint (Mac 2011), followed by a screen shot to turn it into a picture I can upload. $\endgroup$
    – Floris
    Commented Mar 29, 2015 at 19:31
1
$\begingroup$

There's an energy transfer whenever there is a change in potential, not potential difference. The (electric) potential, measured in volts, is the electric potential energy (EPE) of a unit charge at a particular point in the circuit.

So, imagine a particle of unit charge travelling in the direction of current. It starts off with a higher potential (therefore higher EPE), and travels through the circuit. As the unit charge travels through resistors, there is an energy transfer from EPE to heat. So, the unit charge loses EPE as it goes through the resistor, and so it loses potential. Eventually, the unit charge returns to the battery, arriving with a lower potential. The battery then restore the potential of the unit charge to its normal level.

If you want an analogy, a gravitation potential energy one can be adopted: Imagine you have a certain GPE, and you are on a sledge sliding on a smooth, horizontal pathway. In this analogy, you are the charge carrier, and the smooth, horizontal pathway is an ideal wire of zero/negligible resistance. Then your sledge slides down a slope. The slope is analogous to the resistance, where instead of converting EPE to heat, you are converting GPE to KE. (The value of KE the sledge has in this analogy doesn't matter). At the end, you and your sledge is quite low down. So an elevator takes you back up to the starting height again (the battery).

If you are wondering where EPE arises, then, from my basic understanding, the battery sets up an electric field along the wire to accelerate charge carriers along it, and it is this field that provides the EPE (like the gravitation field on Earth provides GPE), so to speak.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.