Do Sidebands mean the frequency of an AM radio wave is not constant?

Question

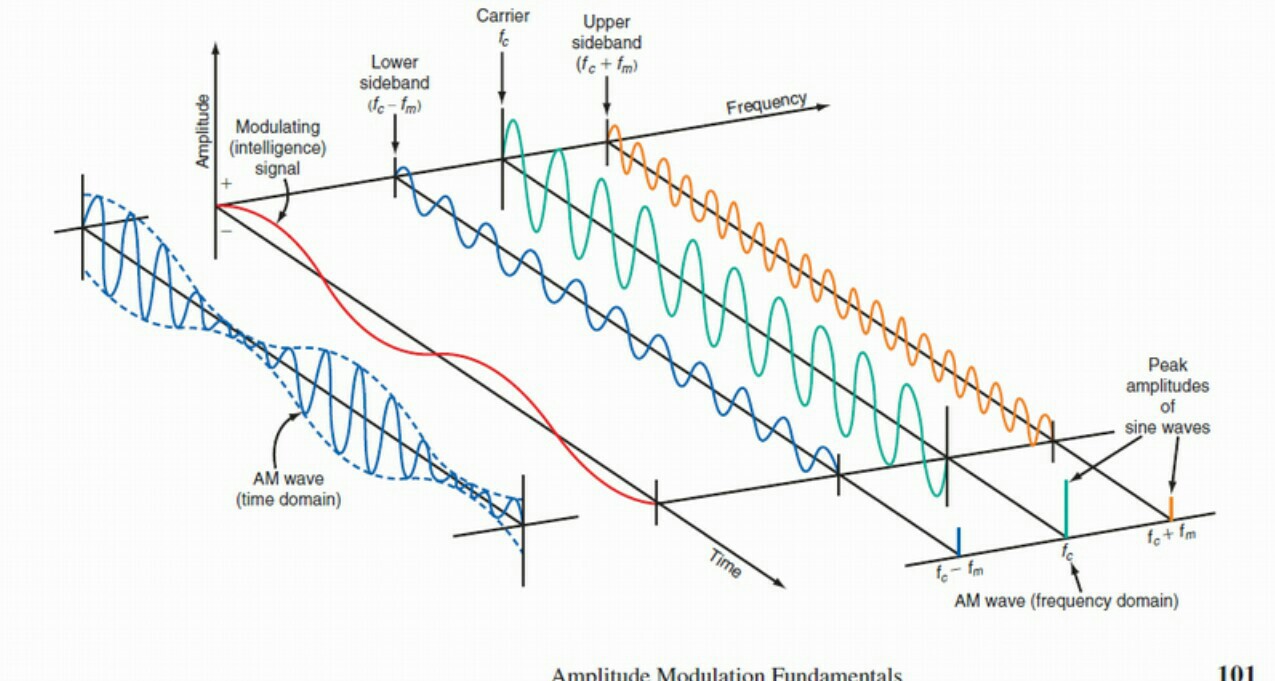
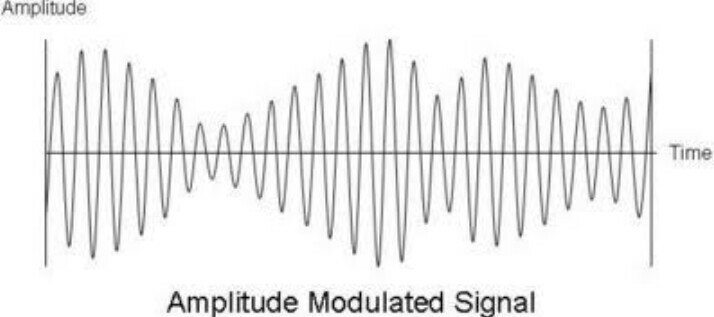
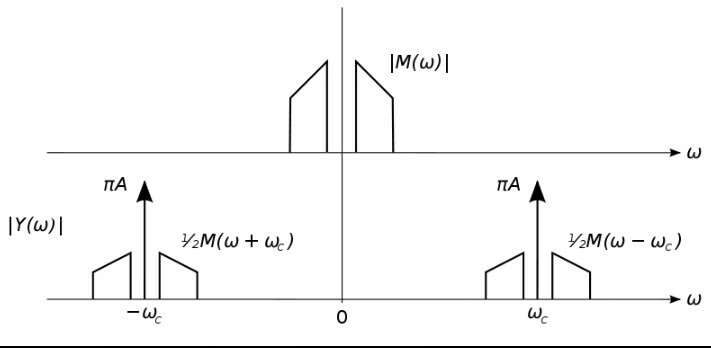
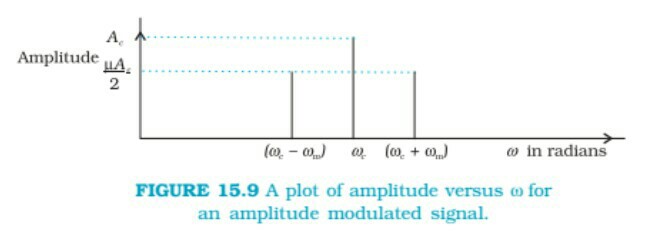
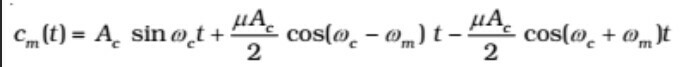I'm studying for A-level now. I have read some other posts explaining how the sidebands generated after the carrier wave is modulated. So, if there is sideband frequency, the frequency isn't constant, right? However, they have written that there is constant frequency for amplitude modulated wave on the book, so which one is right?

Also, there is a question (text below) asking for the frequency of carrier wave according to the graph showing the amplitude modulated radio wave carrying a signal, and the question can be solved by counting the number of modulated wave in certain time. Does it mean that the frequency of AM wave is constant and same as the frequency of carrier wave or we can find out the frequency because the sidebands cancel each other out?

If a radio station carries music, the wave transmitted by the radio station will differ from the wave shown in Figure 33.3. There is only one signal frequency present in the signal in Figure 33.3. Music consists of many, changing frequencies superimposed so that it has a more complex wave pattern. The amplitude of the carrier wave will change as the music pattern changes. The carrier wave frequency does not change but the amplitude of the trace will change with time.

In amplitude modulation (AM), the frequency of the modulated wave is constant. The amplitude of the modulated wave is proportional to, and in phase with, the signal.

Answer 1

In amplitude modulation, the frequency of the carrier wave is constant. The frequency spectrum of an AM signal includes sidebands, but those aren't the carrier wave. In your second figure, the carrier wave is the black line. You'll note that the amplitude changes; it increases and decreases in accordance with the modulation, however the frequency of this wave does not change. That is the essence of amplitude modulation. For a carrier wave of constant frequency, the information is encoded in the amplitude of the signal. The presence of sidebands does not imply the frequency is non-constant, merely that the overall signal is not a single pure frequency. The carrier still remains the same throughout and the frequency of the AM wave is connotatively the same as the frequency of the carrier.

Answer 2

A full answer needs Fourier analysis maths and an appreciation of the resulting bandwidth theorem that spread in frequency (bandwidth) multiplied by time duration is greater than approximately 1 (or 2 pi) or thereabouts. Any modulation of a pure sinusoid will give a spread of frequencies around your central carrier frequency. Both AM and FM produce a spectral bandwidth. When you have a very simple sinusoid amplitude modulation the frequency spectrum (Fourier analysis) comes out as 2 sharp peaks split either side of the carrier by an amount equal to the modulation frequency. Of course the sum of two nearby frequency sinusoids is already studied in high school as beats. The beat frequency being the difference ( or half of).

There is quite a bit of tricky and confusing ideas in spectral analysis. In full Fourier theory an infinite time window is analysed and so there is no concept of variable frequencies at all, "exact" frequencies do not alter. This is akin to a pure sinusoid must go on forever and if you truncate a sinusoid you unavoidably introduce a lot of (a spread of) other frequencies. To get closer to a time varying frequency spectrum we need to take a window in time and the duration T of this window is somewhat arbitrary! Anyway the resulting frequency spectrum is essentially smooth over frequency range of 1/T which can be regarded as the frequency resolution, determined by the bandwidth theorem. We can then move this window along our signal to see how frequency spectrum changes.

Basically I think "frequency" can be a bit ambiguous of a concept. If you have a pure sin wave and you give it some FM yeah you introduce side bands but also with AM you get frequency side bands too, which may seem odd, but the frequency with which you do the modulating is going to come out as a spread in the frequency spectrum whether it's FM or AM.

Answer 3

I have read some other posts explaining how the sidebands generated after the carrier wave is modulated. So, if there is sideband frequency, the frequency isn't constant, right? However, they have written that there is constant frequency for Amplitude modulated wave on the book, so which one is right? 

Amplitude modulation has a constant frequency. This is correct.But, there is a twist.

AM wave actually has got 3 waves, Carrier wave and the side bands. Presence of side bands does not change frequency of AM wave. It simply means 3 different waves can be transmitted.

Carrier has its own fixed frequency. As can be seen from above equation. *Amplitude modulated wave has the same frequency as careier. *

When the three waves are added together, what we get is amplitude modulated wave which has a constant frequency .

Here, is the twist The side bands actually do have variable frequency, thats how the message is carried. Bcoz, message itself has a variable frequency. Var freq is the information we wish to transmit. Look at width of side bands above.

But, remember when the three are added together a constant frequency wave is produced.

Extra:

Any of the two can be used to to retrieve message by transmitting

(a) both the side bands

(b) only one side band

Usually due to economic reasons only the upper or lower side bands of an AM is often transmitted.

Why not to use carrier frequency?

The frequency of a radio or television station is actually the carrier wave's frequency. However, because the information transmitted by a radio signal is not at the carrier frequency itself but contained in sidebands on either side of the carrier, the energy of the carrier component is not useful in transmitting the information. Therefore, in many modern modulation methods the carrier is not transmittedWiki

As, $A_c$ and $\omega_c$ are constant, you cannot use them information transmission. Rather, only side bands are used.

The carrier is reintroduced at the receiver by a beat frequency oscillator(BFO). And, the three waves are regenerated.

Answer 4

The text and the technical article below may add some insight into the spectral components of a "carrier wave" with amplitude modulation. I am the author of same.

» The variation of r-f output power from the transmitter is the result of the vector addition of the power contained in the upper and lower sideband spectra with the power contained in the carrier.

» The carrier wave itself has nearly constant amplitude during all modulation percentages from nearly -100% to +100%.

» Modulation by a perfect sinewave to almost exactly ±100% increases the total r-f output power to almost exactly 1.5X the power of the unmodulated carrier (total r-f current output increases almost exactly by √1.5 = 1.225, or 22.5%).