I'm sure today they can use GPS and radar, but I was pondering the queation when I saw a film clip of a vintage analog dial labeled in mach number. I'm supposing that the usual way of measuring the pressure drop of the air flow would not work in this case. So what does?
$\begingroup$
$\endgroup$
4
-
$\begingroup$ Possible duplicate. aviation.stackexchange.com/questions/1793/… $\endgroup$– BowlOfRedCommented Jan 20, 2015 at 10:05
-
2$\begingroup$ I think this may be off-topic because it is a dupe of aviation.stackexchange.com/questions/1793/… (I don't know how to create a migration request) $\endgroup$– Carl WitthoftCommented Jan 20, 2015 at 12:58
-
1$\begingroup$ Note that cross-site duplicates are not valid close reasons (see also this one). That said, I don't really see any physics question here $\endgroup$– Kyle KanosCommented Jan 20, 2015 at 13:33
-
1$\begingroup$ Cross-site is okay, and this is an acceptable physics question too. It's a common problem in experimental measurements and how it works is intimately tied to the physics of the problem. If this were about correlating Mach number in a wind-tunnel with a pitot-probe for an experiment, there wouldn't be a problem with it. $\endgroup$– tpg2114Commented Jan 20, 2015 at 14:25
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
If we consider a pitot-static probe in supersonic flow we get something that looks like (source):
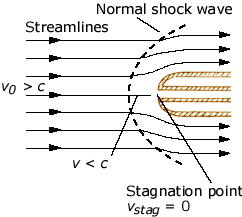
The probe measures the stagnation pressure in the part of the probe normal to the flow and the static pressure in the part of the probe perpendicular to the flow. There is a small bow-shock around the tip of the probe. But we can go ahead and assume that the shock is normal directly in front of the inlet to the probe. This allows us to use the normal shock relations after some manipulations to get:
$$\frac{P_{stag}}{P_{static}} = \frac{\gamma+1}{2}M^2\left(\frac{\left(\gamma+1\right)^2M^2}{4\gamma M^2-2\left(\gamma-1\right)}\right)^\left(1/(\gamma-1)\right) $$
where $\gamma = 1.4$ unless you are flying at hypersonic speeds. This equation is non-linear and requires a solver, but once the two measurements are known from the probe you can determine the Mach number.
$\begingroup$
$\endgroup$
2
The pitot tube measures stagnation (i.e. dynamic) air pressure. There is also a static port that measures the actual air pressure. This is enough information.
First of all, the pilot cares about "knots indicated air speed" (KIAS). That is not true speed over the ground. (A "knot" is one nautical mile per hour. A nautical mile is one arc-second of latitude on the earth, or about 1.15 statute miles.) Rather it is the speed the wings care about, for aerodynamic behavior. If a plane is flying at constant KIAS, its speed over the ground is higher at higher altitudes because the air is thinner.
The static air pressure determines what altitude the altimeter reads. (Actually, there is an adjustment for meteorological air pressure, so it can tell the actual height above mean sea level. The pilot needs to stay above towers, mountains, etc.)
The speed of sound decreases in the thinner air at higher altitude. The airspeed indicator takes that into account, so it knows the plane's current Mach number, which is what you asked.
GPS is of no value in these calculations. It can only give speed over the ground and, in modern versions, altitude. It knows nothing about wind and atmospheric pressure.
answered Jan 20, 2015 at 14:12
-
1$\begingroup$ So my reading is that KIAS is a measure of lift rather than speed, right? - the plane is flying at a speed relative to the air such that the lift is the same as that which would arise from a knot relative speed at a standard pressure (presumably that at sea level?) $\endgroup$ Commented Aug 8, 2015 at 11:45
-
1$\begingroup$ @Wet: Right, so when you are subsonic (takeoff, cruising, landing) the indicated air speeds you should use are always about the same regardless of density altitude (altitude corrected for temperature and humidity), so there are colored bands on the airspeed indicator. $\endgroup$ Commented Aug 8, 2015 at 12:22