How does the center of gravity influence equilibrium?

Question

This is really hard for me to think: How does a single point determine the object's equilibrium? If an object is displaced from its equilibrium position and if the equilibrium is stable, the object again comes back to the initial position. My book says it is due to center of gravity and couple constituted by the gravity and normal reaction force. But how does this coupling and center of gravity help in bringing back the equilibrium state? Can anyone help me visualize this?

Answer 1

But how does this coupling and center of gravity help in bringing back the equilibrium state?

First of all, you may be thinking along the lines of a general definition of the term "coupling", such as "the act of bringing or coming together; Pairing" (Merriam Webster). But in statics the term "couple", sometimes referred to as a "force couple", specifically means a pair of two equal and opposite parallel, non collinear forces that causes rotation without translation.

For an object to be in equilibrium, both the sum of the forces and the sum of the moments (torques) about any point must be zero. Since the forces for a couple are equal and opposite, the net force is zero. But since they are not on the same line of action, they create a torque or moment (turning action).

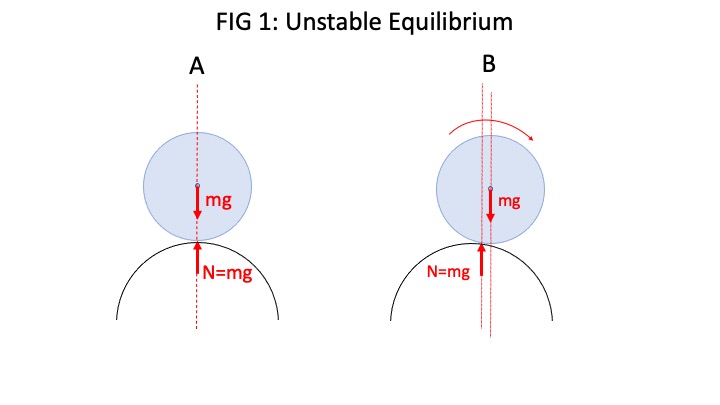
With the above in mind, consider the figures below.

FIG 1A shows a sphere on top of a hemispherical surface where the force acting down on the center of gravity of the sphere is aligned with the normal reaction force of the hemispherical surface acting up on the sphere. Since both the sum of the forces and sum of the moments (torques) is zero, the sphere is in equilibrium.

However, the equilibrium is unstable since the contact surface is a point and if the sphere becomes perturbed to the right as shown in FIG1B, the two forces are no longer aligned and form a clockwise couple. The couple produces rotation to the right which further separates the lines of action of the forces further increasing the magnitude of the couple and clockwise angular acceleration. Ultimately, the sphere rolls off the surface.

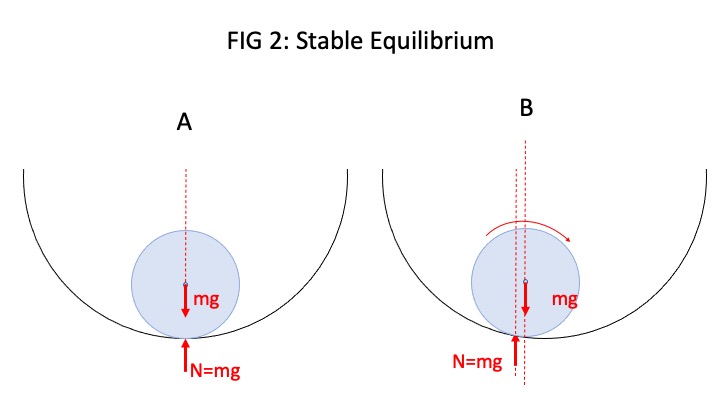
FIG2A, on the other hand, shows a sphere in the well having a hemispherical surface. This sphere is in stabile equilibrium. If it is perturbed, say to the left as shown in FIG2B, the clockwise couple that is produced by the two forces causes the sphere to roll back to its original position. (Note: technically it will roll back and forth until the potential energy it was given when perturbed is dissipated by rolling resistance that is always present to some extent).

Hope this helps.

Answer 2

How does a single point determine the object's equilibrium?

It does not. At least, if we are talking about any object. However, if we are taking about a rigid body, that is different.

Mechanics is about movement of points. The Newton's laws and everything else are very clear and sharp about what happens to points when they are moving, points subject to forces, points accelerating, etc. But a point is a mathematical idealization. In practice, there are no dimensionless objects, just approximately dimensionless ones. And of course, there are extensive bodies, for which the point abstractions are pointless.

For a point, the condition of equilibrium is achieved when all the forces applied to it cancels each other out. In other words, the net force over it are zero. It guarantees that if the point is at rest, it will remain so, until another force breaks the equilibrium condition somehow.

I don't know the context, but it seems that your book is talking about the equilibrium between gravity and the Normal force. Earth's Gravity attracts any massive point to the center of Earth. When we are in the surface of Earth, since it is solid and we can't cross the floor, we stay at its surface. What happens is that the floor stop us, or any massive body, to fall to the center of Earth. The way it stops us is by exerting a force, counter-balancing the force of gravity. We call it by Normal force.

It is clear that any massive object in Earth's surface should be in equilibrium, at least if it's not flying or falling. Out of equilibrium would mean that there are non zero net force, and by second law, some acceleration in some direction.

---

Now everything here said is true about points. But in mechanics, we also work with rigid bodies. A rigid body is a collection of points whose the relative positions are fixed somehow. So if one point moves, all the others also moves, with same velocity and direction. It means that if we lock one point position all the others are fixed? No.

Rigid bodies can rotate about any point. Rotations doesn't change the relative distances in the body. But if we lock the position of one point, and we lock the angle of any axis passing in this locked point with any other axis, the entire body position is locked.

To visualize this, consider two examples.

1. You put a coin over a table. The coin does not cross the table and fall in the ground, so there is a normal force acting on the coin. The coin didn't jumped away after you left it in the table, so the normal force is not "winning" against the gravitational force, so the net force is zero and the coin is at equilibrium. But wait: a coin is not a point! Well, but it is (approximately) rigid, so all the relative distances of points in the coin are fixed. Is it spinning? No? So actually you put it with one of the faces parallel with the table and table's surface are preventing the coin to rotate (you can rotate the coin with your hand if you want, but then you need to add one more force on it, and this force should win against friction between coin and table).

2. You hold a raw bread dough in the tip of your finger, over your head. It is well positioned, with its center of mass right above your finger, so you feel all the weight initially over your finger, and the normal force prevent it to fall immediately. However, unfortunately it falls in your head. You thought that all conditions of equilibrium was fulfilled, but forget about it not being a rigid body.

Answer 3

I am going to present an example which helped me understand this!

Assume two surfaces as shown below: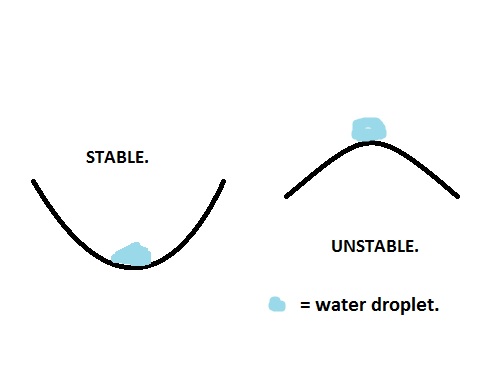

Assume a droplet of water to be placed in a stable state on both of them. (i.e. at the bottom of the left one, and the top of the right one.

In the left one, if you push the water droplet by a small amount, the water droplet would tend to flow back into the point where you pushed it away from. Therefore it is in a Stable Equilibrium state. (i.e. the object comes back to its initial position.) this happens because the gravitational force always tries to bring a body to a lower potential energy position.

On the contrary, in the surface represented on the right, the slightest push would cause the droplet to go further down the surface, under gravitational acceleration. i.e. It is in an unstable equilibrium state.

Here, the gravitational force causes the droplet to return/move further away from its equilibrium state, and that is how it returns to its initial position in the left hand representation.

I hope this has been clear enough.